1
GATE EE 2005
MCQ (Single Correct Answer)
+2
-0.6
A load centre is at an equidistant from the two thermal generating stations $${G_1}$$ and $${G_2}$$ as shown in figure. The fuel cost characteristics of the generating stations are given by
$${F_1} = a + b{P_1} + cP_1^2\,Rs/hour$$
$${F_2} = a + b{P_2} + 2cP_2^2\,Rs/hour$$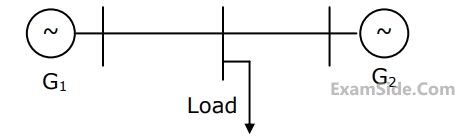
$${F_1} = a + b{P_1} + cP_1^2\,Rs/hour$$
$${F_2} = a + b{P_2} + 2cP_2^2\,Rs/hour$$

Where $${P_1}$$ and $${P_2}$$ are the generations in $$MW$$ of $${G_1}$$and $${G_2}$$, respectively. For most economic generation to meet $$300MW$$ of load $${P_1}$$ and $${P_2},$$ respectively, are
2
GATE EE 2003
MCQ (Single Correct Answer)
+2
-0.6
Incremental fuel costs (in some appropriate unit) for a power plant consisting of three generating units are
$${\rm I}{C_1} = 20 + 0.3\,\,{P_1},\,{\rm I}{C_2} = 30 + 0.4\,\,{P_2},\,{\rm I}{C_3} = 30$$
Assume that all the three units are operating all the time. Minimum and maximum loads on each unit are $$50$$ $$MW$$ and $$300$$ $$MW$$ respectively. If the plant is operating on economic load dispatch to supply the total power demand of $$700$$ $$MW$$, the power generated by each unit is
$${\rm I}{C_1} = 20 + 0.3\,\,{P_1},\,{\rm I}{C_2} = 30 + 0.4\,\,{P_2},\,{\rm I}{C_3} = 30$$
Assume that all the three units are operating all the time. Minimum and maximum loads on each unit are $$50$$ $$MW$$ and $$300$$ $$MW$$ respectively. If the plant is operating on economic load dispatch to supply the total power demand of $$700$$ $$MW$$, the power generated by each unit is
3
GATE EE 2000
MCQ (Single Correct Answer)
+2
-0.6
The incremental cost characteristic of two generators delivering $$200$$ $$MW$$ are as follows
$$\,\,\,{{d{F_1}} \over {d{P_1}}} = 20 + 0.1{P_1},\,\,{{d{F_2}} \over {d{P_2}}} = 16 + 0.2{P_2}$$
For economic operation, the generations $${P_1}$$ and $${P_2}$$ should be
For economic operation, the generations $${P_1}$$ and $${P_2}$$ should be
4
GATE EE 1999
MCQ (Single Correct Answer)
+2
-0.6
An industrial consumer has a daily load pattern of $$2000$$ $$kW$$, $$0.8$$ lag for $$12$$ Hrs. and $$1000$$ $$kW$$ $$UPF$$ for $$12$$ Hrs. The load factor is
Questions Asked from Power Generation Cost (Marks 2)
Number in Brackets after Paper Indicates No. of Questions
GATE EE Subjects
Electric Circuits
Electromagnetic Fields
Signals and Systems
Electrical Machines
Engineering Mathematics
General Aptitude
Power System Analysis
Electrical and Electronics Measurement
Analog Electronics
Control Systems
Power Electronics