A ram in the form of a rectangular body of size $l = 9 \text{ m}$ and $b = 2 \text{ m}$ is suspended by two parallel ropes of lengths $7 \text{ m}$. Assume the center-of-mass of the body is at its geometric center and $g = 9.81 \text{ m/s}^2$. For striking the object P with a horizontal velocity of $5 \text{ m/s}$, what is the angle $\theta$ with the vertical from which the ram should be released from rest?

A rigid massless tetrahedron is placed such that vertex O is at the origin and the other three vertices A, B, and C lie on the coordinate axes as shown in the figure. The body is acted on by three point loads, of which one is acting at A along x-axis and another at point B along y-axis. For the body to be in equilibrium, the third point load acting at point O must be

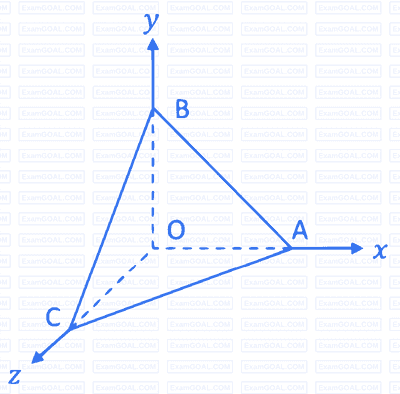
A massive uniform rigid circular disc is mounted on a frictionless bearing at the end E of a massive uniform rigid shaft AE which is suspended horizontally in a uniform gravitational field by two identical light inextensible strings AB and CD as shown, where G is the center of mass of the shaft-disc assembly and g is the acceleration due to gravity. The disc is then given a rapid spin w about its axis in the positive xaxis direction as shown, while the shaft remains at rest. The direction of rotation is defined by using the right-hand thumb rule. If the string AB is suddenly cut, assuming negligible energy dissipation, the shaft AE will