GATE ECE 2006 | GATE ECE Year Wise Previous Years Questions

GATE ECE 2006

MCQ (Single Correct Answer)

-0.6

The diagonal clipping in Amplitude Demodulation (using envelope detector) can be avoided if RC time-constant of the envelope detector satisfies the following condition, (here W is message bandwidth and ω_c is carrier frequency both in rad/sec)

$$\mathrm{RC}\;<\frac1{\mathrm W}$$

$$\mathrm{RC}\;>\frac1{\mathrm W}$$

$$\mathrm{RC}\;<\frac1{{\mathrm\omega}_\mathrm c}$$

$$\mathrm{RC}\;>\frac1{{\mathrm\omega}_\mathrm c}$$

GATE ECE 2006

MCQ (Single Correct Answer)

-0.6

Consider two transfer functions $${G_1}\left( s \right) = {1 \over {{s^2} + as + b}}$$ and $${G_2}\left( s \right) = {s \over {{s^2} + as + b}}.$$ The 3-dB bandwidths of their frequency responses are, respectively

$$\sqrt {{a^2} - 4b,} $$ $$\sqrt {{a^2} + 4b,} $$

$$\sqrt {{a^2} - 4b,} $$ $$\sqrt {{a^2} - 4b,} $$

$$\sqrt {{a^2} + 4b,} $$ $$\sqrt {{a^2} - 4b,} $$

$$\sqrt {{a^2} + 4b,} $$ $$\sqrt {{a^2} + 4b,} $$

GATE ECE 2006

MCQ (Single Correct Answer)

-0.3

In the system shown below, x(t)=(sin t). In steady-state, the response y(t) will be GATE ECE 2006 Control Systems - Frequency Response Analysis Question 61 English

GATE ECE 2006 Control Systems - Frequency Response Analysis Question 61 English

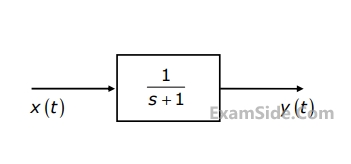
$${1 \over {\sqrt 2 }}\sin \left( {t - {\pi \over 4}} \right)$$

$${1 \over {\sqrt 2 }}\sin \left( {t + {\pi \over 4}} \right)$$

$${1 \over {\sqrt 2 }}{e^{ - t}}\sin t$$

$$\sin t - \cos t$$

GATE ECE 2006

MCQ (Single Correct Answer)

-0.3

The open-loop transfer function of a unity-gain feedback control system is given by $$G(s) = {K \over {(s + 1)(s + 2)}},$$ the gain margin of the system in dB is given by

$$\infty $$

PREVIOUS NEXT

GATE ECE Papers

2024