The gap between a moving circular plate and a stationary surface is being continuously reduced, as the circular plate comes down at a uniform speed $$V$$ towards the stationary bottom surface, as shown in the figure. In the process, the fluid contained between the two plates flows out radially. The fluid is assumed to be incompressible and inviscid.

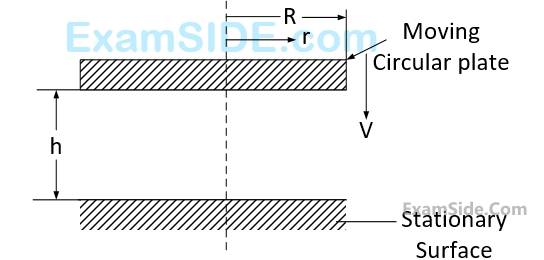
The radial component of the fluid acceleration at $$r=R$$ is

Which combination of the following statements about steady incompressible forced vortex flow is correct?

P: Shear stress is zero at all points in the flow.
Q: Velocity is directly proportional to the radius from the centre of the vortex.
R: Total mechanical energy per unit mass is constant in the entire flow field.
S: Total mechanical energy per unit mass is constant in the entire flow field.

A leaf is caught in a whirlpool. At a given instant, the leaf is at a distance of $$120$$ $$m$$ from the centre of the whirlpool. The whirlpool can be described by the following velocitry distribution ;
$${V_r} = - \left( {{{60 \times {{10}^3}} \over {2\pi r}}} \right)m/s$$
and $${V_\theta } = - \left( {{{300 \times {{10}^3}} \over {2\pi r}}} \right)m/s.$$

Where $$r$$ (in meters) is the distance from the centre of the whirlpool . What will be the distance of the leaf from the centre when it has moved through half a revolution?

A closed cylinder having a radius $$R$$ and height $$H$$ is filled with oil of density $$\rho .$$ If the cylinder is rotated about its axis at an angular velocity of $$\omega $$ , then thrust at the bottom of the cylinder is