GATE ME 2011 | Conduction Question 22 | Heat Transfer

GATE ME 2011

MCQ (Single Correct Answer)

-0.3

A pipe of $$25$$ $$mm$$ outer diameter carries steam. The heat transfer coefficient between the cylinder and surrounding is $$5$$ $$W/{m^2}K.$$ It is propsed to reduce the heat loss from the pipe by adding insulation having a thermal conductivity of $$0.05$$ $$W/mK$$. Which one of the following statements is TRUE?

The outer radius of the pipe is equal to the critical radius.

The outer radius of the pipe is less than the critical radius.

Adding the insulation will reduce the heat loss.

Adding the insulation will increase the heat loss.

GATE ME 2006

MCQ (Single Correct Answer)

-0.3

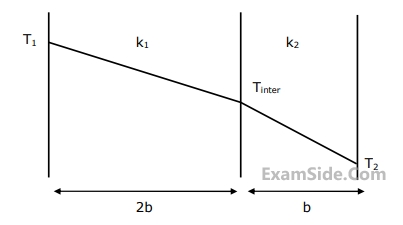
In a composite slab, the temperature at the interface (T_inter) between two materials is equal to the average of the temperatures at the two ends. Assuming steady one-dimensional heat conduction, which of the following statements is true about the respective thermal conductivities? GATE ME 2006 Heat Transfer - Conduction Question 43 English

GATE ME 2006 Heat Transfer - Conduction Question 43 English

$$2{K_1} = {K_2}$$

$${K_1} = {K_2}$$

$$2{K_1} = 3{K_2}$$

$${K_1} = 2{K_2}$$v

GATE ME 2005

MCQ (Single Correct Answer)

-0.3

In case of one dimensional heat conduction in a medium with constant properties, $$T$$ is the temperature at position $$x,$$ at time $$t.$$ Then $${{\partial T} \over {\partial t}}$$ is proportional to

$${T \over x}$$

$${{\partial T} \over {\partial x}}$$

$${{{\partial ^2}T} \over {\partial x\partial t}}$$

$${{{\partial ^2}T} \over {\partial {x^2}}}$$

GATE ME 2004

MCQ (Single Correct Answer)

-0.3

One dimensional unsteady state heat transfer equation for a sphere with heat generation at the rate $$'{q_g}',$$ can be written as

$${1 \over {r^2}}\,{\partial \over {\partial r}}\left( {r{{\partial T} \over {\partial r}}} \right) + {q \over k} = {1 \over \alpha }\,{{\partial T} \over {\partial t}}$$

$${1 \over {r^2}}\,{\partial \over {\partial r}}\left( {{r^2}{{\partial T} \over {\partial r}}} \right) + {q \over k} = {1 \over \alpha }\,{{\partial T} \over {\partial t}}$$

$${{{\partial ^2}T} \over {\partial {r^2}}} + {q \over k} = {1 \over \alpha }\,{{\partial T} \over {\partial t}}$$

$${{{\partial ^2}} \over {\partial {r^2}}}\left( {rT} \right) + {q \over k} = {1 \over \alpha }\,{{\partial T} \over {\partial t}}$$

PREVIOUS NEXT