In the following two-dimensional momentum equation for natural convection over a surface immersed in a quiescent fluid at temperature T_∞ (g is the gravitational acceleration, β is the volumetric thermal expansion coefficient, ν is the kinematic viscosity, u and v are the velocities in x and y directions, respectively, and T is the temperature)

$\rm u \frac{\partial u}{\partial x} + v \frac{\partial u}{\partial y} = g β (T - T_∞) + \nu \frac{\partial^2 u}{\partial y^2} $

the term gβ(T - T_∞) represent

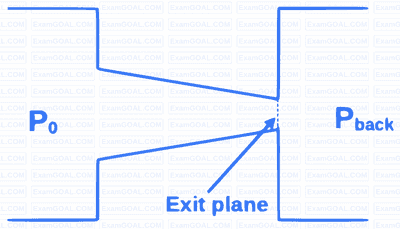
The figure shows a purely convergent nozzle with a steady, inviscid compressible flow of an ideal gas with constant thermophysical properties operating under choked condition. The exit plane shown in the figure is located within the nozzle. If the inlet pressure (P0) is increased while keeping the back pressure (Pback) unchanged, which of the following statements is/are true?

Navier Stoke's equation represents the conservation of

In a venturimeter, the angle of the diverging section is more than that of converging section.