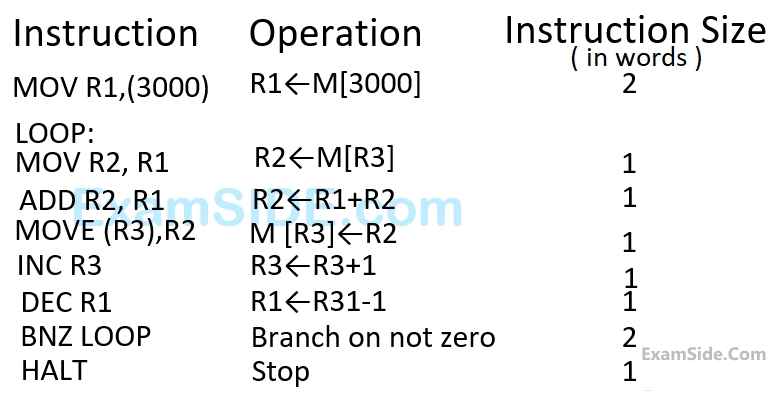
Assume that the content of memory location $$3000$$ is $$10$$ and the content of the register $$R3$$ is $$2000$$. The content of each of the memory locations from $$2000$$ to $$2010$$ is $$100.$$ The program is loaded from the memory location $$1000.$$ All the numbers are in decimal.
Assume that the memory is word addressable. The number of memory references for accessing the data in executing the program completely is

Assume that the content of memory location $$3000$$ is $$10$$ and the content of the register $$R3$$ is $$2000$$. The content of each of the memory locations from $$2000$$ to $$2010$$ is $$100.$$ The program is loaded from the memory location $$1000.$$ All the numbers are in decimal.
Assume that the memory is byte addressable and the word size is $$32$$ bits. If an interrupt occurs during the execution of the instruction $$''INC$$ $$R3'',$$ what return address will be pushed on to the stack?

Assume that the content of memory location $$3000$$ is $$10$$ and the content of the register $$R3$$ is $$2000$$. The content of each of the memory locations from $$2000$$ to $$2010$$ is $$100.$$ The program is loaded from the memory location $$1000.$$ All the numbers are in decimal.
Assume that the memory is word addressable. After the execution of this program, the content of memory location $$2010$$ is
The first operand (destination) ''$$A$$ $$\left[ {{R_0}} \right]''$$ uses indexed addressing mode with $${{R_0}}$$ as the index register. The second operand (source) $$''@B''$$ used indirect addressing mode. $$A$$ and $$B$$ are memory addresses residing at the second and the third words, respectively. The first word of the instruction specific the opcode, the index register designation and the source and destination addressing modes. During execution of $$ADD$$ instruction, the two operands are added and stored in the destination (first operand).
The number of memory cycles needed during the execution cycle of the instruction is