If the impulse response of a discrete-time system is $$h\left[ n \right]\, = \, - {5^n}\,\,u\left[ { - n\, - 1} \right],$$ then the system function $$H\left( z \right)\,\,\,$$ is equal to

Consider a sampled signal $$y\left( t \right) = 5 \times {10^{ - 6}}\,x\left( t \right)\,\,\sum\limits_{n = - \infty }^{ + \infty } {\delta \left( {t - n{T_s}} \right)} $$

where $$x\left( t \right) = 10\,\,\cos \,\left( {8\pi \times {{10}^3}} \right)\,\,t$$ and
$${T_s} = 100\,\,\mu \sec .$$ When $$y\left( t \right)$$ is passed through an ideal low-pass filter with a cutoff frequency of 5 KHz, the output of the filter is

A linear phase channel with phase delay $${\tau _p}$$ and group delay $${\tau _g}$$ must have

In Fig. m(t) = $$ = {{2\sin 2\pi t} \over t}$$, $$s(t) = \cos \,200\pi t\,\,andn(t) = {{\sin 199\pi t} \over t}$$.

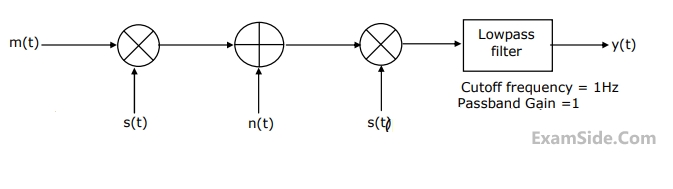
The output y(t) will be