A continuous-time system is described by $$y\left( t \right) = {e^{ - |x\left( t \right)|}},$$ where $$y(t)$$ is the output and $$x(t)$$ is the input. $$y(t)$$ is bounded

A discrete real all pass system has a pole at $$z = 2\angle {30^ \circ };\,$$ it, therefore,

$$y\left[ n \right]$$ denotes the output and $$x\left[ n \right]$$ denotes the input of a discrete-time system given by the difference equation $$y\left[ n \right] - 0.8y\left[ {n - 1} \right] = x\left[ n \right] + 1.25\,x\left[ {n + 1} \right].$$ Its right-sided impulse response is

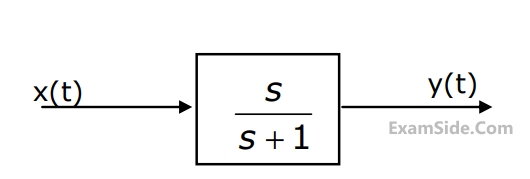
In the system shown in Fig. the input $$x\left( t \right) = \sin t.$$ In the steady-state, the response $$y(t)$$ will be