An amplifier with resistive negative feedback has two left half-plane poles in its open-loop transfer function. The amplifier

The block diagram of a feedback system is shown in Figure.
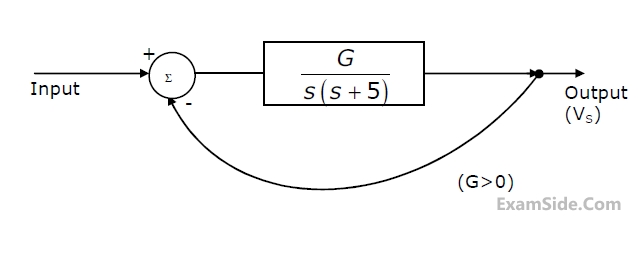
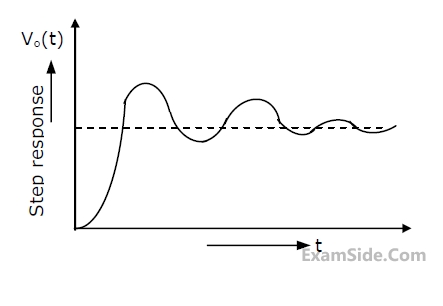
(a) Find the closed loop transfer function.
(b) Find the minimum value of G for which the step response of the system would exhibit an overshoot, as shown in Figure.
(c) For G equal to twice this minimum value, find the time period T indicated in Figure.

A system described by the transfer function $$$H\left(s\right)=\frac1{s^3+\alpha s^2+ks+3}$$$ is stable. The constraints on $$\alpha$$ and k are,

A certain linear, time-invariant system has the state and output representation shown below: $$$\eqalign{ & \left[ {\matrix{ {\mathop {{x_1}}\limits^ \bullet } \cr {\mathop {{x_2}}\limits^ \bullet } \cr } } \right] = \left[ {\matrix{ { - 2} & 1 \cr 0 & { - 3} \cr } } \right]\left[ {\matrix{ {{x_1}} \cr {{x_2}} \cr } } \right] + \left[ {\matrix{ 1 \cr 0 \cr } } \right]u \cr & y = \left( {\matrix{ 1 & 1 \cr } } \right)\left[ {\matrix{ {{x_1}} \cr {{x_2}} \cr } } \right] \cr} $$$
(a) Find the eigen values (natural frequencies) of the system.
(b)If u(t)=$$\delta \left( t \right)$$ and x₁(0⁺)=x₂(0⁺)=0, find x₁(t),x₂(t) and y(t), for t>0.
(c)When the input is zero, choose initial conditions $${x_1}\left( {{0^ + }} \right)$$ and $${x_2}\left( {{0^ + }} \right)$$ such that $$y\left( t \right) = A{e^{ - 2t}}$$ for t>0