A second order system starts with an initial condition of $$\left( {\matrix{ 2 \cr 3 \cr } } \right)$$ without any external input. The state transition matrix for the system is given by $$\left( {\matrix{ {{e^{ - 2t}}} & 0 \cr 0 & {{e^{ - t}}} \cr } } \right).$$ The state of the system at the end of $$1$$ second is given by.

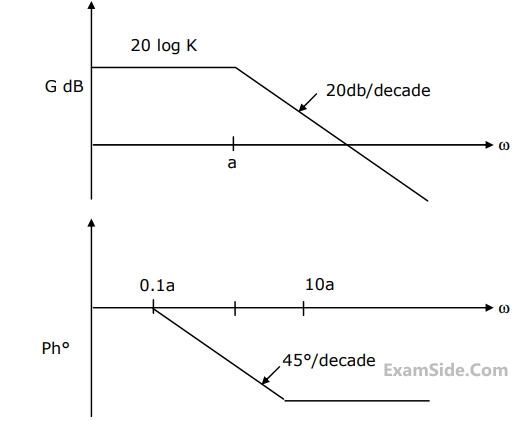
The asymptotic Bode plot of the transfer function $${K \over {1 + {s \over a}}}$$. The error in phase angle and $$dB$$ gain at a frequency of $$\omega = 0.5a$$ are respectively

The loop gain $$GH$$ of a closed loop system is given by the following expression $${K \over {s\left( {s + 2} \right)\left( {s + 4} \right)}}.$$ The value of $$K$$ for which the system just becomes unstable is

A control system with certain excitation is governed by the following mathematical equation $$${{{d^2}x} \over {d{t^2}}} + {1 \over 2}{{dx} \over {dt}} + {1 \over {18}}x = 10 + 5{e^{ - 4t}} + 2{e^{ - 5t}}$$$
The natural time constants of the response of the system are