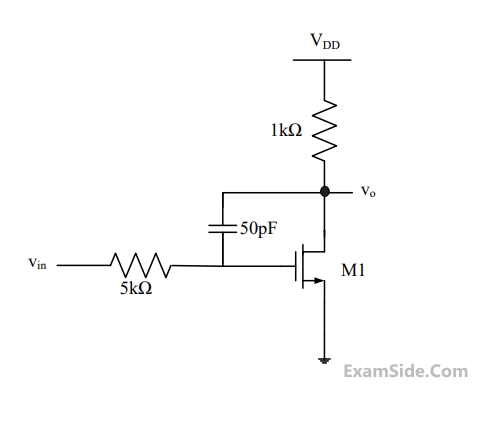
In the circuit shown in the figure, transistor M1 is in saturation and has transconductance g_m = 0.01 siemens. Ignoring internal parasitic capacitances and assuming the channel length modulation $$\lambda $$ to be zero, the small signal input pole frequency (in kHz) is _____

In the circuit shown in the figure, the channel length modulation of all transistors is non-zero $$\left( {\lambda \ne 0} \right)$$. Also all transistors operate in saturation and have negligible body effect. The ac small signal voltage gain $$\left( {{V_0}/{V_{in}}} \right)$$ of the circuit is

If the vectors $${e_1} = \left( {1,0,2} \right),\,{e_2} = \left( {0,1,0} \right)$$ and $${e_3} = \left( { - 2,0,1} \right)$$ form an orthogonal basis of the three dimensional real space $${R^3},$$ then the vectors $$u = \left( {4,3, - 3} \right) \in {R^3}$$ can be expressed as

Consider a $$2 \times 2$$ square matrix $$A = \left[ {\matrix{ \sigma & x \cr \omega & \sigma \cr } } \right]$$
Where $$x$$ is unknown. If the eigenvalues of the matrix $$A$$ are $$\left( {\sigma + j\omega } \right)$$ and $$\left( {\sigma - j\omega } \right)$$, then $$x$$ is equal to