1
MHT CET 2025 22nd April Evening Shift
MCQ (Single Correct Answer)
+1
-0
At any time ' $t$ ', the co-ordinates of moving particle are $x=a t^2$ and $y=b t^2$. The speed of the particle is
2
MHT CET 2025 21st April Evening Shift
MCQ (Single Correct Answer)
+1
-0
A body starts from rest and moves with a uniform acceleration. The ratio of the distance covered by the body in the $n^{\text {th }}$ second of its motion to the total distance travelled in n second is
3
MHT CET 2025 21st April Morning Shift
MCQ (Single Correct Answer)
+1
-0
The velocity time graph of a body moving in a straight line is shown in figure. The ratio of displacement to distance travelled by the body in time 0 to 8 s is
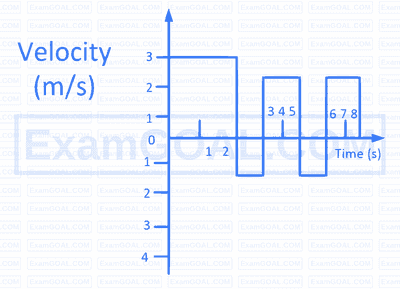
4
MHT CET 2025 20th April Evening Shift
MCQ (Single Correct Answer)
+1
-0
Two bodies A and B move in same straight line starting from same position. Body moves with constant velocity ' u ' and body B moves with constant acceleration ' $a$ '. When their velocities become equal, the distance between them is
Questions Asked from Motion (MCQ (Single Correct Answer))
Number in Brackets after Paper Indicates No. of Questions
MHT CET 2025 22nd April Evening Shift (1)
MHT CET 2025 21st April Evening Shift (1)
MHT CET 2025 21st April Morning Shift (1)
MHT CET 2025 20th April Evening Shift (2)
MHT CET 2024 16th May Evening Shift (1)
MHT CET 2024 16th May Morning Shift (1)
MHT CET 2024 15th May Evening Shift (1)
MHT CET 2024 11th May Evening Shift (1)
MHT CET 2024 11th May Morning Shift (2)
MHT CET 2024 10th May Evening Shift (1)
MHT CET 2024 9th May Evening Shift (1)
MHT CET 2024 9th May Morning Shift (1)
MHT CET 2024 4th May Evening Shift (1)
MHT CET 2024 4th May Morning Shift (2)
MHT CET 2024 2nd May Evening Shift (1)
MHT CET 2024 2nd May Morning Shift (1)
MHT CET 2023 14th May Evening Shift (1)
MHT CET 2023 13th May Evening Shift (2)
MHT CET 2023 13th May Morning Shift (2)
MHT CET 2023 12th May Evening Shift (1)
MHT CET 2023 11th May Evening Shift (1)
MHT CET 2023 11th May Morning Shift (1)
MHT CET 2023 10th May Evening Shift (1)
MHT CET 2023 10th May Morning Shift (1)
MHT CET 2022 11th August Evening Shift (1)
MHT CET 2021 23th September Morning Shift (1)
MHT CET 2021 22th September Evening Shift (1)
MHT CET 2021 21th September Evening Shift (1)
MHT CET 2021 21th September Morning Shift (1)
MHT CET 2021 20th September Evening Shift (1)
MHT CET 2021 20th September Morning Shift (1)
MHT CET 2020 16th October Morning Shift (1)
MHT CET Subjects
Physics
Mechanics
Units & Measurement and Dimensions Vector Algebra Motion Laws of Motion Circular Motion Work, Energy and Power Center of Mass and Collision Rotational Motion Gravitation Simple Harmonic Motion Fluid Mechanics Elasticity Waves Heat and Thermodynamics
Optics
Electromagnetism
Electrostatics Current Electricity Capacitor Moving Charges and Magnetism Magnetism and Matter Electromagnetic Waves Electromagnetic Induction Alternating Current
Modern Physics
Chemistry
Physical Chemistry
Some Basic Concepts of Chemistry Atomic Structure States of Matter Thermodynamics Chemical Equilibrium Ionic Equilibrium Liquid Solution Redox Reactions Surface Chemistry Solid State Electrochemistry Chemical Kinetics Nuclear Chemistry
Inorganic Chemistry
Periodic Table and Periodicity Chemical Bonding and Molecular Structure Metallurgy Hydrogen and It's Compounds s-Block Elements p-Block Elements d and f Block Elements Coordination Compounds Environmental Chemistry
Organic Chemistry
Mathematics
Algebra
Sets and Relations Logarithms Quadratic Equations Sequences and Series Binomial Theorem Permutations and Combinations Probability Vector Algebra Three Dimensional Geometry Matrices and Determinants Statistics Mathematical Reasoning Linear Programming Complex Numbers
Trigonometry
Trigonometric Ratios & Identities Trigonometric Equations Inverse Trigonometric Functions Properties of Triangles
Calculus
Functions Limits, Continuity and Differentiability Differentiation Application of Derivatives Indefinite Integration Definite Integration Area Under The Curves Differential Equations
Coordinate Geometry