Consider a binary min-heap containing 105 distinct elements. Let k be the index (in the underlying array) of the maximum element stored in the heap. The number of possible values of k is

Let A be a priority queue for maintaining a set of elements. Suppose A is implemented using a max-heap data structure. The operation EXTRACT-MAX(A) extracts and deletes the maximum element from A. The operation INSERT(A, key) inserts a new element key in A. The properties of a max-heap are preserved at the end of each of these operations.

When A contains n elements, which one of the following statements about the worst case running time of these two operations is TRUE?

Consider the C function foo and the binary tree shown.

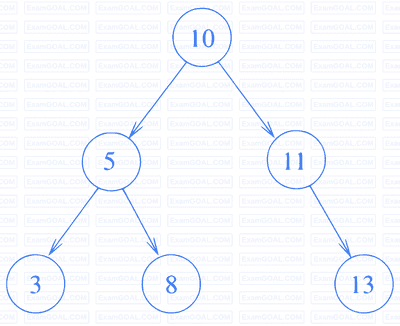
typedef struct node {
    int val;
    struct node *left, *right;
} node;
int foo(node *p) {
    int retval;
    if (p == NULL)
        return 0;
    else {
        retval = p->val + foo(p->left) + foo(p->right);
        printf("%d ", retval);
        return retval;
    }
}

When foo is called with a pointer to the root node of the given binary tree, what will it print?