1
GATE CSE 2014 Set 3
MCQ (Single Correct Answer)
+2
-0.6
Consider the pseudocode given below. The function Dosomething () takes as argument a pointer to the root of an arbitrary tree represented by the leftMostChild-rightSibling representation. Each node of the tree is of type treeNode.
typedef struct treeNode* treeptr;
Struct treeNode
{
Treeptr leftMostchild, rightSibiling;
};
Int Dosomething (treeptr tree)
{
int value =0;
if (tree ! = NULL) {
If (tree -> leftMostchild = = NULL)
value=1;
else
value = Dosomething (tree->leftMostchild);
value = value + Dosometing (tree->rightsibiling);
}
return (value);
}2
GATE CSE 2014 Set 2
Numerical
+2
-0
Consider the expression tree shown. Each leaf represents a numerical value, which can either be 0 or 1. Over all possible choices of the values at the leaves, the maximum possible value of the expression represented by the tree is ___.
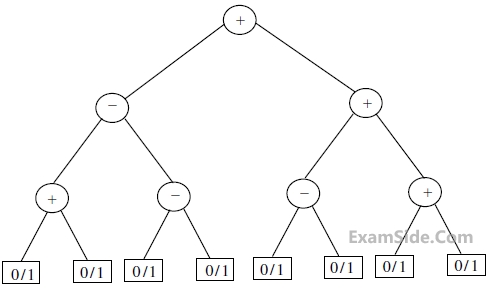

Your input ____
3
GATE CSE 2012
MCQ (Single Correct Answer)
+2
-0.6
The height of a tree is defined as the number of edges on the longest path in the tree. The function shown in the pseudocode below is invoked as height (root) to compute the height of a binary tree rooted at the tree pointer root.
int height (treeptr n)
{ if (n== NULL) return -1;
if (n-> left == NULL)
if (n-> right ==NULL) return 0;
else return B1 ; // Box 1
else {h1 = height (n -> left);
if (n -> right == NULL) return (1 + h1);
else {h2 = height (n -> right);
return B2 ; // Box 2
}
}
}4
GATE CSE 2011
MCQ (Single Correct Answer)
+2
-0.6
We are given a set of n distinct elements and an unlabeled binary tree with n nodes. In how many ways can we populate the tree with the given set so that it becomes a binary search tree?
Questions Asked from Trees (Marks 2)
Number in Brackets after Paper Indicates No. of Questions
GATE CSE 2025 Set 2 (1)
GATE CSE 2024 Set 2 (1)
GATE CSE 2024 Set 1 (1)
GATE CSE 2023 (2)
GATE CSE 2020 (2)
GATE CSE 2019 (1)
GATE CSE 2016 Set 2 (2)
GATE CSE 2014 Set 3 (3)
GATE CSE 2014 Set 2 (1)
GATE CSE 2012 (1)
GATE CSE 2011 (1)
GATE CSE 2009 (1)
GATE CSE 2008 (5)
GATE CSE 2007 (4)
GATE CSE 2006 (5)
GATE CSE 2005 (6)
GATE CSE 2004 (3)
GATE CSE 2002 (1)
GATE CSE 2000 (1)
GATE CSE 1998 (1)
GATE CSE 1997 (1)
GATE CSE 1996 (2)
GATE CSE 1991 (1)
GATE CSE Subjects
Theory of Computation
Operating Systems
Algorithms
Database Management System
Data Structures
Computer Networks
Software Engineering
Compiler Design
Web Technologies
General Aptitude
Discrete Mathematics
Programming Languages