GATE EE 2002 | GATE EE Year Wise Previous Years Questions

GATE EE 2002

Subjective

-0

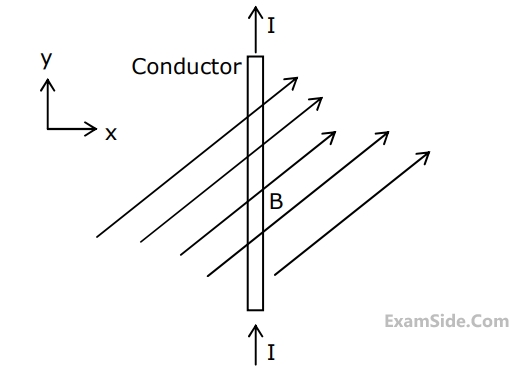
The magnetic vector potential in a region is defined by $$\overrightarrow A = {e^{ - y}}\sin \left( x \right){\widehat a_z}.$$ An infinitely long conductor, having a cross section area, $$a=5$$ $$m{m^2}$$ and carrying a $$dc$$ current, $${\rm I} = 5\,A$$ in the $$Y$$ direction, passes through this region as shown in Fig. Determine the expression for $$(a)$$ $$\overrightarrow B $$ and $$(b)$$ force density $$\overrightarrow f $$ exerted on the conductor GATE EE 2002 Electromagnetic Fields - Magnetostatics Question 6 English

GATE EE 2002

MCQ (Single Correct Answer)

-0.3

Let $$Y(s)$$ be the Laplace transform of function $$y(t),$$ then the final value of the function is __________.

$$\mathop {Lim}\limits_{s \to 0} \,\,Y\left( s \right)$$

$$\mathop {Lim}\limits_{s \to \infty } \,\,Y\left( s \right)$$

$$\mathop {Lim}\limits_{s \to 0} \,s\,\,Y\left( s \right)$$

$$\mathop {Lim}\limits_{s \to \infty } \,s\,\,Y\left( s \right)$$

GATE EE 2002

MCQ (Single Correct Answer)

-0.3

Given a vector field $${\overrightarrow F ,}$$ the divergence theorem states that

$$\int\limits_s {\overrightarrow F .d\overrightarrow s = \int\limits_v \nabla .\overrightarrow F \,dv} $$

$$\int\limits_s {\overrightarrow F .d\overrightarrow s = \int\limits_v \nabla \times \overrightarrow F \,dv} $$

$$\int\limits_s {\overrightarrow F \times d\overrightarrow s = \int\limits_v \nabla .\overrightarrow F \,dv} $$

$$\int\limits_s {\overrightarrow F \times d\overrightarrow s = \int\limits_v \nabla \times \overrightarrow F \,dv} $$

GATE EE 2002

MCQ (Single Correct Answer)

-0.3

The determinant of the matrix $$\left[ {\matrix{ 1 & 0 & 0 & 0 \cr {100} & 1 & 0 & 0 \cr {100} & {200} & 1 & 0 \cr {100} & {200} & {300} & 1 \cr } } \right]$$ is

$$100$$

$$200$$

$$1$$

$$300$$

PREVIOUS NEXT

GATE EE Papers

2024