There is no value of $$x$$ that can simultaneously satisfy both the given equations. Therefore, find the 'least squares error' solution to the two equations, i.e., find the value of $$x$$ that minimizes the sum of squares of the errors in the two equations
$$2x=3$$
$$4x=1$$

The square root of a number $$N$$ is to be obtained by applying the Newton $$-$$ Raphson iteration to the equation $$\,{x^2} - N = 0.\,\,$$ If $$i$$ denotes the iteration index, the correct iterative scheme will be

The table below gives values of a function $$f(x)$$ obtained for values of $$x$$ at intervals of $$0.25$$

The value of the integral of the function between the limits $$0$$ to $$1,$$ using Simpson's rule is

The area under the curve shown between $$x=1$$ and $$x=5$$ is to be evaluated using the trapezoidal rule. The following points on the curve are given

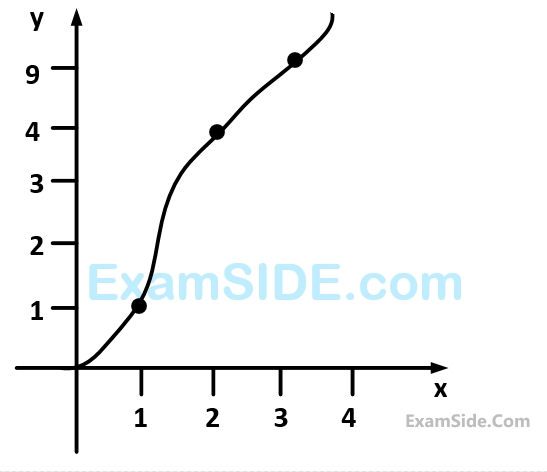
The evaluated area (In m²) will be