1
GATE EE 2007
MCQ (Single Correct Answer)
+2
-0.6
A three phase balanced star connected voltage source with frequency $$\omega \,\,rad/s$$ is connected to a star connected balanced load which is purely inductive. The instantaneous line currents and phase to neutral voltages are denoted by $$\left( {{i_a},{i_b},{i_c}} \right)$$ and $$\left( {{V_{an}},\,\,{V_{bn}},\,\,{V_{cn}}} \right)$$ respectively and their $$rms$$ values are denoted by $$V$$ and $$1.$$ If
$$$R = \left[ {{V_{an}}\,\,{V_{bn}}\,\,{V_{cn}}} \right]\left[ {\matrix{
0 & {{1 \over {\sqrt 3 }}} & { - {1 \over {\sqrt 3 }}} \cr
{ - {1 \over {\sqrt 3 }}} & 0 & {{1 \over {\sqrt 3 }}} \cr
{{1 \over {\sqrt 3 }}} & { - {1 \over {\sqrt 3 }}} & 0 \cr
} } \right]\left[ {\matrix{
{{i_a}} \cr
{{i_b}} \cr
{{i_c}} \cr
} } \right],$$$
then the magnitude of $$R$$ is
then the magnitude of $$R$$ is
2
GATE EE 2007
MCQ (Single Correct Answer)
+2
-0.6
The resonant frequency for the given circuit will be
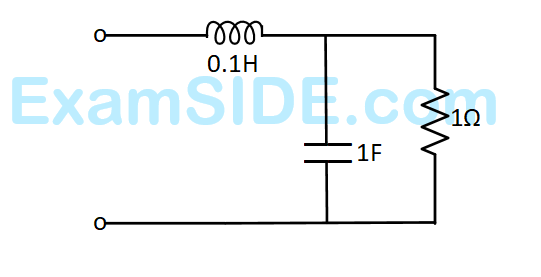

3
GATE EE 2007
MCQ (Single Correct Answer)
+2
-0.6
In the figure given below, all phasors are with reference to the potential at point $$''O''.$$ The locus of voltage phasor $${V_{YX}}$$ as $$R$$ is varied from zero to infinity is shown by
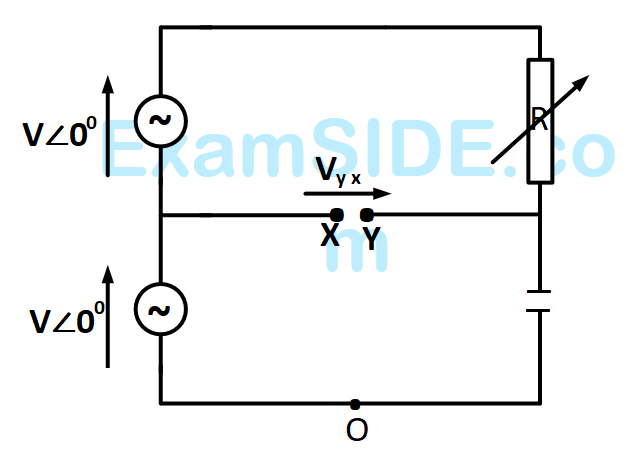

4
GATE EE 2007
MCQ (Single Correct Answer)
+2
-0.6
The $$R-L-C$$ series circuit shown is supplied from a variable frequency voltage source. The admittance $$-$$ locus of the $$R-L$$ $$-C$$ network at terminals $$AB$$ for increasing frequency $$\omega $$ is
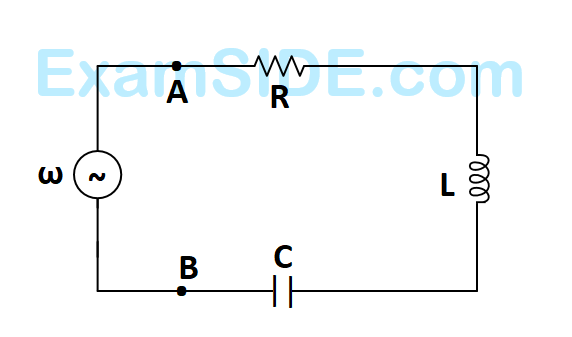

Paper analysis
Total Questions
Analog Electronics
6
Control Systems
8
Digital Electronics
5
Electric Circuits
8
Electrical and Electronics Measurement
2
Electrical Machines
10
Electromagnetic Fields
4
Engineering Mathematics
8
Power Electronics
11
Power System Analysis
10
Signals and Systems
8
More papers of GATE EE
GATE EE 2025
GATE EE 2024
GATE EE 2023
GATE EE 2022
GATE EE 2021
GATE EE 2020
GATE EE 2019
GATE EE 2018
GATE EE 2017 Set 2
GATE EE 2017 Set 1
GATE EE 2016 Set 2
GATE EE 2016 Set 1
GATE EE 2015 Set 1
GATE EE 2015 Set 2
GATE EE 2014 Set 2
GATE EE 2014 Set 3
GATE EE 2014 Set 1
GATE EE 2013
GATE EE 2012
GATE EE 2011
GATE EE 2010
GATE EE 2009
GATE EE 2008
GATE EE 2007
GATE EE 2006
GATE EE 2005
GATE EE 2004
GATE EE 2003
GATE EE 2002
GATE EE 2001
GATE EE 2000
GATE EE 1999
GATE EE 1998
GATE EE 1997
GATE EE 1996
GATE EE 1995
GATE EE 1994
GATE EE 1993
GATE EE 1992
GATE EE 1991
GATE EE
Papers
2025
2024
2023
2022
2021
2020
2019
2018
2013
2012
2011
2010
2009
2008
2007
2006
2005
2004
2003
2002
2001
2000
1999
1998
1997
1996
1995
1994
1993
1992
1991







