ExamSIDE (Powered by ExamGOAL)
Questions
PREVIOUS NEXT
1
GATE CE 2024 Set 2
Numerical
+2
-0.833

For the 6m long horizontal cantilever beam PQR shown in the figure, Q is the midpoint. Segment PQ of the beam has flexural rigidity $EI = 2 \times 10^5$ kN. $m^2$ whereas the segment QR has infinite flexural rigidity. Segment QR is subjected to uniformly distributed, vertically downward load of 5 kN/m.

GATE CE 2024 Set 2 Strength of Materials Or Solid Mechanics - Deflection of Beams Question 2 English

The magnitude of the vertical displacement (in mm) at point Q is _______ (rounded off to 3 decimal places).

Your input ____
2
GATE CE 2017 Set 2
MCQ (Single Correct Answer)
+2
-0.6
Two prismatic beams having the same flexural rigidity of $$1000\,kN$$-$${m^2}$$ are shown in the figures. GATE CE 2017 Set 2 Strength of Materials Or Solid Mechanics - Deflection of Beams Question 5 English

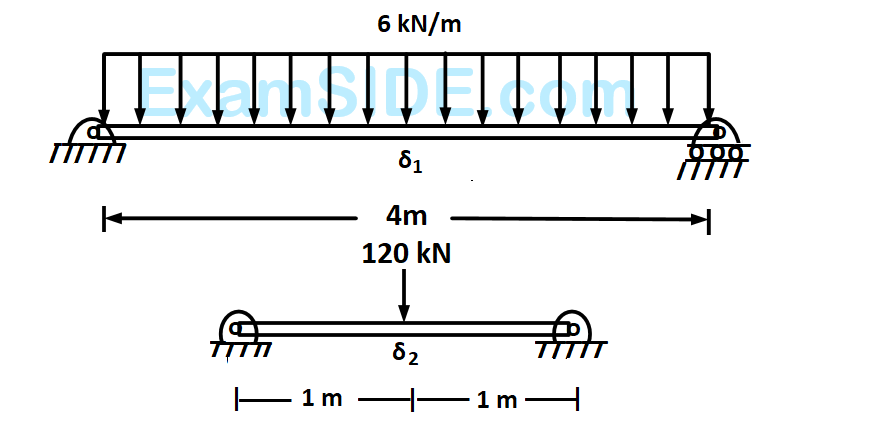
If the mid-span deflections of these beams are denoted by $${\delta _1}$$ and $${\delta _2}$$ (as indicated in the figures), the correct option is

A
$${\delta _1} = {\delta _2}$$
B
$${\delta _1} < {\delta _2}$$
C
$${\delta _1} > {\delta _2}$$
D
$${\delta _1} > > \delta $$
3
GATE CE 2016 Set 1
MCQ (Single Correct Answer)
+2
-0.6
A $$3$$ $$m$$ long simply supported beam of uniform cross section is subjected to a uniformly distributed load of $$w = 20$$ $$kN/m$$ in the central $$1$$ $$m$$ as shown in the figure. GATE CE 2016 Set 1 Strength of Materials Or Solid Mechanics - Deflection of Beams Question 20 English

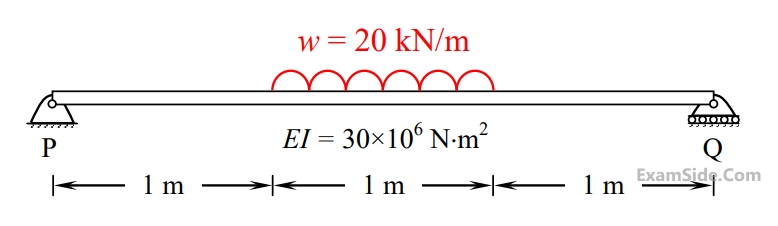
If the flexural rrigidity $$(EI)$$ of the beam is $$30 \times {10^6}\,\,N$$ - $${m^2},$$ the maximum slope (expressed in radians) of the deformed beam is

A
$$0.681 \times {10^{ - 7}}$$
B
$$0.943 \times {10^{ - 7}}$$
C
$$4.310 \times {10^{ - 7}}$$
D
$$3.610 \times {10^{ - 4}}$$
4
GATE CE 2015 Set 2
Numerical
+2
-0
A simply supported reinforced concrete beam of length $$10$$ $$m$$ sags while undergoing shrinkage. Assuming a uniform curvature of $$0.004\,\,{m^{ - 1}}$$ along the span, the maximum deflection (in $$m$$) of the beam at mid-span is ________
Your input ____
PREVIOUS NEXT
GATE CE Subjects
Construction Material and Management
Critical Path Method Construction Materials Program Evolution Review Technique
Geomatics Engineering Or Surveying
Levelling Traversing Theodolites and Plane Table Surveying Measurement of Area, Volume and Theory of Errors and Survey Adjustment Field Astronomy and Photogrammetric Surveying Basics of GIS, GPS and Remote Sensing Angular Measurements and Compass Survey Basic Concepts Linear Measurements and Chain Survey
Engineering Mechanics
Equilibrium of Force Systems Work Energy Principle and Impulse Momentum Equation
Hydrology
Precipitation and Frequency of Rainfall Data Evaporation and Transpiration Infiltration Hydrograph Flood Estimation and Flood Routing
Transportation Engineering
Highway Devolopment and Planning Highway Geometric Design Traffic Engineering Highway Materials Railway, Runway and Taxiway Design
Strength of Materials Or Solid Mechanics
Centroid and Moment of Inertia Torsion Pure Bending Shear Stress In Beams Strain Energy Method Columns and Struts Complex Stress Deflection of Beams Thin Cylinder Simple Stresses Shear Force and Bending Moment Propped Cantilever Beam
Reinforced Cement Concrete
Doubly Reinforced Sections Limit State of Collapse Shear Footings Bond Slabs Limit State of Collapse Torsion Limit State of Serviceability Prestressed Concrete Singly Reinforced Sections Workmenship and Fundamentals Flanged Beams Limit State of Collapse Compression
Steel Structures
Materials and Specifications Tension Members Riveted Joints and Bolted Joints Welded Connections Eccentric Connections Compression Members Beams Plate Girder
Irrigation
Water Requirement of Crops Gravity Dams and Spillways
Environmental Engineering
Sources and Coveyances Water Quality Control of Water Treatment of Water Water Demand Waste Water Characteristics Treatment of Sewage Disposal of Sewage Effluent Solid Waste Management Air Pollution Noise Pollution
Engineering Mathematics
Probability and Statistics Differential Equations Transform Theory Calculus Complex Variable Linear Algebra Vector Calculus Numerical Methods
Structural Analysis
Energy Principle Truss Stability and Static Indeterminacy Methods of Analysis Indeterminacy Arches and Cable Slope Deflection Method Matrix Method Moment Distribution Method Influence Line Diagram Plastic Analysis
Geotechnical Engineering
Origin of Soils Definitions and Properties of Soils Effective Stress and Permeability Compaction of Soil Compressibility and Consolidation Shear Strength of Soil Stress Distribution of Soil Retaining Wall and Earth Pressure Stability of Slopes Shallow Foundation Pile Foundation
Fluid Mechanics and Hydraulic Machines
Fluid Pressure and Measurement Boundary Layer Theory Drag and Lift Open Channel Flow Hydraulic Machines Properties of Fluids Fluid Statics
General Aptitude
Numerical Ability Logical Reasoning Verbal Ability
EXAM MAP
Joint Entrance Examination
JEE MainJEE AdvancedWB JEEBITSATMHT CETKCETCOMEDKVITEEEAP EAPCET
Medical
NEETAIIMS
Graduate Aptitude Test in Engineering
GATE CSEGATE ECEGATE EEGATE MEGATE CEGATE PIGATE IN
Civil Services
UPSC Civil Service
Defence
NDA
Staff Selection Commission
SSC CGL Tier I
CBSE
Class 12