GATE EE 2006 | GATE EE Year Wise Previous Years Questions

GATE EE 2006

MCQ (Single Correct Answer)

-0.6

A student has made a-$$3$$ bit binary down counter and connected to the $$R$$-$$2R$$ ladder type $$DAC$$ [Gain $$=$$ $$\left( { - 1k\Omega /2R} \right)$$ as shown in figure to generate a staircase waveform.

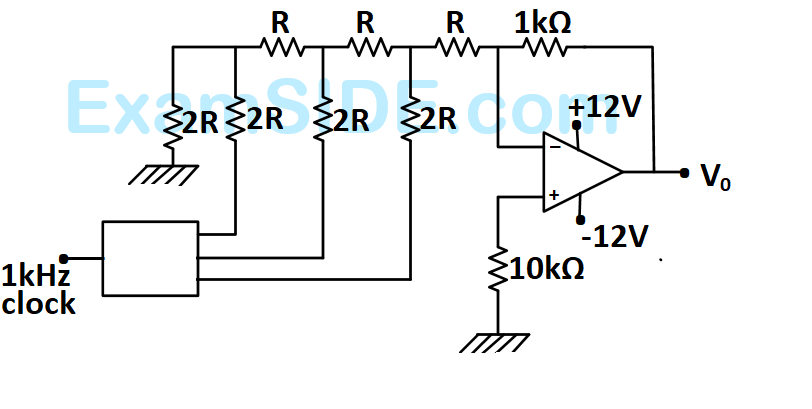
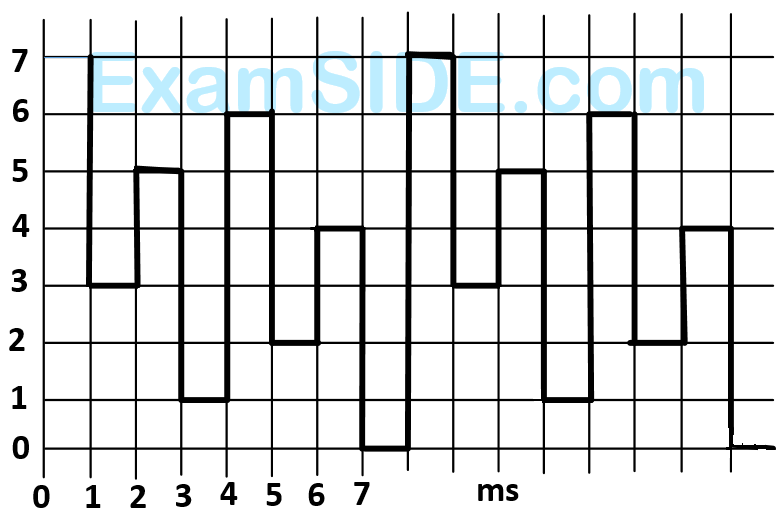
The output achieved is different as shown in figure. What could be the possible cause of this error?

GATE EE 2006 Digital Electronics - Analog to Digital and Digital to Analog Converter Question 2 English 1

GATE EE 2006 Digital Electronics - Analog to Digital and Digital to Analog Converter Question 2 English 2

The resistance values are incorrect

The counter is not working property

The connection from the counter to $$DAC$$ is not proper

The $$R$$ and $$2R$$ resistances are interchanged

GATE EE 2006

MCQ (Single Correct Answer)

-0.6

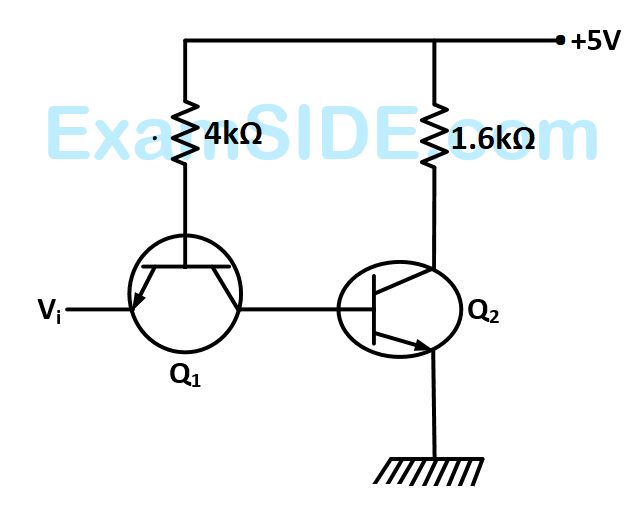
A TTL NOT gate circuit is shown in figure. Assuming $${V_{BE}} = 0.7\,v$$ of both the transistors, if $${V_i} = 3.0\,V,$$ then the states of the two transistors will be GATE EE 2006 Digital Electronics - Logic Families and Memories Question 1 English

GATE EE 2006 Digital Electronics - Logic Families and Memories Question 1 English

$${Q_1}\,\,ON$$ and $${Q_2}\,\,OFF$$

$${Q_1}$$ reverse $$\,\,ON$$ and $${Q_2}\,\,OFF$$

$${Q_1}$$ reverse $$\,\,ON$$ and $${Q_2}\,\,ON$$

$${Q_1}\,\,OFF$$ and $${Q_2}$$ reverse $$\,\,ON$$

GATE EE 2006

MCQ (Single Correct Answer)

-0.6

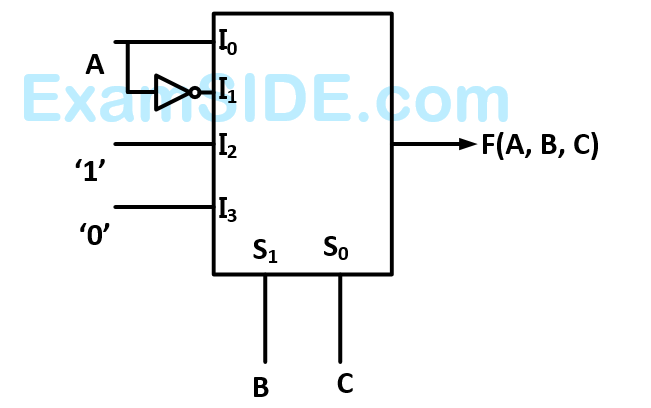
A $$4 \times 1\,\,MUX$$ is used to implement a $$3$$- input Boolean function as shown in figure. The Boolean function $$F\left( {A,\,\,B,\,\,C} \right)$$ implemented is GATE EE 2006 Digital Electronics - Combinational Circuits Question 3 English

GATE EE 2006 Digital Electronics - Combinational Circuits Question 3 English

$$F\left( {A,\,\,B,\,\,C} \right) = \sum \left( {1,\,\,2,\,\,4,\,\,6} \right)$$

$$F\left( {A,\,\,B,\,\,C} \right) = \sum \left( {1,\,\,2,\,\,6} \right)$$

$$F\left( {A,\,\,B,\,\,C} \right) = \sum \left( {2,\,\,4,\,\,5,\,\,6} \right)$$

$$F\left( {A,\,\,B,\,\,C} \right) = \sum \left( {1,\,\,5,\,\,6} \right)$$

GATE EE 2006

MCQ (Single Correct Answer)

-0.3

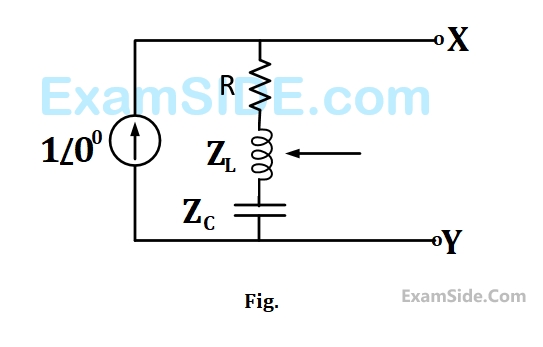
In the figure the current source is $$1\,\,\angle \,0\,A,$$ $$R = \,1\,\,\Omega ,$$ the impedances are $${Z_C} = - j\,\,\Omega ,$$ and $${Z_L} = 2\,j\,\,\Omega .$$ The Thevenin equivalent looking into the circuit across $$X-Y$$ is. GATE EE 2006 Electric Circuits - Network Theorems Question 13 English

GATE EE 2006 Electric Circuits - Network Theorems Question 13 English

$$\sqrt 2 \,\,\angle 0,\,\,V,\,\,\left( {1 + 2j} \right)\,\,\Omega $$

$$2\,\,\angle {45^ \circ },\,\,V,\,\,\left( {1 - 2j} \right)\,\,\Omega $$

$$2\,\,\angle {45^ \circ },\,\,V,\,\,\left( {1 + j} \right)\,\,\Omega $$

$$\sqrt 2 \,\,\angle {45^ \circ },\,\,V,\,\,\left( {1 + j} \right)\,\,\Omega $$

PREVIOUS NEXT

GATE EE Papers

2024