A three-hinge arch ABC in the form of a semi-circle is shown in the figure. The arch is in static equilibrium under vertical loads of $P = 100$ kN and $Q = 50$ kN. Neglect friction at all the hinges. The magnitude of the horizontal reaction at B is _________ kN (rounded off to 1 decimal place).

The velocity field of a two-dimensional, incompressible flow is given by $\overrightarrow{V} = \ 2sin{h}\,x\,\hat{i} + v(x,y)\hat{j}$ where $ \hat{i}$ and $\underset{\dot{}}{j}$ denote the unit vectors in x and y directions, respectively. If $v(x, 0) = cosh\ x$, then $v(0,-1)$ is
In the pipe network shown in the figure, all pipes have the same cross-section and can be assumed to have the same friction factor. The pipes connecting points W, N, and S with point J have an equal length L. The pipe connecting points J and E has a length 10L. The pressures at the ends N, E, and S are equal. The flow rate in the pipe connecting W and J is Q. Assume that the fluid flow is steady, incompressible, and the pressure losses at the pipe entrance and junction are negligible. Consider the following statements:
I : The flow rate in pipe connecting J and E is Q/21.
II: The pressure difference between J and N is equal to the pressure difference between J and E.
$$ \text { Which one of the following options is CORRECT? } $$
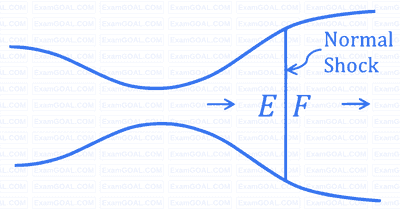
Steady, compressible flow of air takes place through an adiabatic converging-diverging nozzle, as shown in the figure. For a particular value of pressure difference across the nozzle, a stationary normal shock wave forms in the diverging section of the nozzle. If $E$ and $F$ denote the flow conditions just upstream and downstream of the normal shock, respectively, which of the following statement(s) is/are TRUE?