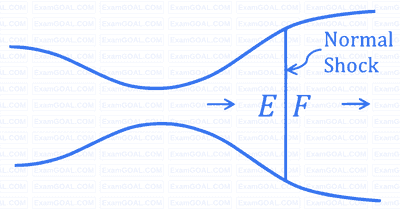
Steady, compressible flow of air takes place through an adiabatic converging-diverging nozzle, as shown in the figure. For a particular value of pressure difference across the nozzle, a stationary normal shock wave forms in the diverging section of the nozzle. If $E$ and $F$ denote the flow conditions just upstream and downstream of the normal shock, respectively, which of the following statement(s) is/are TRUE?

A liquid fills a horizontal capillary tube whose one end is dipped in a large pool of the liquid. Experiments show that the distance $L$ travelled by the liquid meniscus inside the capillary in time $t$ is given by

$L = k \gamma^a R^b \mu^c \sqrt{t},$

where $ \gamma $ is the surface tension, $ R $ is the inner radius of the capillary, and $ \mu $ is the dynamic viscosity of the liquid. If $ k $ is a dimensionless constant, then the exponent $ a $ is _______ (rounded off to 1 decimal place).

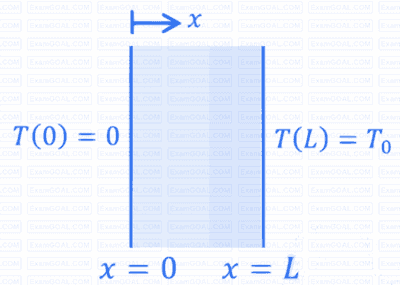
A plane, solid slab of thickness L, shown in the figure, has thermal conductivity k that varies with the spatial coordinate x as k = A + Bx, where A and B are positive constants (A > 0, B > 0). The slab walls are maintained at fixed temperatures of T(x = 0) = 0 and T(x = L) = T₀ > 0. The slab has no internal heat sources. Considering one-dimensional heat transfer, which one of the following plots qualitatively depicts the steady-state temperature distribution within the slab?

Consider incompressible laminar flow over a flat plate with freestream velocity of $u_{\infty}$. The Nusselt number corresponding to this flow velocity is $Nu_1$. If the freestream velocity is doubled, the Nusselt number changes to $Nu_2$. Choose the correct option for $Nu_{2}/Nu_{1}$.