For the following set of simultaneous equations $$$1.5x - 0.5y + z = 2$$$ $$$4x + 2y + 3z = 9$$$ $$$7x + y + 5z = 10$$$

Area bounded by the curve $$y = {x^2}$$ and the lines $$x=4$$ and $$y=0$$ is given by

The order of error in the simpson's rule for numerical integration with a step size $$h$$ is

Solve the initial value problem
$${{{d^2}y} \over {d{x^2}}} - 4{{dy} \over {dx}} + 3y = 0$$ with $$y=3$$ and
$${{dy} \over {dx}} = 7$$ at $$x=0$$ using the laplace transform technique?

A mass of 35 kg is suspended from a weightless bar AB which is supported by a cable CB and a pin at A as shown in. The pin reactions at A on the bar AB are

The dimension of surface tension is :

Refer to figure, the absolute pressure of gas $$A$$ in the bulb is

In certain $$HE,$$ both the fluids have identical mass flow rate-specific heat product. The hot fluid enters at $${76^ \circ }C$$ and leaves at $${47^ \circ }C$$ and the cold fluid entering at $${28^ \circ }C$$ leave at $${55^ \circ }C$$. The effectiveness of the heat exchanger $$(HE)$$ is

In a certain double pipe heat exchanger hot water flows at a rate of $$50,000$$ $$kg/h$$ and gets cooled from $${95^ \circ }C$$ to $${65^ \circ }C$$. At the same time $$50,000$$ $$kg/h$$ of cooling water at $${30^ \circ }C$$ enters the heat exchanger. The flow conditions are such that the overall heat transfer coefficient remains constant at $$2270$$ $$W/{m^2}K$$. Calculate the heat transfer area required, assuming the two streams are in parallel flow, and for both the streams $${C_p} = 4.2\,\,kJ/kg\,\,K$$

Setup costs do not include

A dummy activity is used in $$PERT$$ network to describe

Determine the number of production runs and also the total incremental cost in a factory for the data given below:

A project plan is given below:

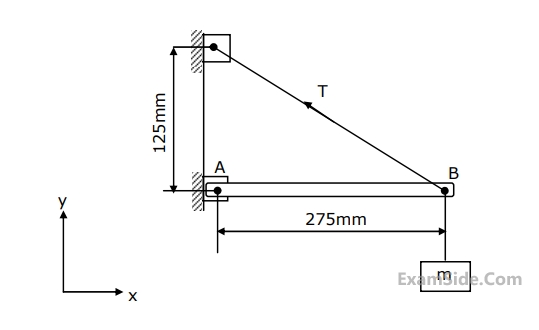
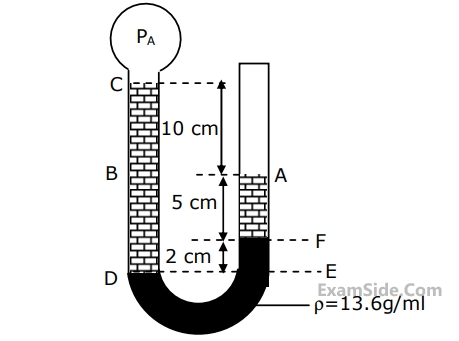
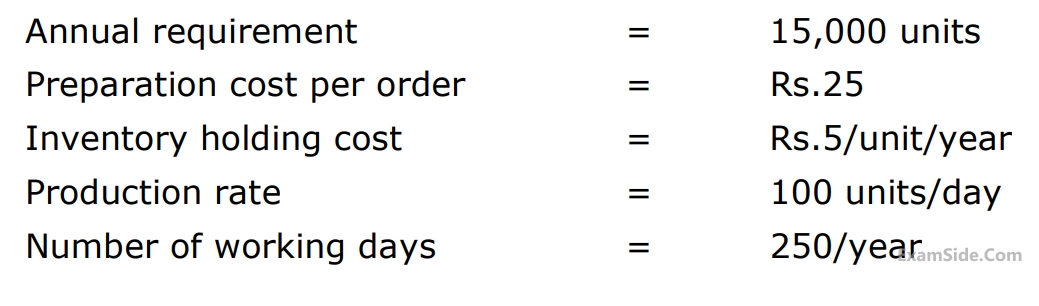
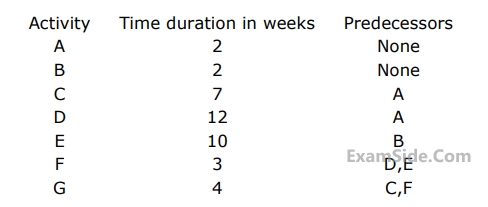
(a) Construct a $$PERT$$ network
(b) Find the critical path and estimate the project duration.

The most commonly used criteria for measuring forecast error is

In a forecasting model, at the end of period $$13,$$ the forecasted value for period $$14$$ is $$75.$$ Actual value in the periods $$14$$ to $$16$$ are constant at $$100$$. if the assumed simple exponential smoothing parameter is $$0.5,$$ then the $$MSE$$ at the end of period $$16$$ is

The cost of providing service in a queuing system increases with

People arrive at a hotel in a Poisson distributed arrival rate of $$8$$ per hour. Service time distribution is closely approximated by the negative exponential. The average service time is $$5$$ minutes. Calculate (a) the mean number in the waiting line; (b) the mean number in the system; (c) the waiting time in the queue; (d) the mean time in the system, and (e) the utilization factor.

A company rents three warehouses $$A, B$$ and $$C$$ from which they supply bearings to two customers $$P$$ and $$Q.$$ The profit per piece, the annual demands of the customers and the supplies available from the warehouses are shown below:

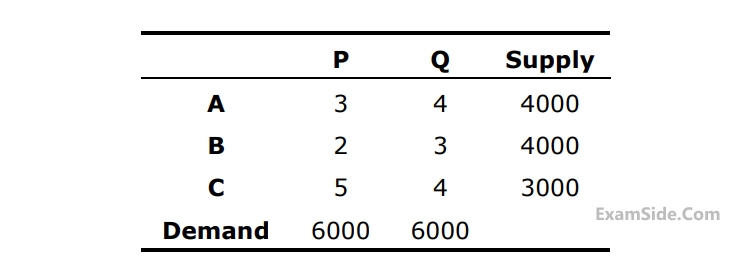
As the company is not able to meet the demands, it is proposed to replace warehouse $$C$$ with a warehouse rented at $$D$$ whose supply capacity would be $$5000.$$ The expected profit would be Rs.$$6$$ and Rs.$$5$$ per piece distributed to $$P$$ and $$Q$$ respectively. The rental charges for warehouse $$D$$ is Rs.$$5,000$$ per year. Find the increase in the profit of the company after replacing warehouse $$C$$ by $$D.$$

A small element at the critical section of a component in bi-axial state of stress with the two principal stresses being $$360$$ $$MPa$$ and $$140$$ $$MPa.$$ The maximum working stress according to distortion energy theory is

To make a worm drive reversible, it is necessary to increase

Match the following
List - $${\rm I}$$
(a)$$\,\,\,\,\,$$ Automobile wheel mounting on axle
(b)$$\,\,\,\,\,$$ High speed grinding spindle
(c)$$\,\,\,\,\,$$ $${\rm IC}$$ - Engine connecting rod
(d)$$\,\,\,\,\,$$ Leaf spring eye mounting

List - $${\rm II}$$
1.$$\,\,\,\,\,$$ Magneto bearing
2.$$\,\,\,\,\,$$ Angular contact bearing
3.$$\,\,\,\,\,$$ Taper roller bearing
4.$$\,\,\,\,\,$$ Hydrodynamic journal bearing
5.$$\,\,\,\,\,$$ Sintered metal bearing
6.$$\,\,\,\,\,$$ Teflon/Nylon bush

The dynamic load capacity of $$6306$$ bearing is $$22KN.$$ The maximum radial load it can sustain to operate at $$600$$ $$rev/min,$$ for $$2000$$ hours is

To restore stable operating condition in a hydrodynamic journal bearing, when it encounters higher magnitude loads.

$$3$$ blocks of $${B_1},\,\,{B_2},\,\,{B_3}$$ are to be inserted in a channel of width $$'S'$$ maintaining a minimum gap of width $$T=0.125mm,$$ as shown in fig. For $$P = 18.75 \pm 0.08,\,$$ $$\,Q = 25.00 \pm 0.12,\,$$ $$\,R = 28.125 \pm 0.1$$ and $$S=72.35+X.$$ The tolerance $$' X '$$ is _______________.

A metal strip is to be rolled from an initial wrought thickness of $$3.5$$ $$mm$$ to a final rolled thickness of $$2.5$$ $$mm$$ in a single pass rolling mill having rolls of $$250$$ $$mm$$ diameter. The strip is $$450$$ $$mm$$ wide. The average coefficient of friction in the roll gap is $$0.08$$. Taking plane strain low stress of $$140$$ $$MPa,$$ for the metal and assuming negligible spreading, the roll separating force is . . . . . . . . .

Welding of $$C40$$ steel plate of $$10mm$$ thickness required a current of $$16$$amps, while it was $$360$$amps when the plate thickness was increased by $$50\% $$. Estimate the welding current for $$8mm$$ thickness of same material.

In a typical metal cutting operation, using a cutting tool of positive rake $$\gamma = 10\deg ,$$ it was observed that the shear angle was $$20deg$$. The friction angle is

A cutting tool has a nose radius of $$1.8mm$$. The feed rate for a theoretical surface roughness of $${R_t} = 5$$ microns is

In a turning trail using orthogonal tool geometry, a chip length of $$84mm$$ was obtained for an uncut chip length of $$200mm.$$ The cutting conditions were $$V=30m/min,$$ $${t_1} = 0.5mm,$$ rake angle $$=20deg,$$ cutting tool is $$HSS.$$ Estimate shear plane angle, chip thickness and the shear plane angle for minimum chip strain.

A Throwaway carbide insert was used to machine a steel work pieces with a cutting speed of $$60$$ $$m/min,$$ tool life of $$40$$ minutes was observed, when the cutting speed was increased to $$100$$ $$m/min,$$ the tool life decreased to $$10$$ minutes. The cutting speed for maximum productivity, if tool change time is $$2$$ minutes is

Helix angle of fast helix drill is normally

In a point to point control $$NC$$ machine, the slides are positioned by an integrally mounted stepper motor drive. If the specification of the motor is $$1$$ deg/pulse, and the pitch of the lead screw is $$3.6$$ $$mm,$$ what is the expected positioning accuracy?

A mass of 35 kg is suspended from a weightless bar AC, which is supported by a cable CB and a pin at A as shown in Fig. below. The pin reactions at A on the bar AB are

The suspension system of a two-wheeler can be equated to a single spring-mass system with a viscous damper connected in series. Sketch the free body diagram and give the equations of motion. For a mass $$m = 50$$ $$kg$$ and a spring with a stiffness of $$35$$ $$kN/m,$$ determine what should be the damping coefficient (damping constant) for critical damping. What can be the damping force for a plunger velocity of $$0.05$$ $$m/s$$?

Consider an actual regenerative Rankine cycle with one open feed water heater. For each $$kg$$ steam entering the turbine, if $$m$$ $$kg$$ steam with a specific enthalpy of $${h_1}$$ is bled from the turbine, and the specific enthalpy of liquid water entering the heater is $${h_2},$$ then $${h_3},$$ the specific enthalpy of saturated liquid leaving the heater is equal to

The following data pertain to a single stage impulse steam turbine:
Nozzle angle $$=$$ $${20^ \circ };$$
Blade velocity $$= 200$$ $$m/s;$$
Relative steam velocity at entry $$= 350$$ $$m/s;$$
Blade inlet $$30;$$
Blade exit angle $$ = {25^ \circ }.$$

If blade friction is neglected, the work done per $$kg$$ steam is

Match the following

Which among the following is the boiler mounting?

Air enters a frictionless adiabatic converging nozzle at $$10$$ bar, $$500$$ $$K$$ with negligible velocity. The nozzle dis-charges to a region at $$2$$ bar. If the exit area of the nozzle is $$2.5\,c{m^2}$$ find the flow rate of air through the nozzle. Assume for air, $${C_p} = 1005\,J/kg\,K$$ and $${C_v} = 718\,J/kg\,K.$$

For an ideal gas the expression $$\left[ {T{{\left( {{{\delta s} \over {\delta T}}} \right)}_p} - T{{\left( {{{\delta s} \over {\delta T}}} \right)}_v}} \right]$$ is always equal to :

A system undergoes a state change from $$1$$ to $$2.$$ According to the second law of thermodynamics, for the process to be feasible, the entropy changes, $${S_2} - {S_1}$$ of the system

At a place where the surroundings are at $$1$$ bar $${27^ \circ }C,$$ a closed rigid thermally insulated tank contains $$2$$ $$kg$$ air at $$2$$ bar, $${27^ \circ }C,$$ This air is then churned for a while, by a paddle wheel connected to an external motor. If it is given that the irreversibility of the process is $$100$$ $$kJ,$$ find the final temperature and the increase in availability of air. Assume for air $${C_v} = 0.718\,\,kJ/kg\,K$$

Kaplan turbine is

A water turbine delivering $$10$$ $$MW$$ power is to be tested with the help of a geometrically similar $$1:8$$ model, which runs at the same speed as the proto-type.

(a) Find the power developed by the model assuming the efficiencies of the model and the proto-type are equal.

(b) Find the ratio of the heads and the ratio of mass flow rates between the proto-type and the model.