A spring mass damper system (mass m, stiffness k, and damping coefficient c) excited by a force F(t) = B sin ωt, where B, ω and t are the amplitude, frequency and time, respectively, is shown in the figure. Four different responses of the system (marked as (i) to (iv)) are shown just to the right of the system figure. In the figures of the responses, A is the amplitude of response shown in red color and the dashed lines indicate its envelope. The responses represent only the qualitative trend and those are not drawn to any specific scale.

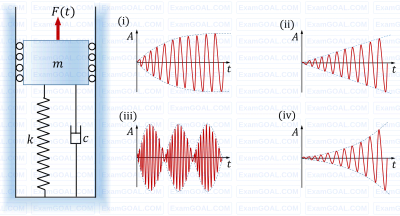
Four different parameter and forcing conditions are mentioned below.

(P) c > 0 and $ω=\sqrt{k/m}$

(Q) c < 0 and ω ≠ 0

(R) c = 0 and $\omega=\sqrt{k/m}$

(S) c = 0 and $\omega \cong\sqrt{k/m}$

Which one of the following options gives correct match (indicated by arrow →) of the parameter and forcing conditions to the responses?

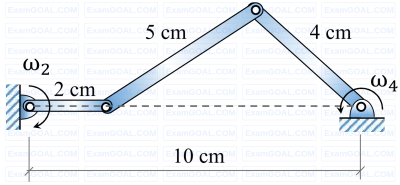
In the configuration of the planar four-bar mechanism at a certain instant as shown in the figure, the angular velocity of the 2 cm long link is ω₂ = 5 rad/s. Given the dimensions as shown, the magnitude of the angular velocity ω₄ of the 4 cm long link is given by rad/s (round off to 2 decimal places).

Which one of the following is an intensive property of a thermodynamic system?

Consider 1 kg of an ideal gas at 1 bar and 300 K contained in a rigid and perfectly insulated container. The specific heat of the gas at constant volume c_v is equal to 750 J-kg^-1K^-1. A stirrer performs 225 kJ of work on the gas. Assume that the container does not participate in the thermodynamic interaction. The final pressure of the gas will be ______ bar (in integer).