All the elements in the circuit are ideal. The power delivered by the 10 V source in watts is

The circuit shown in the figure with the switch S open, is in steady state. After the switch S is closed, the time constant of the circuit in seconds is

For a two-phase network, the phase voltages $V_p$ and $V_q$ are to be expressed in terms of sequence voltages $V_\alpha$ and $V_\beta$ as $\begin{bmatrix} V_p \\ V_q \end{bmatrix} = S \begin{bmatrix} V_\alpha \\ V_\beta \end{bmatrix}$. The possible option(s) for matrix $S$ is/are

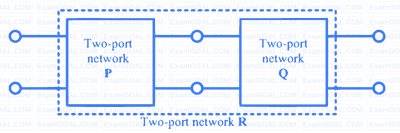
Two passive two-port networks P and Q are connected as shown in the figure. The impedance matrix of network P is $Z_P = \begin{bmatrix} 40 \Omega & 60 \Omega \\ 80 \Omega & 100 \Omega \end{bmatrix}$. The admittance matrix of network Q is $Y_Q = \begin{bmatrix} 5 \, \text{S} & -2.5 \, \text{S} \\ -2.5 \, \text{S} & 1 \, \text{S} \end{bmatrix}$. Let the ABCD matrix of the two-port network R in the figure be $\begin{bmatrix} \alpha & \beta \\ \gamma & \delta \end{bmatrix}$. The value of $\beta$ in $\Omega$ is ______________ (rounded off to 2 decimal places).