Consider the second-order linear ordinary differential equation

$\rm x^2\frac{d^2y}{dx^2}+x\frac{dy}{dx}-y=0, x\ge1$

with the initial conditions 

$\rm y(x=1)=6, \left.\frac{dy}{dx}\right|_{x=1}=2$

The value of 𝑦 at 𝑥 = 2 equals ________.

(Answer in integer)

The initial value problem

$\rm \frac{dy}{dt}+2y=0, y(0)=1$

is solved numerically using the forward Euler’s method with a constant and positive time step of Δt. 

Let 𝑦_𝑛 represent the numerical solution obtained after 𝑛 steps. The condition |𝑦_n+1| ≤ |𝑦_n| is satisfied if and only if Δt does not exceed _____________.

(Answer in integer)

The options show frames consisting of rigid bars connected by pin joints. Which one of the frames is non-rigid? 

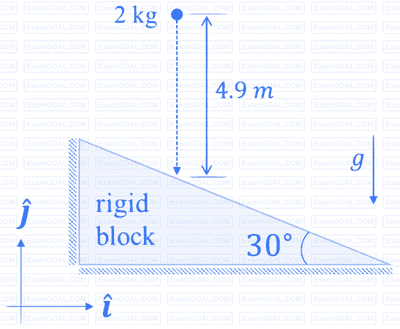
A spherical ball weighing 2 kg is dropped from a height of 4.9 m onto an immovable rigid block as shown in the figure. If the collision is perfectly elastic, what is the momentum vector of the ball (in kg m/s) just after impact?

Take the acceleration due to gravity to be 𝑔 = 9.8 m/s². Options have been rounded off to one decimal place.