Consider the state equation $$\mathop X\limits^ \bullet \left( t \right) = Ax\left( t \right)$$
Given : $${e^{AT}} = \left[ {\matrix{ {{e^{ - t}} + t{e^{ - t}}} & {t{e^{ - t}}} \cr { - t{e^{ - t}}} & {{e^{ - t}} - t{e^{ - t}}} \cr } } \right]$$

(a) Find a set of states $${x_1}\left( 1 \right)$$ and $${x_2}\left( 1 \right)$$ such that $${x_1}\left( 2 \right) = 2.$$
(b) Show that $$\,{\left( {s{\rm I} - A} \right)^{ - t}} = \Phi \left( s \right) = {1 \over \Delta }\left[ {\matrix{ {s + 2} & 1 \cr { - 1} & s \cr } } \right];$$ $$\Delta = {\left( {s + 1} \right)^2}$$
(c) From $$\Phi \left( s \right),$$ find the matrix $$A$$.

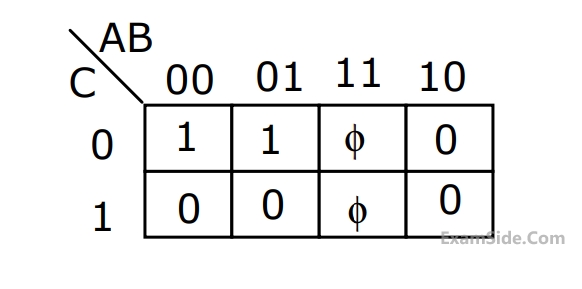
The minimal product-of-sums function described by the $$K$$-map given in Fig.

A dual-slope analog-to-digital converter uses an $$N$$-bit counter. When the input signal $${V_a}$$ is being integrated, the counter is allowed to count up to a value:

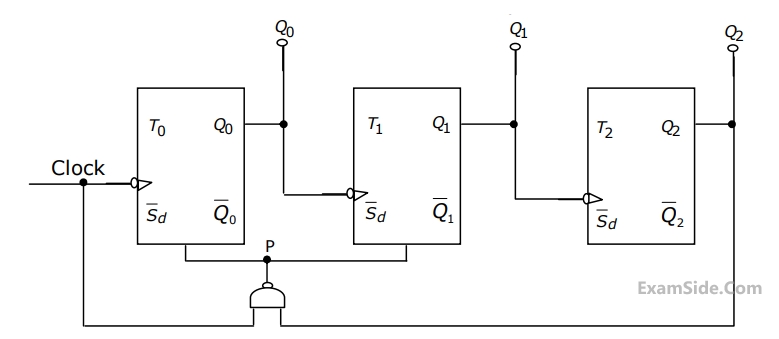
The counter shown in Fig. is initially in state $${Q_2} = 0,\,{Q_1} = 1,\,{Q_0} = 0.$$ With reference to the $$CLK$$ input, draw waveforms for $${Q_2},{Q_1},{Q_0}$$ and $$P$$ for the next three $$CLK$$ cycles.