A company need to develop digital signal processing software for one of its newest inventions. The software is expected to have $$4000$$ lines of code. The company needs to determine the effort in person months needed to develop this software using basic $$COCOMO$$ model. The multiplicative factor for this model is given as $$2.8$$ for the software development on embedded systems. While the exponentiation factor is given as $$1.20.$$ What is the estimated effort in person months?

Which of the following is NOT desired in a good Software Requirement Specifications $$(SRS)$$ document?

A company needs to develop a strategy for Software Product development for which it has a choice of two programming language $${L_1}$$ and $${L_2}$$. The number of lines of code $$(LOC)$$ developed using $${L_2}$$ is estimated to be twice the $$LOC$$ developed with $${L_1}$$ the product will have to be maintained for five years. Various parameters for the company are given in the table below.

Total cost of the project includes cost of development & maintenance. What is the $$LOC$$ for $${L_1}$$ for which the cost of the project using $${L_1}$$ is equal to the cost of the project using $${L_2}$$

The following is comment written for $$a$$ $$c$$ function. This function computes the roots of quadratic equation. $$a{x^2} + bx + c = 0$$ the function stores two real roots in $${}^ * root1\,\,\& \,\,{}^ * root2\,\,\,\& $$ returns the status of validity of roots. In handles four different kinds of cases
$$i)$$ When coefficient $$a$$ is zero or irrespective of discriminate
$$ii)$$ When discriminate is positive.
$$iii)$$ When discriminate is zero
$$iv)$$ When discriminate is negative

Only in cases $$(ii)$$ & $$(iii)$$ the stored roots are valid Otherwise $$0$$ is stored in the roots the function returns $$0$$ when the roots are valid & - $$1$$ otherwise. The function also ensures root $$1$$ $$> =$$ root $$2.$$

int get QuadRoots(float a, float b, float c, float $${}^ * root1$$, float $${}^ * root2$$);

A software test engineer is assigned the job of doing block box testing. He comes up with the following test cases, many of which are redundant

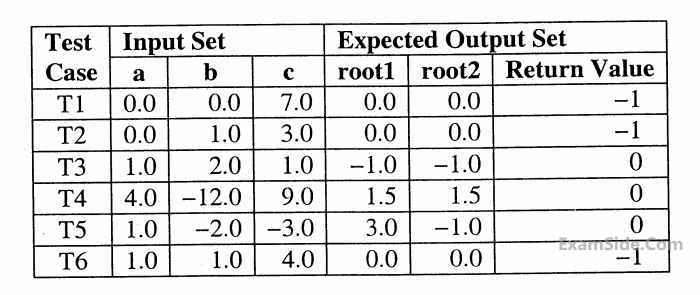
Which one of the following options provide the set of non-redundant tests using equivalence class partitioning approach from input perspective for black box testing?