Application of Derivatives · Mathematics · COMEDK
MCQ (Single Correct Answer)
If $f(x)=x^3+\frac{3}{2} x^2+3 x+3$, then $f(x)$ is
An open hemispherical storage tank has radius 13 m . Oil flows into the tank such that the depth ' $\boldsymbol{h}$ ' of oil in the tank changes at the rate of $3 \mathrm{~m} / \mathrm{hr}$. When the depth $\boldsymbol{h}=1 \mathrm{~m}$, the rate of change of the area of the top surface of the oil is
The function $f(x)=e^{a x}+e^{-a x}, x \in \mathbb{R}$ and $a<0$, is strictly decreasing for all values of ' $x$ ', where
The absolute maximum and minimum values of the function $f(x)=\sin x+\sqrt{3} \cos x$ in $[0, \pi]$ are
A movie screen on a wall is $\mathbf{2 0}$ feet high and $\mathbf{1 0}$ feet above the floor. What is the maximum viewing angle $\boldsymbol{\theta}$ (in radians) that can be achieved by positioning yourself at the optimal distance from the wall?
A square plate is contracting at a uniform rate of $2 \mathrm{~cm}^2 / \mathrm{min}$. The rate at which the perimeter is decreasing when the side of the square is 16 cm is:
If $\mathbf{3 ~ c m} / \mathbf{s}$ is the rate at which the side of an equilateral triangle increases, then the rate of change of area, when the side is $\mathbf{1 2 ~ c m}$ is:
If the function $f(x)=x^4-31 x^2+\boldsymbol{a} x+5$ has a turning point at $x=1$, then the value of ' $\boldsymbol{a}$ ' is $\_\_\_\_$ and the function attains a $\_\_\_\_$ at $x=1$
The behaviour of the function $f(x)=\sin \left(2 x+\frac{\pi}{4}\right)$ on $\left(\frac{3 \pi}{8}, \frac{5 \pi}{8}\right)$ is:
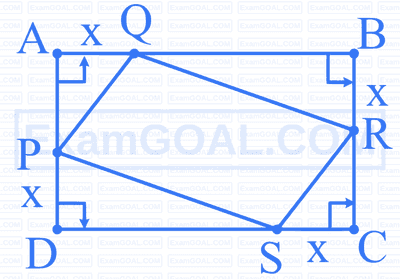
Quadrilateral PQRS is inscribed inside a rectangle of dimensions $10 \mathrm{~cm} \times 8 \mathrm{~cm}$. The value of ' $x$ ', if the area of the quadrilateral is minimum is
$$ \text { The rate of change of the volume of a sphere with respect to its surface area } \mathrm{S} \text { is } $$
The turning point of the function $$y=\frac{a x-b}{(x-1)(x-4)}$$ at the point $$P(2,-1)$$ is
The side of a cube is equal to the diameter of a sphere. If the side and radius increase at the same rate then the ratio of the increase of their surface area is
What is the nature of the function $$f(x)=x^3-3 x^2+4 x$$ on real numbers?
$$ \text { If } f(x)=\frac{a \sin x+b \cos x}{c \sin x+d \cos x} \text { is decreasing for all } x \text {, then } $$
$$ \text { The function } y=\tan x-x \text { is } $$
If $$f(x)=\log x+b x^2+a x, x \neq 0$$ has extreme values (or turning points) at $$x=-1$$ and $$x=2$$ then the values of $$\mathrm{a}$$ and $$\mathrm{b}$$ are
The dimensions of the largest rectangle of side $$x$$ and $$y$$ that can be inscribed in the right angled triangle of sides $$\mathrm{a}$$ and $$\mathrm{b}$$ is
If $$(x-a)^2+(y-b)^2=c^2$$, where $$\mathrm{a}, \mathrm{b}, \mathrm{c}$$ are some constants, $$c>0$$ then $$\frac{\left[1+\left(\frac{d y}{d x}\right)^2\right]^{\frac{3}{2}}}{\frac{d^2 y}{d x^2}}$$ is independent of
The side of an equilateral triangle expands at the rate of $$\sqrt{3} \mathrm{~cm} / \mathrm{sec}$$. When the side is $$12 \mathrm{~cm}$$, the rate of increase of its area is
If $$f(x)=2 x^3+9 x^2+\lambda x+20$$ is a decreasing function of $$x$$ in the largest possible interval $$(-2,-1)$$, then $$\lambda$$ is equal to
$$ \text { The point on the curve } x^2=x y \text { which is closest to }(0,5) \text { is } $$
For a given curve $$y=2 x-x^2$$, when $$x$$ increases at the rate of 3 units/sec, then how does the slope of the curve change?
The most economical proportion of the height of a covered box of fixed volume whose base is a rectangle with one side three times as long as the other, is
The slope of the tangent to the curve, $$y=x^2-x y$$ at $$\left(1, \frac{1}{2}\right)$$ is
Let $$f(x)=a+(x-4)^{\frac{4}{9}}$$, then minima of $$f(x)$$ is
The function $$f(x)=\frac{x}{2}+\frac{2}{x}$$ has a local minimum at
$$ f(x)=2 x-\tan ^{-1} x-\log (x+\sqrt{x^2+1}) \text { is monotonically increasing, when } $$
The altitude of a cone is $$20 \mathrm{~cm}$$ and its semi vertical angle is $$30^{\circ}$$. If the semi vertical angle is increasing at the rate of $$2^0$$ per second, then the radius of the base is increasing at the rate of
If the volume of a sphere is increasing at a constant rate, then the rate at which its radius is increasing is
If the tangent to the curve $$xy + ax + by = 0$$ at (1, 1) is inclined at an angle $${\tan ^{ - 1}}2$$ with X-axis, then
Let $$f(x) = a - {(x - 3)^{8/9}}$$, then maxima of $$f(x)$$ is
The approximate value of $$f(5.001)$$, where $$f(x)=x^3-7x^2+15$$ is
Find the maximum value of $$f(x) = {1 \over {4{x^2} + 2x + 1}}$$.
The point on the curve $$y^2=x$$, the tangent at which makes an angle 45$$^\circ$$ with X-axis is
The length of the subtangent to the curve $${x^2}{y^2} = {a^4}$$ at $$( - a,a)$$ is
The range in which $$y = - {x^2} + 6x - 3$$ is increasing, is
OA and OB are two roads enclosing an angle of 120$$^\circ$$. X and Y start from O at the same time. X travels along OA with a speed of 4 km/h and Y travels along OB with a speed of 3 km/h. The rate at which the shortest distance between X and Y is increasing after 1 hour is
