MHT CET 2023 13th May Morning Shift | MHT CET Year Wise Previous Years Questions

MHT CET 2023 13th May Morning Shift

MCQ (Single Correct Answer)

-0

A line is drawn through the point $$(1,2)$$ to meet the co-ordinate axes at $$\mathrm{P}$$ and $$\mathrm{Q}$$ such that it forms a $$\triangle \mathrm{OPQ}$$, where $$\mathrm{O}$$ is the origin. If the area of $$\triangle \mathrm{OPQ}$$ is least, then the slope of the line $$\mathrm{PQ}$$ is

$$-2$$

$$\frac{-1}{2}$$

$$\frac{1}{2}$$

MHT CET 2023 13th May Morning Shift

MCQ (Single Correct Answer)

-0

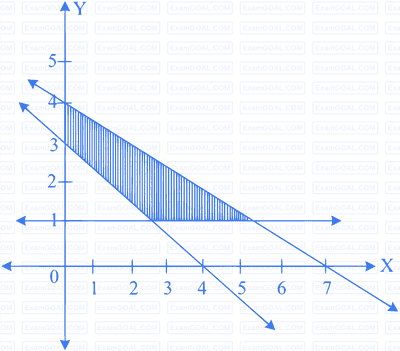
If feasible region is as shown in the figure, then related inequalities are

MHT CET 2023 13th May Morning Shift Mathematics - Linear Programming Question 33 English

$$3 x+4 y \geq 12,4 x+7 y \leq 28, y \leq 1, x \geq 0, y \geq 0$$

$$3 x+4 y \geq 12,4 x+7 y \leq 28, y \geq 1, x \geq 0, y \geq 0$$

$$3 x+4 y \leq 12,4 x+7 y \leq 28, y \leq 1, x \geq 0, y \geq 0$$

$$3 x+4 y \leq 12,4 x+7 y \geq 28, y \geq 1, x \geq 0, y \geq 0$$

MHT CET 2023 13th May Morning Shift

MCQ (Single Correct Answer)

-0

The general solution of the equation $$3 \sec ^2 \theta=2 \operatorname{cosec} \theta$$ is

$$\mathrm{n} \pi+\frac{\pi}{4}, \mathrm{n} \in \mathrm{Z}$$

$$2 \mathrm{n} \pi+(-1)^{\mathrm{n}} \frac{\pi}{12}, \mathrm{n} \in \mathrm{Z}$$

$$\mathrm{n} \pi+(-1)^{\mathrm{n}} \frac{\pi}{6}, \mathrm{n} \in \mathrm{Z}$$

$$\mathrm{n} \pi+(-1)^{\mathrm{n}} \frac{\pi}{3}, \mathrm{n} \in \mathrm{Z}$$

MHT CET 2023 13th May Morning Shift

MCQ (Single Correct Answer)

-0

A right circular cone has height $$9 \mathrm{~cm}$$ and radius of base $$5 \mathrm{~cm}$$. It is inverted and water is poured into it. If at any instant, the water level rises at the rate $$\frac{\pi}{\mathrm{A}} \mathrm{cm} / \mathrm{sec}$$. where $$\mathrm{A}$$ is area of the water surface at that instant, then cone is completely filled in

70 sec.

75 sec.

72 sec.

77 sec.

PREVIOUS NEXT