Two plates are connected by fillet welds of size 10 mm and subjected to tension, as shown in the figure. The thickness of each plate is 12 mm. The yield stress and the ultimate stress of steel under tension are 250 MPa and 410 MPa, respectively. The welding is done in the workshop (partial safety factor, 𝛾_𝑚𝑤 = 1.25). As per the Limit State Method of IS 800: 2007, what is the minimum length (in mm, rounded off to the nearest higher multiple of 5 mm) required of each weld to transmit a factored force P equal to 275 kN? 

A circular solid shaft of span L = 5 m is fixed at one end and free at the other end. A torque T = 100 kN.m is applied at the free end. The shear modulus and polar moment of inertia of the section are denoted as G and J, respectively. The torsional rigidity GJ is 50,000 kN.m² /rad. The following are reported for this shaft:

Statement i) The rotation at the free end is 0.01 rad

Statement ii) The torsional strain energy is 1.0 kN.m

With reference to the above statements, which of the following is true?

When a simply-supported elastic beam of span L and flexural rigidity EI (E is the modulus of elasticity and I is the moment of inertia of the section) is loaded with a uniformly distributed load w per unit length, the deflection at the mid-span is

$\rm \Delta_0=\frac{5}{384}\frac{wL^4}{El}$

If the load on one half of the span is now removed, the mid-span deflection _______.

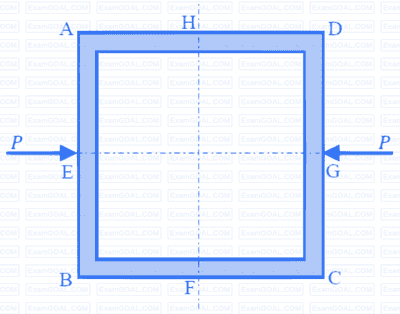
In the frame shown in the figure (not to scale), all four members (AB, BC, CD, and AD) have the same length and same constant flexural rigidity. All the joints A, B, C, and D are rigid joints. The midpoints of AB, BC, CD, and AD, are denoted by E, F, G, and H, respectively. The frame is in unstable equilibrium under the shown forces of magnitude 𝑃 acting at E and G. Which of the following statements is/are TRUE?