For a given matrix $$A = \left[ {\matrix{ 2 & { - 2} & 3 \cr { - 2} & { - 1} & 6 \cr 1 & 2 & 0 \cr } } \right],$$ one of the eigen value is $$3.$$ The other two eigen values are

Using Cauchy's Integral Theorem, the value of the integral (integration being taken in counter clockwise direction)

$$\int\limits_C {{{{z^3} - 6} \over {3z - i}}} dz$$ is where C is |z| = 1

The solution of the differential equation $$\,{x^2}{{dy} \over {dx}} + 2xy - x + 1 = 0\,\,\,$$ given that at $$x=1,$$ $$y=0$$ is

The directional derivative of $$\,\,f\left( {x,y,z} \right) = 2{x^2} + 3{y^2} + {z^2}\,\,$$ at the point $$P(2,1,3)$$ in the direction of the vector $${\mkern 1mu} \vec a = \widehat i - 2\widehat k{\mkern 1mu} $$ is _________.

Solution for the system defined by the set of equations $$4y+3z=8, 2x-z=2$$ & $$3x+2y=5$$ is

As per $$IS:$$ $$456$$-$$2000,$$ consider the following statements:

$$1.$$ The modular ratio considered in the working stress method depends on the type of steel used.
$$2.$$ There is an upper limit on the nominal shear stress in beams (even with shear reinforcement) due to the possibility of crushing of concrete in diagonal compression.
$$3.$$ A rectangular slab whose length is equal to its width may not be a two-way slab for some support conditions.

The TRUE statements are

Assuming concrete below the neutral axis to be cracked, the shear stress across the depth of a singly-reinforced rectangular beam section

In the design of beams for the limit state of collapse in flexure as per $$IS:$$ $$456$$ - $$2000,$$ let the maximum strain in concrete be limited to $$0.0025$$ (in place of $$0.0035$$). For this situation, consider a rectangular beam section with breadth as $$250$$ $$mm,$$ effective depth as $$350$$ $$mm,$$ area of tension steel as $$1500\,\,m{m^2},$$ and characteristic strengths of concrete and steel as $$30$$ $$MPa$$ and $$250$$ $$MPa$$ respectively.

At the limiting state of collapse in flexure, the force acting on the compression zone of the section is

In the design of beams for the limit state of collapse in flexure as per $$IS:$$ $$456$$ - $$2000,$$ let the maximum strain in concrete be limited to $$0.0025$$ (in place of $$0.0035$$). For this situation, consider a rectangular beam section with breadth as $$250$$ $$mm,$$ effective depth as $$350$$ $$mm,$$ area of tension steel as $$1500\,\,m{m^2},$$ and characteristic strengths of concrete and steel as $$30$$ $$MPa$$ and $$250$$ $$MPa$$ respectively.

The depth of neutral axis for the balanced failure is

Consider the following statements:

$$1.\,\,\,\,\,\,$$ The width-to-thickness ratio limitations on the plate elements under
$$\,\,\,\,\,\,\,\,\,\,\,$$ compression in steel members are imposed by $$IS:$$ $$800$$ - $$1984$$ in order to avoid
$$\,\,\,\,\,\,\,\,\,\,\,$$ fabrication difficulties.
$$2.\,\,\,\,\,\,$$ In a doubly reinforced concrete beam, the strain in compressive reinforcement is
$$\,\,\,\,\,\,\,\,\,\,\,$$ higher than the strain in the adjoining concrete.
$$3.\,\,\,\,\,\,$$ If a cantilever $${\rm I}$$-section supports slab construction all along its length with
$$\,\,\,\,\,\,\,\,\,\,\,$$ sufficient friction between them, the permissible bending stress in compression
$$\,\,\,\,\,\,\,\,\,\,\,$$ will be the same as that in tension.

The TRUE statements are

Consider the following statements

$${\rm I}.\,\,\,\,$$ Effective length of a battened column is usually increased to account for the additional load on battens due to the lateral expansion of columns.
$${\rm II}.\,\,\,\,$$ As per $$IS:$$ $$800$$-$$1984,$$ permissible stress in bending compression depends on both Euler buckling stress and the yield stress of steel.
$${\rm III}.\,\,\,\,$$ As per $$IS:$$ $$800$$-$$1984,$$ the effective length of a column effectively held in position at both ends but not restrained against rotation, is taken to be greater than that in the ideal end conditions.

The TRUE statements are

In the design of welded tension members, consider the following statements:

$${\rm I}.\,\,\,\,\,\,\,\,\,$$ The entire cross-sectional area of the connected leg is assumed to contribute to the effective area in case of angles.
$${\rm II}.\,\,\,\,\,\,\,\,\,$$ Two angles back-to-back and tack-welded as per code requirements may be assumed to be behave as a $$T$$-section
$${\rm III}.\,\,\,\,\,\,\,\,\,$$ A check on slenderness ratio may be necessary in some cases.

The TRUE statements are

The buckling load $$P = {P_{cr}}$$ for the column $$AB$$ in figure, as $${K_T}$$ approaches infinity, becomes $$\alpha {{{\pi ^2}E{\rm I}} \over {{L^2}}}$$

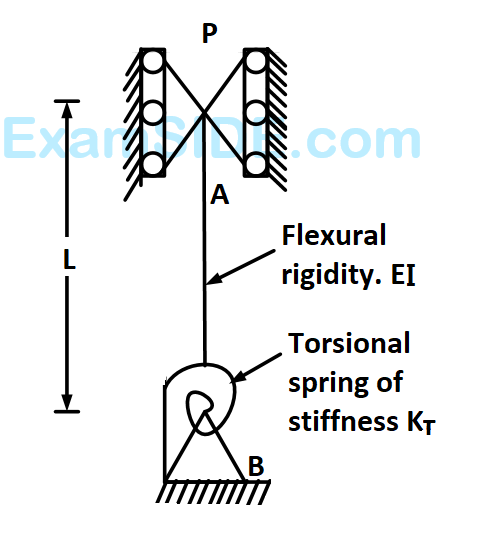
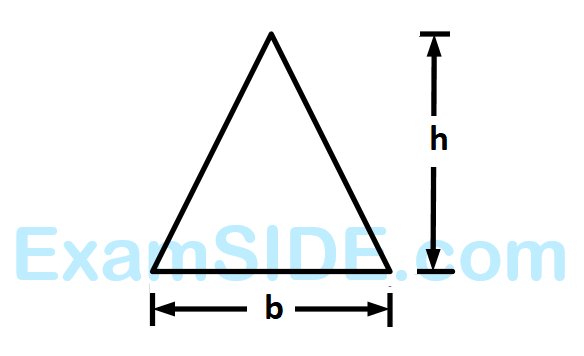
Where $$\alpha $$ is equal to

Consider a propped cantilever $$ABC$$ under two loads of magnitude $$P$$ each as shown in figure below. Flexural rigidity of the beam is $$EI$$.

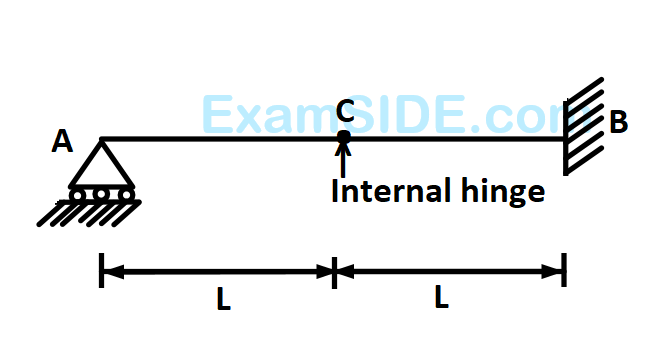
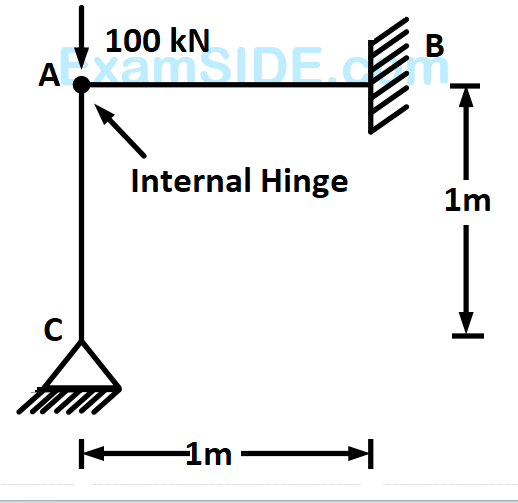
The reaction at $$C$$ is

Consider a propped cantilever $$ABC$$ under two loads of magnitude $$P$$ each as shown in figure below. Flexural rigidity of the beam is $$EI$$.

The rotation at $$B$$ is

A thin-walled long cylindrical tank of inside radius $$'r'$$ is subjected simultaneously to internal gas pressure $$P$$ and axial compressive force $$F$$ at its ends. In order to produce 'pure shear' state of stress in the wall of the cylinder, $$F$$ should be equal to

Consider the beam $$AB$$ shown in the figure below. Part $$AC$$ of the beam is rigid while Part $$CB$$ has the flexural rigidity $$EI$$. Identify the correct combination of deflection at end $$B$$ and bending moment at end $$A,$$ respectively.

A beam with cross-section given below is subjected to a positive bending moment (causing compression at the top) of $$16$$ $$kN$$-$$m$$ acting around the horizontal axis. The tensile force acting on the hatched area of the cross-section is

For the section shown below, second moment of the area about an axis $$d/4$$ distance above the bottom of the area is

$${\rm I}$$-section of a beam is formed by gluing wooden planks as shown in the figure below. If this beam transmits a constant vertical shear force of $$3000$$ $$N$$, the glue at any of the four joints will be subjected to a shear force (in $$kN$$ per meter length ) of

A long shaft of diameter $$d$$ is subjected to twisting moment $$T$$ at its ends. The maximum normal stress acting at its cross-section is equal to

A simply supported beam $$AB$$ has the bending moment diagram as shown in the following figure :

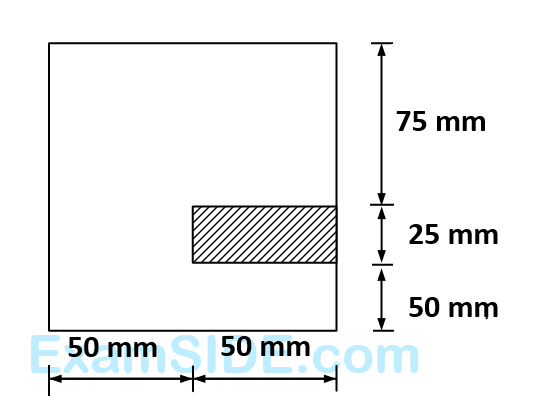
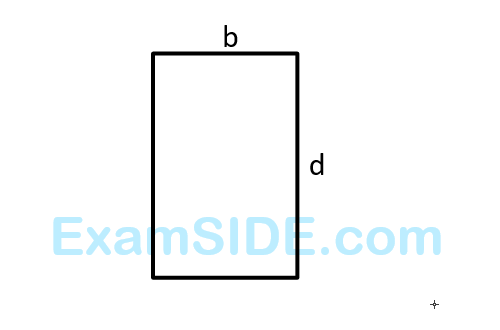
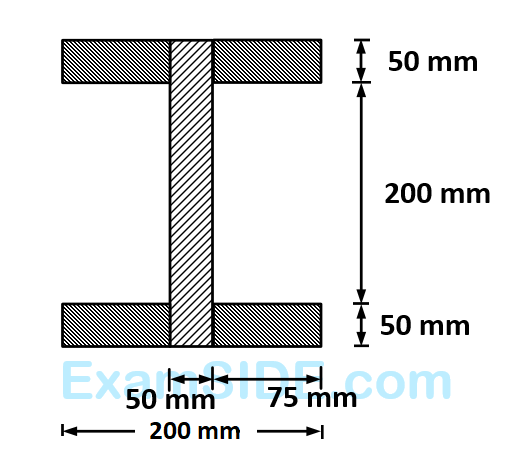
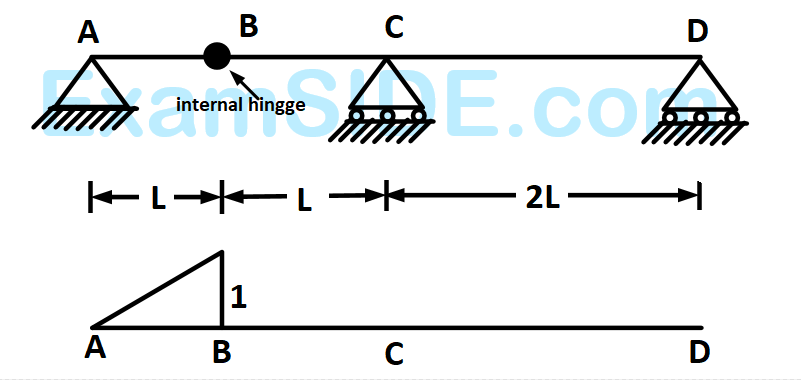
The beam is possibly under the action of following loads

Mohr's cicle for the state of stress defined by $$\left[ {\matrix{ {30} & 0 \cr 0 & {30} \cr } } \right]$$ $$MPa$$ is a circle with

When the triangular section of a beam as shown below becomes a plastic hinge, the compressive force acting on the section (with $${\sigma _y}$$ denoting the yield stress) becomes

Carry-over factor $${C_{AB}}$$ for the beam shown in the figure below is

Vertical reaction developed at $$B$$ in the frame below due to the applied load of $$100$$ $$kN$$ (with $$150,000$$ $$m{m^2}$$ cross- sectional area and $$3.125 \times {10^9}$$ $$m{m^4}$$ moment of inertia for both members) is

Consider the beam $$ABCD$$ and the influence line as shown below. The influence line pertains to