A square loop of side length $a$ is moving away from an infinitely long current carrying conductor at a constant speed $v$ as shown. Let $x$ be the instantaneous distance between the long conductor and side $A B$. The mutual inductance $M$ of the square loop-long conductor pair changes with time $t$ according to which of the following graphs?

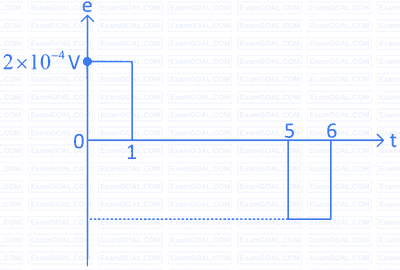
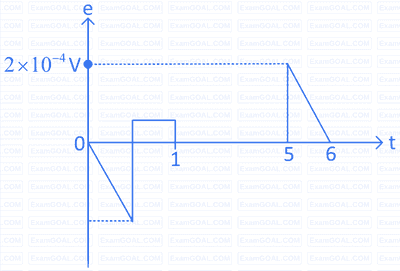
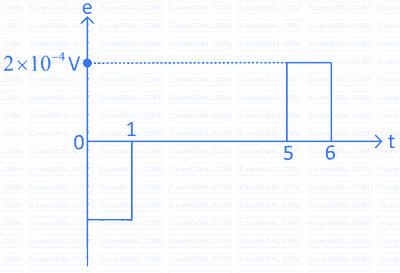
A square loop of side $$2 \mathrm{~cm}$$ enters a magnetic field with a constant speed of $$2 \mathrm{~cm} \mathrm{~s}^{-1}$$ as shown. The front edge enters the field at $$t=0 \mathrm{~s}$$. Which of the following graph correctly depicts the induced emf in the loop?
(Take clockwise direction positive)

The current in a coil changes from $$2 \mathrm{~A}$$ to $$5 \mathrm{~A}$$ in $$0.3 \mathrm{~s}$$. The magnitude of emf induced in the coil is $$1.0 \mathrm{~V}$$. The value of self-inductance of the coil is
A metallic rod of length $$1 \mathrm{~m}$$ held along east-west direction is allowed to fall down freely. Given horizontal component of earth's magnetic field $$B_H=3 \times 10^{-5} \mathrm{~T}$$. the emf induced in the rod at an instant $$t=2 \mathrm{~s}$$ after it is released is
(Take, $$g=10 \mathrm{~ms}^{-2}$$ )