TG EAPCET 2024 (Online) 10th May Morning Shift

Paper was held on Fri, May 10, 2024 3:30 AM

Chemistry

The wavenumber of first spectral line of Lyman series of $\mathrm{He}^{+}$ion is $x \mathrm{~m}^{-1}$. What is the wave number ( $\mathrm{in}^{-1}$ ) of second spectral line of Balmer series of $\mathrm{Li}^{2+}$ ion?

The uncertainty in determination of position of a small ball of mass 10 g is $10^{-33} \mathrm{~m}$. With what $\%$ of accuracy its speed can be measured, if it has a speed of 52.5 $\mathrm{ms}^{-1} ?\left(h=6.6 \times 10^{-34} \mathrm{Js}\right)$

In which of the following ionic pairs, second ion is smaller in size than the first ion?

The set of elements which obey the general electronic configuration $(n-1) d^{1-10} n s^2$ is?

Identify the set of molecules which are not in the correct order of their dipole moments

Match the following.

List I (Molecule)	List II (Shape)
A $\mathrm{SF}_4$	I. T-shaped
B $\mathrm{CIF}_3$	II. Square planar
C $\mathrm{BrF}_5$	III. See-saw
D $\mathrm{XeF}_4$	IV. Square pyramidal

The correct answer is

At 400 K , an ideal gas is enclosed in a $0.5 \mathrm{~m}^3$ vessel at pressure of 203 kPa . What is the change in temperature required (in K ), if it occupies a volume of $0.2 \mathrm{~m}^3$ under a pressure of 304 kPa ? (Nearest interger)

Match the following.

List.l (Substance)	List II (Equivalent weight)
A $\mathrm{Na}_2 \mathrm{CO}_3$	I. $\frac{M}{5}$
B $\mathrm{KMnO}_4 / \mathrm{H}^{+}$	II. $\frac{M}{3}$
C $\mathrm{K}_2 \mathrm{Cr}_2 \mathrm{O}_7 / \mathrm{H}^{+}$	III. $\frac{M}{2}$
D $\mathrm{KMnO}_4 / \mathrm{H}_2 \mathrm{O}$	IV. $\frac{M}{6}$

( $M=$ Formula weight)

The correct answer is

The standard enthalpy of combustion of C (graphite). $\mathrm{H}_2(g)$ and $\mathrm{CH}_3 \mathrm{OH}(l)$ respectively are $-393,-286$ and $-726 \mathrm{~kJ} \mathrm{~mol}^{-1}$. What is the standard enthalpy of formation of methanol?

Observe the following species.

(i) $\mathrm{NH}_3$

(ii) $\mathrm{AlCl}_3$

(iii) $\mathrm{SnCl}_4$

(iv) $\mathrm{CO}_2$

(v) $\mathrm{Ag}^{+}$

(vi) $ \mathrm{HSO}_{4}^{-} $

How many of the above species act as Lewis acids?

The normality of 20 volume solution of hydrogen peroxide is

Consider the following reactions.

$$ \begin{array}{r} \mathrm{Cs}+\mathrm{O}_2 \text { (excess) } \rightarrow X \\ \mathrm{Na}+\mathrm{O}_2 \rightarrow Y \end{array} $$

Identify the correct Statement about $X$ and $Y$

Choose the correct statement from the following.

I. In vapour phase $\mathrm{BeCl}_2$ exists as chlorobridge dimer.

II. $\mathrm{BeSO}_4$ is readily soluble in water.

III. BeO is completely basic in nature.

IV. $\mathrm{BeCO}_3$ being unstable, is kept in the atmosphere of $\mathrm{CO}_2$.

V. $\mathrm{BeCO}_3$ is less soluble among all the carbonates of group 2 elements.

Observe the following reactions (not balanced)

$$ \begin{aligned} & \mathrm{BF}_3+\mathrm{LiAlH}_4 \xrightarrow{\left(\mathrm{C}_2 \mathrm{H}_5\right)_2 \mathrm{O}} X+\mathrm{LiF}+\mathrm{AlF}_3{ }^{-} \\ & X+\mathrm{NaH} \longrightarrow Y \end{aligned} $$

The incorrect statement about $Y$ is

$\mathbf{Assertion (A)}$ Silicones are used for water proofing of fabrics.

$\mathbf{Reason (R)}$ The repeating unit in silicones is

The correct answer is

Acrolein $(X)$ is one of the chemicals formed when $\mathrm{O}_3$ and $\mathrm{NO}_2$ react with unburnt hydrocarbons present in the polluted air. The structure of ' $X$ ' is

An organic compound containing phosphorous on oxidation with $\mathrm{Na}_2 \mathrm{O}_2$ gives a compound ' $X$ '. This ' $X$ ' when boiled with $\mathrm{HNO}_3$ followed by treatment with a reagent gives yellow precipitate $Y . X$ and $Y$ respectively are

The correct IUPAC name of the structure given below is

The major product ' $Y^{\prime}$ in the given sequence of reactions is

$$ \mathrm{C}_3 \mathrm{H}_7 \mathrm{OH} \xrightarrow[443 \mathrm{~K}]{\text { Conc. } \mathrm{H}_2 \mathrm{SO}_4} X \xrightarrow[\left(\mathrm{C}_6 \mathrm{H}_5 \mathrm{CO}\right)_2 \mathrm{O}_2]{\mathrm{HBr}} Y $$

Compound ' $A$ ' on heating with sodalime gives propane. Identify the compound ' $A$ '.

An element with molar mass $2.7 \times 10^{-2} \mathrm{~kg} \mathrm{~mol}^{-1}$ forms a cubic unit cell with edge length of 405 pm . If its density is $2.7 \times 10^3 \mathrm{~kg} \mathrm{~m}^{-3}$, the number of atoms present in one unit cell of it is (Given; $N_A=6.023 \times 10^{23} \mathrm{~mol}^{-1}$ )

At $300 \mathrm{~K}, 0.06 \mathrm{~kg}$ of an organic solute is dissolved in 1 kg water. The vapour pressure of solution at 300 K is 3.768 kPa . If vapour pressure of water at that temperature is 3.78 kPa , what is the molar mass of the organic solute (in $\mathrm{g} \mathrm{mol}^{-1}$ )? Assume the solution is dilute

The molar conductivity of 0.02 M solution of an electrolyte is $124 \times 10^{-4} \mathrm{~S} \mathrm{~m}^2 \mathrm{~mol}^{-1}$. What is the resistance of same solution (in ohms), kept in a cell of cell constant $129 \mathrm{~m}^{-1}$ ?

The decomposition of benzene diazonium chloride is a first order reaction. The time taken for its decomposition to $\frac{1}{4}$ and $\frac{1}{10}$ of its initial concentration are $t_{\frac{1}{4}}$ and $t_{\frac{1}{4}}^{10}$ respectively. The value of $\frac{t_{\frac{1}{4}}^4}{t_1} \times 100$ is (Give: $\log 2=0.3$ )

10 mL of 0.5 M NaCl is required to coagulate 1 L of $\mathrm{Sb}_2 \mathrm{~S}_3 \mathrm{sol}$ in 2 hours time. The flocculating value of NaCl (in millimoles) is

Kaolinite is a silicate mineral of metal ' $X$ ' and calamine is a carbonate mineral of metal ' $Y^{\prime}, X$ and $Y$ respectively are

$\mathrm{NH}_2 \mathrm{CONH}_2+2 \mathrm{H}_2 \mathrm{O} \rightarrow[\mathrm{X}] \rightleftharpoons 2 \mathrm{NH}_3+\mathrm{H}_2 \mathrm{O}+[\mathrm{Y}]$

The hybridisation of carbon in $X$ and $Y$ respectively are

Among the hydrides $\mathrm{NH}_3, \mathrm{PH}_3$ and $\mathrm{BiH}_3$, the hydride with highest boiling point is $X$ and the hydride with lowest boiling point is $Y$. What are $X$ and $Y$ respectively?

Xenon (VI) fluoride on complete hydrolysis gives an oxide of xenon ' $O$ '. The total number of $\sigma$ and $\pi$-bonds $\sin$ ' $O$ ' is i"

In which of the following ions the spin only magnetic moment is lowest?

Identify the complex ion with electronic configuration $t_{2 g}^3 e_g^2$.

Identify the structure of the polymer 'P' formed in the given reaction

Caprolactam $\xrightarrow[\mathrm{H}_2 \mathrm{O}]{533-543 \mathrm{~K}} P$

Which of the following vitamin is also called pyridoxine?

The number of -OH groups present in the structures of bithionol, terpineol and chloroxylenol is respectively

Conversion of $X$ to $Y$ in the above reaction is

$$ \mathrm{CH}_3 \mathrm{CH}_2 \mathrm{OH} \xrightarrow[443 \mathrm{~K}]{\text { Conc. } \mathrm{H}_2 \mathrm{SO}_4} X \xrightarrow[\text { (ii) } \mathrm{Zn} / \mathrm{H}_2 \mathrm{O}\,(2 Moless)]{\text { (i) } \mathrm{O}_3} Y \xrightarrow[\text { (i) } \mathrm{H}^{+}]{\text {(i) Conc. } \mathrm{NaOH}} \mathrm{Z} $$

$Z$ is a mixture of alcohol and acid. Reaction of conversion of $Y$ to $Z$ is

Arrange the following in the increasing order of pKa values

What is ' $C$ ' in the following reaction sequence?

Identify the products $R$ and $S$ in the reaction sequence given

$\left(\mathrm{CH}_3\right)_3 \mathrm{COH} \xrightarrow{\mathrm{Na}} P \xrightarrow{\mathrm{CH}_3 \mathrm{Br}} Q \xrightarrow[\Delta]{\mathrm{HI}} R+S$

In the given reaction sequence sequence, $Z$ is

Mathematics

If the real valued function $f(x)=\sin ^{-1}\left(x^2-1\right)-3 \log _3\left(3^x-2\right)$ is not defined for all $x \in(-\infty, a) \cup(b, \infty)$, then $3^a+b^2=$

If $f$ is a real valued function from $A$ onto $B$ defined by $f(x)=\frac{1}{\sqrt{|x-|x||}}$, then $A \cap B=$

Among the following four statements, the statement which is not true, for all $n \in N$ is

If $A=\left[\begin{array}{lll}x & y & y \\ y & x & y \\ y & y & x\end{array}\right]$ is a matrix such that $5 A^{-1}=\left[\begin{array}{ccc}-3 & 2 & 2 \\ 2 & -3 & 2 \\ 2 & 2 & -3\end{array}\right]$, then $A^2-4 A=$

If $A=\left[\begin{array}{lll}9 & 3 & 0 \\ 1 & 5 & 8 \\ 7 & 6 & 2\end{array}\right]$ and $A A^T-A^2=\left[\begin{array}{lll}a_{11} & a_{12} & a_{13} \\ a_{21} & a_{22} & a_{23} \\ a_{31} & a_{32} & a_{33}\end{array}\right]$, then $\sum\limits_{\substack{1 \leq i \leq 3 \\ 1 \leq j \leq 3}} a_{i j}=$

If $a \neq b \neq c, \Delta_1=\left[\begin{array}{lll}1 & a^2 & b c \\ 1 & b^2 & c a \\ 1 & c^2 & a b\end{array}\right]$, $\Delta_2=\left[\begin{array}{ccc}1 & 1 & 1 \\ a^2 & b^2 & c^2 \\ a^3 & b^3 & c^3\end{array}\right]$ and $\frac{\Delta_1}{\Delta_2}=\frac{6}{11}$, then $11(a+b+c)=$

The system of equations $x+3 y+7=0$, $3 x+10 y-3 z+18=0$ and $3 y-9 z+2=0$ has

If $x$ and $y$ are two positive real numbers such that $x+i y=\frac{13 \sqrt{-5+12 i}}{(2-3 i)(3+2 i)}$, then $13 y-26 x=$

If $z=x+i y$ and if the point $P$ represents $z$ in the argand plane, then the locus of $z$ satisfying the equation $|z-1|+|z+i|=2$ is

One of the values of $(-64 i)^{5 / 6}$ is

If $\alpha, \beta$ are the roots of the equation $x+\frac{4}{x}=2 \sqrt{3}$, then $\frac{2}{\sqrt{3}}\left|\alpha^{2024}-\beta^{2024}\right|=$

$\alpha, \beta$ are the real roots of the equation $12 x^{\frac{1}{3}}-25 x^{\frac{1}{6}}+12=0$. If $\alpha>\beta$, then $6 \sqrt{\frac{\alpha}{\beta}}=$

If the expression $7+6 x-3 x^2$ attains its extreme value $\beta$ at $x=\alpha$, then the sum of the squares of the roots of the equation $x^2+\alpha x-\beta=0$ is

$\alpha, \beta$ and $\gamma$ are the roots of the equation $x^3+3 x^2-10 x-24=0$. If $\alpha>\beta>\gamma$ and $\alpha^3+3 \beta^2-10 \gamma-24=11 k$, then $k=$

$\alpha, \beta$ and $\gamma$ are the roots of the equation $8 x^3-42 x^2+63 x-27=0$. If $\beta<\gamma<\alpha$ and $\beta, \gamma$ and $\alpha$ are in geometric progression, then the extreme value of the expression $\gamma x^2+4 \beta x+\alpha$ is

All the letters of word 'COLLEGE' are arranged in all possible ways and all the seven letter words (with or without meaning) thus formed are arranged in the dictionary order. Then, the rank of the word 'COLLEGE' is

If all the possible 3-digit numbers are formed using the digits $1,3,5,7$ and 9 without repeating any digit, then the number of such 3 -digit numbers which are divisible by 3 is

A question paper has 3 parts $A, B$ and $C$. Part $A$ contains 7 questions, part $B$ contains 5 questions and Part Ccontains 3 questions. If a candidate is allowed to answer not more than 4 questions from part $A$; not more than 3 questions from part $B$ and not more than 2 questions from part $C$, then the number of ways in which a candidate can answer exactly 7 questions is

If $p$ and $q$ are the real numbers such that the 7 th term in the expansion of $\left(\frac{5}{p^3}-\frac{3 q}{7}\right)^8$ is 700 , then $49 p^2=$

If $T_4$ represents the 4 th term in the expansion of $\left(5 x+\frac{7}{x}\right)^{\frac{-3}{2}}$ and $x \notin\left[-\sqrt{\frac{7}{5}}, \sqrt{\frac{7}{5}}\right]$, then $\left(x^7 \sqrt{5 x}\right) T_4=$

If $\frac{2 x^3+1}{2 x^2-x-6}=a x+b+\frac{A}{P x-2}+\frac{B}{2 x+q}$, then 51 apB $=$

$\tan A=\frac{-60}{11}$ and $A$ does not lie in the 4th quadrant. $\sec B=\frac{41}{9}$ and $B$ does not lie in the 1st quadrant. If $\operatorname{cosec} A+\cot B=K$, then $24 K=$

If $\tan A+\tan B+\cot A+\cot B=\tan A \tan B-\cot A \cot B$ and $0^{\circ} < A+B<270^{\circ}$, then $A+B=$

If $\cos ^2 84^{\circ}+\sin ^2 126^{\circ}-\sin 84^{\circ} \cos 126^{\circ}=K$ and $\cot A+\tan A=2 K$, then the possible values of $\tan A$ are

The equation that is satisfied by the general solution of the equation $4-3 \cos ^2 \theta=5 \sin \theta \cos \theta$ is

If $\sin ^{-1}(4 x)-\cos ^{-1}(3 x)=\frac{\pi}{6}$, then $x=$

If $\sin h^{-1}(-\sqrt{3})+\cos ^{-1}(2)=K$, then $\cosh K=$

In triangle $A B C$, if $a=4, b=3$ and $c=2$, then $2(a-b \cos C)(a-c \sec B)=$

In $\triangle A B C$, if $A=45^{\circ}, C=75^{\circ}$ and $R=\sqrt{2}$, than $r=$

$P$ and $Q$ are the points of trisection of the segment $A B$. If $2 \hat{\mathbf{i}}-5 \hat{\mathbf{j}}+3 \hat{\mathbf{k}}$ and $4 \hat{\mathbf{i}}+\hat{\mathbf{j}}-6 \hat{\mathbf{k}}$ are the position vectors of $A$ and $B$ respectively, then the position vector of the point which divides $P Q$ in the ratio $2: 3$ is

The position vector of the point of intersection of the line joining the points $\hat{\mathbf{i}}-\hat{\mathbf{j}}+\hat{\mathbf{k}}, \hat{\mathbf{i}}+\hat{\mathbf{j}}-2 \hat{\mathbf{k}}$ and the line joining the points $2 \hat{\mathbf{i}}+\hat{\mathbf{j}}-6 \hat{\mathbf{k}}, 3 \hat{\mathbf{i}}-\hat{\mathbf{j}}-7 \hat{\mathbf{k}}$ is

If $\mathbf{a}=4 \hat{\mathbf{i}}+5 \hat{\mathbf{j}}-3 \hat{\mathbf{k}}$ and $\mathbf{b}=6 \hat{\mathbf{i}}-2 \hat{\mathbf{j}}-2 \hat{\mathbf{k}}$ are two vectors, then the magnitude of the component of $\mathbf{b}$ parallel to $\mathbf{a}$ is

A plane $\pi_1$ passing through the point $3 \hat{\mathbf{i}}-7 \hat{\mathbf{j}}+5 \hat{\mathbf{k}}$ is perpendicular to the vector $\hat{\mathbf{i}}+2 \hat{\mathbf{j}}-2 \hat{\mathbf{k}}$ and another plane $\pi_2$ passing through the point $2 \hat{\mathbf{i}}+7 \hat{\mathbf{k}}-8 \hat{\mathbf{k}}$ is perpendicular to the vector $3 \hat{\mathbf{i}}+2 \hat{\mathbf{j}}+6 \hat{\mathbf{k}}$. If $p_1$ and $p_2$ are the perpendicular distances from the origin to the planes $\pi_1$ and $\pi_2$ respectively, then $p_1-p_2=$

$\mathbf{a}=2 \hat{\mathbf{i}}-\hat{\mathbf{j}}, \mathbf{b}=2 \hat{\mathbf{j}}-\hat{\mathbf{k}}$ and $\mathbf{c}=2 \hat{\mathbf{k}}-\hat{\mathbf{i}}$ are three vectors and $\mathbf{d}$ is a unit vector perpendicular to $\mathbf{c}$. If $\mathbf{a}, \mathbf{b}$ and $\mathbf{d}$ are coplanar vectors, then $|\mathbf{d} \cdot \mathbf{b}|=$

If $M_1$ is the mean deviation from the mean of the discrete data $44,5,27,20,8,54,9,14,35$ and $M_2$ is the mean deviation from the median of the same data, then $M_1-M_2=$

If two dice are thrown, then the probability of getting co-prime numbers on the dice is

If two cards are drawn at random simultaneously from a well shuffled pack of 52 playing cards, then the probability of getting a cards having a composite number and a card having a number which is a multiple of 3 is

Bag $P$ contains 3 white, 2 red, 5 blue balls and bag $Q$ contains 2 white, 3 red, 5 blue balls. A ball is chosen at random from $P$ and is placed in $Q$. If a ball is chosen from bag $Q$ at random, then the probability that it is a red ball is

If the probability distribution of a random variable $X$ is as follow, then the variance of $X$ is

$X=x$	2	3	5	9
$P(X=x)$	$k$	$2 k$	$3 k^2$	$k$

The mean of a binomial variate $X \sim B(n, p)$ is 1 . If $n>2$ and $P(X=2)=\frac{27}{128}$, then the variance of the distribution is

If the distance from a variable point $P$ to the point $(4,3)$ is equal to the perpendicular distance from $P$ to the line $x+2 y-1=0$, then the equation of the locus of the point $P$ is

$(0, k)$ is the point to which the origin is to be shifted by the translation of the axes so as to remove the first degree terms from the equation $a x^2-2 x y+b y^2-2 x+4 y+1=0$ and $\frac{1}{2} \tan ^{-1}(2)$ is the angle through which the coordinate axes are to be rotated about the origin to remove the $x y$-term from the given equation, then $a+b=$

$\beta$ is the angle made by the perpendicular drawn from origin to the line $L \equiv x+y-2=0$ with the positive $X$-axis in the anticlockwise direction. If $a$ is the $X$-intercept of the line $L=0$ and $p$ is the perpendicular distance from the origin to the line $L=0$, then $a \tan \beta+p^2=$

The line $2 x+y-3=0$ divides the line segment joining the points $A(1,2)$ and $B(-2,1)$ in the ratio $a: b$ at the point $C$. If the point $C$ divides the line segment joining the points $P\left(\frac{b}{3 a},-3\right)$ and $Q\left(-3,-\frac{b}{3 a}\right)$ in the ratio $p: q$, then $\frac{p}{q}+\frac{q}{p}=$

If $Q$ and $R$ are the images of the point $P(2,3)$ with respect to the lines $x-y+2=0$ and $2 x+y-2=0$ respectively, then $Q$ and $R$ lie on

If $(2,-1)$ is the point of intersection of the pair of lines $2 x^2+a x y+3 y^2+b x+c y-3=0$, then $3 a+2 b+c=$

$(1, k)$ is a point on the circle passing through the points $(-1,1),(0,-1)$ and $(1,0)$. If $k \neq 0$, then $k=$

If the tangents $x+y+k=0$ and $x+a y+b=0$ drawn to the circle $S=x^2+y^2+2 x-2 y+1=0$ are perpendicular to each other and $k, b$ are both greater than 1 , then $b-k=$

If $(h, k)$ is the internal centre of similitude of the circles $x^2+y^2+2 x-6 y+1=0$ and $x^2+y^2-4 x+2 y+4=0$, then $4 h=$

The slope of a common tangent to the circles $x^2+y^2-4 x-8 y+16=0$ and $x^2+y^2-6 x-16 y+64=0$ is

$x^2+y^2+2 x-6 y-6=0$ and $x^2+y^2-6 x-2 y+k=0$ are two intersecting circles and $k$ is not an integer. If $\theta$ is the angle between the two circles and $\cos \theta=\frac{-5}{24}$, then $k=$

If $(p, q)$ is the centre of the circle which cuts the three circles $x^2+y^2-2 x-4 y+4=0, x^2+y^2+2 x-4 y+1=0$ and $x^2+y^2-4 x-2 y-11=0$ orthogonally, then $p+q=$

If the focal chord of the parabola $x^2=12 y$, drawn through the point $(3,0)$ intersects the parabola at the points $P$ and $Q$ then the sum of the reciprocals of the abscissae of the points $P$ and $Q$ is

If the normal drawn at the point $P(9,9)$ on the parabola $y^2=9 x$ meets the parabola again at $Q(a, b)$, then $2 a+b=$

The length of the latus rectum of the ellipse $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1(a>b)$ is $\frac{8}{3}$. If the distance from the centre of the ellipse to its focus is $\sqrt{5}$, then $\sqrt{a^2+6 a b+b^2}=$

$S$ is the focus of the ellips $\frac{x^2}{25}+\frac{y^2}{b^2}=1,(b<5)$ lying on the negative $X$-axis and $P(\theta)$ is a point on this ellipes. If the distance between the foci of this ellipse is 8 and $S^{\prime} P=7$, then $\theta=$

The slope of the tangent drawn from the point $(1,1)$ to the hyperbola $2 x^2-y^2=4$ is

$A(2,3, k), B(-1, k,-1)$ and $C(4,-3,2)$ are the vertices of $\triangle A B C$. If $A B=A C$ and $k>0$, then $\triangle A B C$ is

If $A(1,2,-3), B(2,3,-1)$ and $C(3,1,1)$ are the vertices of $\triangle A B C$, then $\left|\frac{-\cos A}{\cos B}\right|=$

If $a, b$ and $c$ are the intercepts made on $X, Y, Z$-axes respectively by the plane passing through the points $(1,0,-2),(3,-1,2)$ and $(0,-3,4)$, then $3 a+4 b+7 c=$

If $\lim \limits_{x \rightarrow 4} \frac{2 x^2+(3+2 a) x+3 a}{x^3-2 x^2-23 x+60}=\frac{11}{9}$, then $\lim \limits_{x \rightarrow a} \frac{x^2+9 x+20}{x^2-x-20}=$

If the function $$ f(x)= \begin{cases}\frac{\tan a(x-1)}{x-1}, & \text { if } 04\end{cases} $$ domain, then $6 a+9 b^4=$

If $y=\log \left[\tan \sqrt{\frac{2^x-1}{2^x+1}}\right], x>0$, then $\left(\frac{d y}{d x}\right)_{x=1}=$

If $y=\cos ^{-1}\left(\frac{6 x-2 x^2-4}{2 x^2-6 x+5}\right)$, then $\frac{d y}{d x}=$

If $\log y=y^{\log x}$, then $\frac{d y}{d x}=$

If $y=a \cos 3 x+b e^{-x}$, then $y^{\prime \prime}(3 \sin 3 x-\cos 3 x)=$

The approximate value of $\sec 59^{\circ}$ obtained by taking $1^{\circ}$ $=0.0174$ and $\sqrt{3}=1.732$ is

The equation of the normal drawn to the curve $y^3=4 x^5$ at the point $(4,16)$ is

A point $P$ is moving on the curve $x^3 y^4=2^7$. The $x$-coordinate of $P$ is decreasing at the rate of 8 units per second. When the point $P$ is at $(2,2)$, the $y$-coordinate of $P$

If the function $f(x)=x^3+a x^2+b x+40$ satisfies the conditions of Rolle's theorem on the interval $[-5,4]$ and $-5,4$ are two roots of the equation $f(x)=0$, then one of the values of $c$ as stated in that theorem is

If $x$ and $y$ are two positive integers such that $x+y=24$ and $x^3 y^5$ is maximum, then $x^2+y^2=$

$\int \sqrt{4 \cos ^2 x-5 \sin ^2 x} \cos x d x=$

$\int\left(\frac{4 \tan ^4 x+3 \tan ^2 x-1}{\tan ^2 x+4}\right) d x=$

$\int\left(\frac{\left(\sin ^4 x+2 \cos ^2 x-1\right) \cos x}{(1+\sin x)^6}\right) d x=$

$\int(\log x)^3 d x=$

$\int_0^\pi\left(\sin ^3 x+\cos ^2 x\right)^2 d x=$

$\int_{\frac{-\pi}{8}}^{\frac{\pi}{8}} \frac{\sin ^4(4 x)}{1+e^{4 x}} d x=$

The area of the region enclosed by the curves $y^2=4(x+1)$ and $y^2=5(x-4)$ is

If $A$ and $B$ are arbitrary constants, then the differential equation having $y=A e^{-x}+B \cos x$ as its general solution is

The general solution of the differential equation $\frac{d y}{d x}+\frac{\sin (2 x+y)}{\cos x}+2=0$ is

Physics

Which of the following statement regarding nature of physical laws is not correct?

The internal and external diameters of hollow cylinder measured with vernier callipers are $(5.73 \pm 0.01) \mathrm{cm}$ and $(6.01 \pm 0.01) \mathrm{cm}$ respectively. Then, the thickness of the cylinder wall is

A body moving with uniform acceleration, travels a distance of 25 m in the fourth second and 37 m in the sixth second. The distance covered by body in the next two seconds is

A body is projected from the ground at an angle of $\tan ^{-1}(\sqrt{7})$ with the horizontal. At half of the maximum height, the speed of the body is $n$ times the speed of projection. The value of $n$ is

An aircraft executes a horizontal loop of radius 9 km at a constant speed of $540 \mathrm{kmh}^{-1}$. The wings of the aircraft are banked at an angle of (Acceleration due to gravity $=10 \mathrm{~ms}^{-2}$ )

A body thrown vertically upwards from the ground reaches a maximum height $h$. The ratio of the kinetic and potential energies of the body at a height $40 \%$ of $h$ from the ground is

A ball of mass 1.2 kg moving with a velocity of $12 \mathrm{~ms}^{-1}$ makes one-dimensional collision with anothe stationary ball of mass 1.2 kg . If the coefficient of restitution is $\frac{1}{\sqrt{2}}$, then the ratio of the total kinetic energy of the balls after collision and the initial kinetic energy is

An alphabet $T$ made of two similar thin uniform metal plates of each length $L$ and width $a$ is placed on a horizontal surface as shown in the figure. If the alphabet is vertically inverted, the shift in the position of its centre of mass from the horizontal surface is

TG EAPCET 2024 (Online) 10th May Morning Shift Physics - Center of Mass and Collision Question 5 English

A solid sphere and a disc of same mass $M$ and radius $R$ - are kept such that their curved surfaces are in contact and their centres lie along the same horizontal line. The moment of inertia of the two body system about an axis passing through their point of contact and perpendicular to the plane of the disc is

If a body dropped freely from a height of 20 m reaches the surface of a planet with a velocity of $31.4 \mathrm{~ms}^{-1}$. then the length of a simple pendulum that ticks seconds on the planet is

Two stars of masses $M$ and $2 M$ that are at a distance $d$ apart, are revolving one around another. The angular velocity of the system of two stars is ( $G$-Universal gravitational constant)

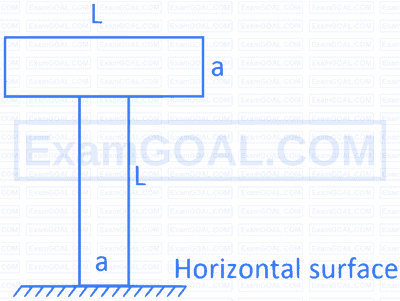
A block of mass 2 kg is tied to one end of a 2 m long metal wire of $1.0 \mathrm{~mm}^2$ area of cross-section and rotated in a vertical circle such that the tension in the wire is zero at the highest point. If the maximum elongation in the wire is 2 mm , the Young's modulus of the metal is

(Acceleration due to gravity $=10 \mathrm{~ms}^{-2}$ )

A big liquid drop splits into $n$ similar small drops under isothermal conditions, then in the process

A wooden cube of side 10 cm floats at the interface between water and oil with its lower surface 3 cm below the interface. If the density of oil is $0.9 \mathrm{~g} \mathrm{~cm}^{-3}$, the mass of the wooden cube is

37 g of ice at $0^{\circ} \mathrm{C}$ temperature is mixed with 74 g of water at $70^{\circ} \mathrm{C}$ temperature. The resultant temperature is (specific heat capacity of water $=1 \mathrm{cal} {\mathrm{g}^{-1o}} \mathrm{C}^{-1}$ and latent heat of fusion of ice $=80 \mathrm{cal} \mathrm{g}^{-1}$ )

The thickness of a uniform rectangular metal plate is 5 mm and the area of each surface is $5 \mathrm{~cm}^5$. In steady state, the temperature difference between the two surfaces of the plate is $14^{\circ} \mathrm{C}$. If the heat flowing through the plate in one second from one surface to the other surface is 42 J , then the thermal conductivity of the metal is

The ratio of the specific heat capacities of a gas is 1.5 . When the gas undergoes adiabatic process, its volume is doubled and pressure becomes $p_1$. When the gas undergoes isothermal process, its volume is doubled and pressure becomes $p_2$. If $p_1=p_2$, the ratio of the initial pressures of the gas when it undergoes adiabatic and isothermal processes is

A vessel contains hydrogen and nitrogen gases in the ratio $2: 3$ by mass. If the temperature of the mixture of the gases is $30^{\circ} \mathrm{C}$, then the ratio of the average kinetic energies per molecule of hydrogen and nitrogen gases is (Molecular mass of hydrogen $=2$ and molecular mass of nitrogen $=28$ )

The difference between the fundamental frequencies of an open pipe and a closed pipe of same length is 100 Hz . The difference between the frequencies of the second harmonic of the open pipe and the third harmonic of the closed pipe is

The displacement equations of sound waves produced by two sources are given by $y_1=5 \sin 400 \pi t$ and $y_2=8 \sin 408 \pi t$, where $t$ is time in seconds. If the waves are produced simultaneously, the number of beats produced per minute is

When an object of height 12 cm is placed at a distance from a convex lens, an image of height 18 cm is formed on a screen. Without changing the positions of the object and the screen. If the lens is moved towards the screen, another clear image is formed on the screen. The height of this image is

A thin plano-convex lens of focal length 73.5 cm has a circular aperture of diameter 8.4 cm . If the refractive index of the material of the lens is $\frac{5}{3}$, then the thickness of the lens is nearly

In Young's double slit experiment, intensity of light at a point on the screen, where the path difference becomes $\lambda$ is $I$. The intensity at a point on the screen, where the path difference becomes $\frac{\lambda}{3}$ is