Chemistry
1. A pair of compounds having the same boiling points are 2. Identify A, B and C in the sequence.
$$\mathrm{CH}_3 \mathrm{CH}_2 \mathrm{Br} \underset{\text { Alc. }}{\stackrel{\math 3. $$\begin{aligned}
& \mathrm{CH}_3-\mathrm{CH}=\mathrm{CH}-\mathrm{CH}_2 \mathrm{OH} \stackrel{\mathrm{PCC}}{\longrightar 4. If a didentate ligand ethane-1, 2-diamine is progressively added in the molar ratio en : Ni :: 1: 1, 2 : 1, 3 : 1 to $$\ 5. Which of the following is an organometallic compound 6. A better reagent to oxidise primary alcohols into aldehyde is 7. In the reaction,
$${C_6}{H_5}CN\mathrel{\mathop{\kern0pt\longrightarrow}
\limits_{(ii)\,{H_3}{O^ + }}^{(i)\,SnC{l_2} + H 8. Compounds P and R in the following reaction are
$$\mathrm{C{H_3}CHO\mathrel{\mathop{\kern0pt\longrightarrow}
\limits_{(i 9. Aniline does not undergo 10. The heating of phenyl methyl ether with HI produces an aromatic compound $$A$$ which on treatment with conc. $$\mathrm{H 11.
Y in the above reaction is 12. Sucrose is dextrorotatory but after hydrolysis the mixture show laevorotation, this is because of 13. The correct order of match between column X and column Y is
.tg {border-collapse:collapse;border-spacing:0;}
.tg td{bo 14. In the reaction,
P, Q and R respectively are 15. Thyroxine produced in the thyroid gland is an iodinated derivative of 16. Which one of the following is a non-narcotic analgesic?
17. Receptors are proteins and crucial to body communication process. These receptors are embedded in 18. Which of the following monomers form biodegradable polymers ? 19. Match the List-I with List - I in the following.
.tg {border-collapse:collapse;border-spacing:0;}
.tg td{border-color: 20. The correct order of first ionisation enthalpy of given elements is 21. Which of the following statement is incorrect? 22. A gas at a pressure of $$2 \mathrm{~atm}$$ is heated from $$25^{\circ} \mathrm{C}$$ to $$323^{\circ} \mathrm{C}$$ and si 23. Lattice enthalpy for $$\mathrm{NaCl}$$ is $$+788 \mathrm{~kJ} \mathrm{~mol}^{-1}$$ and $$\Delta H_{\text {hyd }}^{\circ} 24. At $$500 \mathrm{~K}$$, for a reversible reaction $$A_2(g)+B_2(g) \rightleftharpoons 2 A B(g)$$ in a closed container, $ 25. A weak acid with $$\mathrm{p} K_a ~5.9$$ and weak base with $$\mathrm{p} K_b ~5.8$$ are mixed in equal proportions. $$\m 26. Temperature of $$25^{\circ} \mathrm{C}$$ in Fahrenheit and Kelvin scale respectively are 27. The number of protons, neutrons and electrons in the ion $${ }_{16}^{32} \mathrm{~S}^{2-}$$ respectively are
28. A pair of amphoteric oxides is 29. The composition of water gas is 30. IUPAC name of the compound is
31. Among the following.
The set which represents aromatic species is 32. Which one of the following gases converts haemoglobin into carboxy haemoglobin? 33. What is the oxidation number of $$\mathrm{S}$$ in $$\mathrm{H}_2 \mathrm{S}_2 \mathrm{O}_8$$ ? 34. A $$30 \%$$ solution of hydrogen peroxide is 35. If '$$a$$' stands for the edge length of the cubic systems. The ratio of radii in simple cubic, body centred cubic and f 36. Dimerisation of solute molecules in low dielectric constant solvent is due to 37. The swelling in feet and ankles of an aged person due to sitting continuously for long hours during travel, is reduced b 38. A sample of water is found to contain $$5.85 \%$$ $$\left(\frac{w}{w}\right)$$ of $$A B$$ (molecular mass 58.5 ) and $$9 39. Match the column A (type of crystalline solid) with the column B (example for each type)
.tg {border-collapse:collapse 40. A metal crystallises in a body centred cubic lattice with the metallic radius $$\sqrt3\mathop A\limits^o $$. The volume 41. The resistance of $$0.1 \mathrm{~M}$$ weak acid $$\mathrm{H} A$$ in a conductivity cell is $$2 \times 10^3 \mathrm{~Ohm} 42. In which one of the following reactions, rate constant has the unit $$\mathrm{mol} \mathrm{~L}^{-1} \mathrm{~s}^{-1}$$ ? 43. For a reaction, the value of rate constant at $$300 \mathrm{~K}$$ is $$6.0 \times 10^5 \mathrm{~s}^{-1}$$. The value of 44. The rate constant $$k_1$$ and $$k_2$$ for two different reactions are $$10^{16} \times e^{-2000 / T}$$ and $$10^{15} \ti 45. During the electrolysis of brine, by using inert electrodes, 46. Consider the following 4 electrodes
$$\begin{aligned}
& \mathrm{A}: \mathrm{Ag}^{+}(0.0001 \mathrm{M}) / \mathrm{Ag}(s) 47. When $$\mathrm{FeCl}_3$$ is added to excess of hot water gives a sol '$$X$$'. When $$\mathrm{FeCl}_3$$ is added to $$\ma 48. The reducing agent in the given equations
$$4 \mathrm{Ag}(s)+8 \mathrm{CN}^{-}(a q)+2 \mathrm{H}_2 \mathrm{O}(a q)+\math 49. For the formation of which compound in Ellingham diagram $$\Delta G^{\circ}$$ becomes more and more negative with increa 50. Which of the following compound does not give dinitrogen on heating? 51. Aqueous solution of raw sugar when passed over beds of animal charcoal, it becomes colourless. Pick the correct set of t 52. For Freundlich adsorption isotherm, a graph of $$\log (x / m)$$ vs $$\log (p)$$ gives a straight line. The slope of line 53. In solid state, $$\mathrm{PCl}_5$$ is a/an 54. In which one of the following pairs, both the elements does not have $$(n-1) d^{10} n s^2$$ configuration in its element 55. Which of the following is correct with respect to melting point of a transition element? 56. $$a \mathrm{MnO}_4^{-}+b \mathrm{~S}_2 \mathrm{O}_3^{2-}+\mathrm{H}_2 \mathrm{O} \longrightarrow x \mathrm{MnO}_2 +y \m 57. Which formula and name combination is incorrect? 58. Which of the following system in an octahedral complex has maximum unpaired electrons? 59. The correct decreasing order of basicity of hydrides of group- 15 elements is 60. Which one of the following oxoacids of phosphorus can reduce $$\mathrm{AgNO}_3$$ to metallic silver?
Mathematics
1. If a line makes an angle of $$\frac{\pi}{3}$$ with each $$X$$ and $$Y$$ axis, then the acute angle made by $$\mathrm{Z}$ 2. The length of perpendicular drawn from the point $$(3,-1,11)$$ to the line $$\frac{x}{2}=\frac{y-2}{3}=\frac{z-3}{4}$$ i 3. The equation of the plane through the points $$(2,1,0),(3,2,-2)$$ and $$(3,1,7)$$ is 4. The point of intersection of the line $$x+1=\frac{y+3}{3}=\frac{-z+2}{2}$$ with the plane $$3 x+4 y+5 z=10$$ is 5. If $$(2,3,-1)$$ is the foot of the perpendicular from $$(4,2,1)$$ to a plane, then the equation of the plane is 6. $$|\mathbf{a} \times \mathbf{b}|^2+|\mathbf{a} \cdot \mathbf{b}|^2=144$$ and $$|\mathbf{a}|=4$$, then $$|\mathbf{b}|$$ i 7. If $$\mathbf{a}+2 \mathbf{b}+3 \mathbf{c}=0$$ and $$(\mathbf{a} \times \mathbf{b})+(\mathbf{b} \times \mathbf{c})+(\math 8. A bag contains $$2 n+1$$ coins. It is known that $$n$$ of these coins have head on both sides whereas, the other $$n+1$$ 9. Let $$A=\{x, y, z, u\}$$ and $$B=\{a, b\}$$. A function $$f: A \rightarrow B$$ is selected randomly. The probability tha 10. The shaded region in the figure given is the solution of which of the inequations?
11. If $$A$$ and $$B$$ are events, such that $$P(A)=\frac{1}{4}, P(A / B)=\frac{1}{2}$$ and $$P(B / A)=\frac{2}{3}$$, then $ 12. The value of
$$e^{\log _{10} \tan 1^{\circ}+\log _{10} \tan 2^{\circ}+\log _{10} \tan 3^{\circ}+\ldots+\log _{10} \tan 8 13. $$\text { The value of }\left|\begin{array}{ccc}
\sin ^2 14^{\circ} & \sin ^2 66^{\circ} & \tan 135^{\circ} \\
\sin ^2 6 14. The modulus of the complex number $$\frac{(1+i)^2(1+3 i)}{(2-6 i)(2-2 i)}$$ is 15. Given that $$a, b$$ and $$x$$ are real numbers and $$a 16. Ten chairs are numbered as 1 to 10. Three women and two men wish to occupy one chair each. First the women choose the ch 17. Which of the following is an empty set? 18. If $$f(x)=a x+b$$, where $$a$$ and $$b$$ are integers, $$f(-1)=-5$$ and $$f(3)=3$$, then $$a$$ and $$b$$ are respectivel 19. If $$p\left(\frac{1}{q}+\frac{1}{r}\right), q\left(\frac{1}{r}+\frac{1}{p}\right), r\left(\frac{1}{p}+\frac{1}{q}\right) 20. A line passes through $$(2,2)$$ and is perpendicular to the line $$3 x+y=3$$. Its $$y$$-intercept is 21. The distance between the foci of a hyperbola is 16 and its eccentricity is $$\sqrt{2}$$. Its equation is 22. If $$\lim _\limits{x \rightarrow 0} \frac{\sin (2+x)-\sin (2-x)}{x}=A \cos B$$, then the values of $$A$$ and $$B$$ respe 23. If $$n$$ is even and the middle term in the expansion of $$\left(x^2+\frac{1}{x}\right)^n$$ is $$924 x^6$$, then $$n$$ i 24. $$n$$th term of the series $$1+\frac{3}{7}+\frac{5}{7^2}+\frac{1}{7^2}+\ldots$$ is 25. $$f: R \rightarrow R$$ and $$g:[0, \infty) \rightarrow R$$ defined by $$f(x)=x^2$$ and $$g(x)=\sqrt{x}$$. Which one of t 26. Let $$f: R \rightarrow R$$ be defined by $$f(x)=3 x^2-5$$ and $$g: R \rightarrow R$$ by $$g(x)=\frac{x}{x^2+1}$$, then $ 27. Let the relation $$R$$ be defined in $$N$$ by $$a R b$$, if $$3 a+2 b=27$$, then $$R$$ is 28. Let $$f(x)=\sin 2 x+\cos 2 x$$ and $$g(x)=x^2-1$$ then $$g(f(x))$$ is invertible in the domain 29. The contrapositive of the statement.
"If two lines do not intersect in the same plane, then they are parallel." is 30. The mean of 100 observations is 50 and their standard deviation is 5. Then, the sum of squares of all observations is 31. If $$x\left[\begin{array}{l}3 \\ 2\end{array}\right]+y\left[\begin{array}{r}1 \\ -1\end{array}\right]=\left[\begin{array 32. If $$A$$ and $$B$$ are two matrices, such that $$A B=B$$ and $$B A=A$$, then $$A^2+B^2$$ equals to 33. If $$A=\left[\begin{array}{cc}2-k & 2 \\ 1 & 3-k\end{array}\right]$$ is singular matrix, then the value of $$5 k-k^2$$ i 34. The area of a triangle with vertices $$(-3,0)$$, $$(3,0)$$ and $$(0, k)$$ is 9 sq units, find the value of $$k$$ is 35. If $$\Delta=\left|\begin{array}{ccc}1 & a & a^2 \\ 1 & b & b^2 \\ 1 & c & c^2\end{array}\right|$$ and $$\Delta_1=\left|\ 36. If $$\sin ^{-1}\left(\frac{2 a}{1+a^2}\right)+\cos ^{-1}\left(\frac{1-a^2}{1+a^2}\right)=\tan ^{-1}\left(\frac{2 x}{1-x^ 37. The value of $$\cot ^{-1}\left[\frac{\sqrt{1-\sin x}+\sqrt{1+\sin x}}{\sqrt{1-\sin x}-\sqrt{1+\sin x}}\right]$$, where $ 38. The function $$f(x)=\cot x$$ is discontinuous on every point of the set 39. If the function is $$f(x)=\frac{1}{x+2}$$, then the point of discontinuity of the composite function $$y=f(f(x))$$ is 40. If $$y=a \sin x+b \cos x$$, then $$y^2+\left(\frac{d y}{d x}\right)^2$$ is a 41. If $$f(x)=1+n x+\frac{n(n-1)}{2} x^2+\frac{n(n-1)(n-2)}{6} x^3+\ldots+x^n$$, then $$f^n(1)$$ is equal to : 42. If $$A=\left[\begin{array}{cc}1 & \tan \alpha / 2 \\ -\tan \alpha / 2 & 1\end{array}\right]$$ and $$A B=I$$, then $$B$$ 43. If $$u=\sin ^{-1}\left(\frac{2 x}{1+x^2}\right)$$ and $$v=\tan ^{-1}\left(\frac{2 x}{1-x^2}\right)$$, then $$\frac{d u}{ 44. The distance '$$s$$' in meters travelled by a particle in '$$t$$' seconds is given by $$s=\frac{2 t^3}{3}-18 t+\frac{5}{ 45. A particle moves along the curve $$\frac{x^2}{16}+\frac{y^2}{4}=1$$. When the rate of change of abscissa is 4 times that 46. An enemy fighter jet is flying along the curve, given by $$y=x^2+2$$. A soldier is placed at $$(3,2)$$ wants to shoot do 47. $$\int\limits_2^8 \frac{5^{\sqrt{10-x}}}{5^{\sqrt{x}}+5^{\sqrt{10-x}}} d x \text { is equals to :}$$ 48. $$\int \sqrt{\operatorname{cosec} x-\sin x} d x$$ is equals to 49. If $$f(x)$$ and $$g(x)$$ are two functions with $$g(x)=x-\frac{1}{x}$$ and $$f \circ g(x)=x^3-\frac{1}{x^3}$$, then $$f^ 50. A circular plate of radius $$5 \mathrm{~cm}$$ is heated. Due to expansion, its radius increase at the rate of $$0.05 \ma 51. $$\int_{-2}^0\left(x^3+3 x^2+3 x+3+(x+1) \cos (x+1)\right) d x$$ is equals to 52. $$\int\limits_0^\pi \frac{x \tan x}{\sec x \cdot \operatorname{cosec} x} d x$$ is equals to 53. $$\int \sqrt{5-2 x+x^2} d x$$ is equals to 54. $$\int \frac{1}{1+3 \sin ^2 x+8 \cos ^2 x} d x$$ is equals to 55. If a curve passes through the point $$(1,1)$$ and at any point $$(x, y)$$ on the curve, the product of the slope of its 56. The degree of the differential equation $$1+\left(\frac{d y}{d x}\right)^2+\left(\frac{d^2 y}{d x^2}\right)^2=\sqrt[3]{\ 57. If $$|\vec{a}+\vec{b}|=|\vec{a}-\vec{b}|$$, then 58. The component of $$\hat{\mathbf{i}}$$ in the direction of the vector $$\hat{\mathbf{i}}+\hat{\mathbf{j}}+2 \hat{\mathbf{ 59. In the interval $$(0, \pi / 2)$$ area lying between the curves $$y=\tan x$$ and $$y=\cot x$$ and the $$X$$-axis is 60. The area of the region bounded by the line $$y=x+1$$ and the lines $$x=3$$ and $$x=5$$ is
Physics
1. For a point object, which of the following always produces virtual image in air? 2. For a given pair of transparent media, the critical angle for which colour is maximum? 3. An equiconvex lens made of glass of refractive index $$\frac{3}{2}$$ has focal length $$f$$ in air. It is completely imm 4. A point object is moving at a constant speed of 1 ms$$^{-1}$$ along the principal axis of a convex lens of focal length 5. When light propagates through a given homogeneous medium, the velocities of 6. Total impedance of a series $$L$$-$$C$$-$$R$$ circuit varies with angular frequency of the $$\mathrm{AC}$$ source connec 7. The ratio of the magnitudes of electric field to the magnetic field of an electromagnetic wave is of the order of 8. A 60 W source emits monochromatic light of wavelength 662.5 nm. The number of photons emitted per second is 9. In an experiment to study photoelectric effect the observed variation of stopping potential with frequency of incident r 10. In the Rutherford's alpha scattering experiment, as the impact parameter increases, the scattering angle of the alpha pa 11. Three energy levels of hydrogen atom and the corresponding wavelength of the emitted radiation due to different electron 12. An unpolarised light of intensity $$I$$ is passed through two polaroids kept one after the other with their planes paral 13. In the Young's double slit experiment, the intensity of light passing through each of the two double slits is $$2 \times 14. A radioactive sample has half-life of 3 years. The time required for the activity of the sample to reduce to $$\frac{1}{ 15. When a $$p$$-$$n$$ junction diode is in forward bias, which type of charge carriers flows in the connecting wire?
16. A full-wave rectifier with diodes $$D_1$$ and $$D_2$$ is used to rectify $$50 \mathrm{~Hz}$$ alternating voltage. The di 17. A truth table for the given circuit is
18. The energy gap of an LED is $$2.4 \mathrm{~eV}$$. When the LED is switched ON, the momentum of the emitted photons is 19. In the following equation representing $$\beta^{-}$$ decay, the number of neutrons in the nucleus $$X$$ is
$${ }_{83}^{2 20. A nucleus with mass number 220 initially at rest emits an alpha particle. If the $$Q$$ value of reaction is $$5.5 ~\math 21. A particle is in uniform circular motion, related to one complete revolution of the particle, which among the statements 22. A body of mass $$10 \mathrm{~kg}$$ is kept on a horizontal surface. The coefficient of kinetic friction between the body 23. A ball of mass $$0.2 \mathrm{~kg}$$ is thrown vertically down from a height of $$10 \mathrm{~m}$$. It collides with the 24. The moment of inertia of a rigid body about an axis 25. Seven identical discs are arranged in a planar pattern, so as to touch each other as shown in the figure. Each disc has 26. The true length of a wire is $$3.678 \mathrm{~cm}$$. When the length of this wire is measured using instrument $$A$$, th 27. A body is moving along a straight line with initial velocity $$v_0$$. Its acceleration $$a$$ is constant. After $$t$$ se 28. A closed water tank has cross-sectional area $$A$$. It has a small hole at a depth of $$h$$ from the free surface of wat 29. $$100 \mathrm{~g}$$ of ice at $$0^{\circ} \mathrm{C}$$ is mixed with $$100 \mathrm{~g}$$ of water at $$100^{\circ} \math 30. The $$p$$-$$V$$ diagram of a Carnot's engine is shown in the graph below. The engine uses 1 mole of an ideal gas as work 31. When a planet revolves around the Sun, in general, for the planet 32. A stretched wire of a material whose young's modulus $$Y=2 \times 10^{11} ~\mathrm{Nm}^{-2}$$ has poisson's ratio 0.25 . 33. The speed of sound in an ideal gas at a given temperature $$T$$ is $$v$$. The rms speed of gas molecules at that tempera 34. A positively charged glass rod is brought near uncharged metal sphere, which is mounted on an insulated stand. If the gl 35. In the situation shown in the diagram, magnitude, if $$q < < |Q|$$ and $$r >>>a$$. The net force on the f 36. Pressure of ideal gas at constant volume is proportional to ......... 37. A block of mass $$m$$ is connected to a light spring of force constant $$k$$. The system is placed inside a damping medi 38. Five capacitance each of value $$1 ~\mu \mathrm{F}$$ are connected as shown in the figure. The equivalent capacitance be 39. A uniform electric field vector $$\mathbf{E}$$ exists along horizontal direction as shown. The electric potential at $$A 40. A parallel plate capacitor of capacitance $$C_1$$ with a dielectric slab in between its plates is connected to a battery 41. A cubical Gaussian surface has side of length $$a=10 \mathrm{~cm}$$. Electric field lines are parallel to $$X$$-axis as 42. Electric field at a distance $$r$$ from an infinitely long uniformly charged straight conductor, having linear charge de 43. A wire of resistance $$R$$ is connected across a cell of emf $$(\varepsilon)$$ and internal resistance $$(r)$$. The curr 44. For a given electric current the drift velocity of conduction electrons in a copper wire is $$v_d$$ and their mobility i 45. Ten identical cells each emf $$2 \mathrm{~V}$$ and internal resistance $$1 ~\Omega$$ are connected in series with two ce 46. The equivalent resistance between the points A and B in the following circuit is
47. A charged particle is subjected to acceleration in a cyclotron as shown. The charged particle undergoes increase in its 48. The resistance of a carbon resistor is $$4.7 ~\mathrm{k} \Omega \pm 5 \%$$. The colour of the third band is 49. The four bands of a colour coded resistor are of the colours grey, red, gold and gold. The value of the resistance of th 50. A positively charged particle $$q$$ of mass $m$ is passed through a velocity selector. It moves horizontally rightward w 51. The Curie temperature of cobalt and iron are $$1400 \mathrm{~K}$$ and $$1000 \mathrm{~K}$$ respectively. At $$T=1600 \ma 52. The torque acting on a magnetic dipole placed in uniform magnetic field is zero, when the angle between the dipole axis 53. The horizontal component of Earth's magnetic field at a place is $$3 \times 10^{-5} \mathrm{~T}$$. If the dip at that pl 54. A proton and an alpha-particle moving with the same velocity enter a uniform magnetic field with their velocities perpen 55. A moving coil galvanometer is converted into an ammeter of range 0 to $$5 \mathrm{~mA}$$. The galvanometer resistance is 56. A square loop of side $$2 \mathrm{~cm}$$ enters a magnetic field with a constant speed of $$2 \mathrm{~cm} \mathrm{~s}^{ 57. In series $$L C R$$ circuit at resonance, the phase difference between voltage and current is 58. An ideal transformer has a turns ratio of 10. When the primary is connected to $$220 \mathrm{~V}, 50 \mathrm{~Hz}$$ as s 59. The current in a coil changes from $$2 \mathrm{~A}$$ to $$5 \mathrm{~A}$$ in $$0.3 \mathrm{~s}$$. The magnitude of emf i 60. A metallic rod of length $$1 \mathrm{~m}$$ held along east-west direction is allowed to fall down freely. Given horizont
1
KCET 2023
MCQ (Single Correct Answer)
+1
-0
A square loop of side $$2 \mathrm{~cm}$$ enters a magnetic field with a constant speed of $$2 \mathrm{~cm} \mathrm{~s}^{-1}$$ as shown. The front edge enters the field at $$t=0 \mathrm{~s}$$. Which of the following graph correctly depicts the induced emf in the loop?
(Take clockwise direction positive)

A
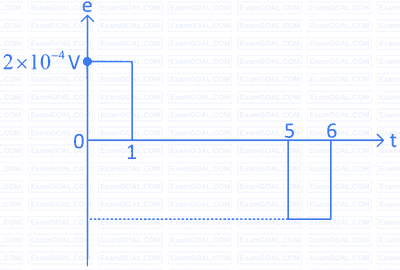
B

C
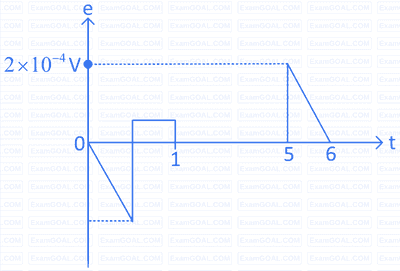
D
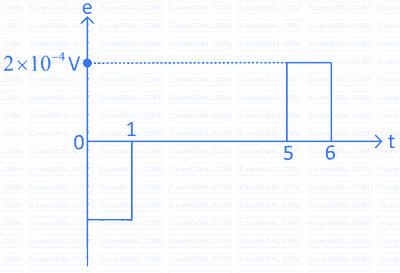
2
KCET 2023
MCQ (Single Correct Answer)
+1
-0
In series $$L C R$$ circuit at resonance, the phase difference between voltage and current is
A
Zero
B
$$\pi$$
C
$$\frac{\pi}{4}$$
D
$$\frac{\pi}{2}$$
3
KCET 2023
MCQ (Single Correct Answer)
+1
-0
An ideal transformer has a turns ratio of 10. When the primary is connected to $$220 \mathrm{~V}, 50 \mathrm{~Hz}$$ as source, the power output is
A
10 times the power input
B
$$\frac{1}{10}$$th the power input
C
equal to power input
D
zero
4
KCET 2023
MCQ (Single Correct Answer)
+1
-0
The current in a coil changes from $$2 \mathrm{~A}$$ to $$5 \mathrm{~A}$$ in $$0.3 \mathrm{~s}$$. The magnitude of emf induced in the coil is $$1.0 \mathrm{~V}$$. The value of self-inductance of the coil is
A
$$1.0 \mathrm{~mH}$$
B
$$100 \mathrm{~mH}$$
C
$$0.1 \mathrm{~mH}$$
D
$$10 \mathrm{~mH}$$