Current Electricity · Physics · COMEDK
MCQ (Single Correct Answer)
An electric coil is rated $400 \mathrm{~W}, 200 \mathrm{~V}$. It is cut into two equal parts and connected in parallel to the same source of 200 V . Calculate the percentage increase in energy produced per second.
A parallel combination of ' $n$ ' cells of emf ' $E$ ' and internal resistance ' $r$ ' each, are connected across the external resistance ' $R$ '. If the external resistance ' $R$ ' is negligibly small, then the current ' $I$ ' through the external resistance is:
The dimensional formula for specific resistance is:
The voltage - current graph for a metal wire of uniform area of cross section at two different temp $T$ and $T^{\prime}$ is shown.
Then choose the correct statement:
A wire of length 1 m has a resistance of $20 \Omega$ at $0^{\circ} \mathrm{C}$. It is uniformly stretched so that its length increases by $21 \%$. Assuming the volume of the wire remains constant, the percentage change in resistance is $n \%$. Alternatively, if the wire is heated [without stretching] through a temperature of $27^{\circ} \mathrm{C}$ and if the temperature coefficient of resistance of the material of wire is $0.004 K^{-1}$, the percentage change in resistance is $m \%$. The values of $m$ and $n$ are:
A current of 3 A enters one vertex P of an equilateral triangle PQR having three resistors of $1 \Omega$ each forming the sides of the equilateral triangle as shown. The value of $i_2$ in amperes is:
Current in a conductor is expressed as $I=8 t^3+3 t^2+2$, where current I is measured in amperes and time t is measured in seconds. What is the charge that flows through a cross-section of the conductor between time $t=1 \mathrm{~s}$ to $t=2 \mathrm{~s}$ ?
In the given circuit, an ideal voltmeter connected across $6 \Omega$ reads 5 V . The internal resistance $r$ of each cell is:
The power dissipated across the $16 \Omega$ resistor in the circuit is 2 watts. The power dissipated in watt units across the $4 \Omega$ resistor is:
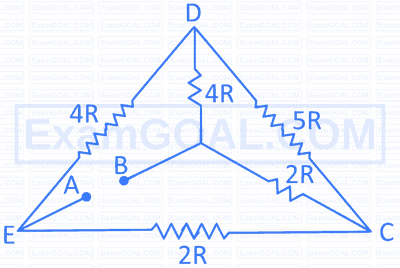
The net resistance between the points A and B in the circuit given below is:
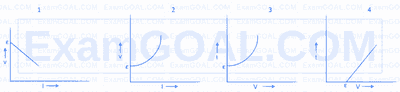
A student measures the terminal potential difference $V$ of a cell of emf $\varepsilon$ and internal resistance $r$ as a function of the current I flowing through it. Which of the following graphs will give the values of emf $\varepsilon$ and internal resistance $r$ ?
Three bulbs of $$40 \mathrm{~W}, 60 \mathrm{~W}$$, and $$100 \mathrm{~W}$$ are arranged in series with a $$220 \mathrm{~V}$$ source. The maximum light is obtained from
The resistance of a $$10 \mathrm{~m}$$ long wire is $$10 \Omega$$. Its length is increased by $$25 \%$$ by stretching the wire uniformly. The new resistance is
Four resistors, each of resistance R, are connected as shown in the figure below.
A voltmeter of resistance $$1000 \Omega .0 .5 \mathrm{~V} /$$ div is to be converted into a voltmeter to make it to read $$=1 \mathrm{~V} / \mathrm{div}$$. The value of high resistance to be connected in series with it is
The resistance of a wire at room temperature $$20^{\circ} \mathrm{C}$$ is found to be $$10 \Omega$$. If resistance of the wire increases by $$10 \%$$, then the temperature of the wire will be (The temperature coefficient of the material of the wire is $$0.002 /{ }^{\circ} \mathrm{C}$$)
The potential difference between the points $$\mathrm{P}$$ and $$\mathrm{Q}$$ of the arrangement shown in figure is
An electron starting from rest and moving with the velocity $$\mathrm{v}$$ through a potential difference $$\mathrm{V}$$ is shown by the graphs below. Identify the correct graph.
Two identical moving coil galvanometers have $$10 \Omega$$ resistance and full-scale deflection at $$2 \mu \mathrm{A}$$ current. One of them is converted into a voltmeter of range $$10 \mathrm{~mV}$$ and the other into an ammeter of range $$1 \mathrm{~mA}$$ using appropriate resistors. The ratio of resistance of the converted voltmeter to that of the ammeter is
A wire of uniform cross section and resistance 4 ohms is bent in the shape of square ABCD. Point A is connected to a point $$\mathrm{P}$$ on DC by a wire AP of resistance $$1 \mathrm{ohm}$$. When a potential difference is applied between $$\mathrm{A}$$ and $$\mathrm{C}$$, the points $$\mathrm{B}$$ and $$\mathrm{P}$$ are seen to be in same potential. What is the resistance of part $$\mathrm{DP}$$ ?
A storage battery of emf $$28.0 \mathrm{~V}$$ and internal resistance $$0.5 \Omega$$ is being charged by a $$140 \mathrm{~V}$$ dc supply using a series resistor of $$27.5 \Omega$$. The terminal voltage of the battery during charging is
The resistance of the galvanometer and shunt of an ammeter are $$90 \mathrm{~ohm}$$ and $$10 \mathrm{~ohm}$$ respectively, then the fraction of the main current passing through the galvanometer and the shut respectively are:
A $$500 \mathrm{~W}$$ heating unit is designed to operate on a $$400 \mathrm{~V}$$ line. If line voltage drops to $$160 \mathrm{~V}$$, the percentage drop in heat output will be:
A cell of emf E and internal resistance r is connected to two external resistances $$\mathrm{R_1}$$ and $$\mathrm{R_2}$$ and a perfect ammeter. The current in the circuit is measured in four different situations:
(a) without any external resistance in the circuit.
(b) with resistance $$\mathrm{R_1}$$ only
(c) with $$\mathrm{R_1}$$ and $$\mathrm{R_2}$$ in series combination.
(d) with $$\mathrm{R_1}$$ and $$\mathrm{R_2}$$ in parallel combination.
The currents measured in the four cases in ascending order are
The current through a conductor is '$$\mathrm{a}$$' when the temperature is $$0^{\circ} \mathrm{C}$$. It is '$$\mathrm{b}$$' when the temperature is $$100^{\circ} \mathrm{C}$$. The current through the conductor at $$220^{\circ} \mathrm{C}$$ is
On increasing the temperature of a conductor, its resistance increases because
A galvanometer having a resistance of $$8 \Omega$$ is shunted by a wire of resistance $$2 \Omega$$. If the total current is $$1 \mathrm{~A}$$, the part of it passing through the shunt will be
Two cells with the same emf $$E$$ and different internal resistances $$r_1$$ and $$r_2$$ are connected in series to an external resistance $$R$$. If the potential difference across the first cell is zero then value of $$R$$.
The current sensitivity of a galvanometer having 20 divisions is $$10 \mu \mathrm{A} /$$ div. If the resistance of the galvanometer is $$100 \Omega$$ then the value of the resistance to be used to convert this galvanometer in to an voltmeter to read up to $$1 \mathrm{~V}$$ is :
The electric flux from cube of side $$1 \mathrm{~m}$$ is '$$\Phi$$' When the side of the cube is made $$3 \mathrm{~m}$$ and the charge enclosed by the cube is made one third of the original value, then the flux from the bigger cube will be :
A resistor of wire $$24 \mathrm{~cm}$$ length and resistance $$8 \Omega$$ is stretched in to a uniform wire of $$48 \mathrm{~cm}$$ length, then the new resistance will be :
The SI unit of electrical conductivity is :
The current passing through the 100$$\Omega$$ resistor in the given electrical circuit is :
A battery is made of 12 cells having emf $$5 \mathrm{~V}$$ each. If three cells are unknowingly connected wrong, the resultant emf of the battery will be:
The current drawn by the primary coil of an ideal transformer, which steps up $$22 \mathrm{~V}$$ into $$220 \mathrm{~V}$$, to operate a device having a load resistance $$110 \Omega$$ is:
A galvanometer having a resistance of 4 $$\Omega$$ is shunted by a wire of resistance 2 $$\Omega$$. If the total current is 1.5 A, the current passing through shunt is
A cell of emf 2 V is connected with a load of resistance 1.5 $$\Omega$$. The power delivered by the cell to the load is maximum, then power transferred to the load is
The resistance of a wire is R ohm. If it is melted and stretched to n times its original length, its new resistance will be
For the given electrical arrangement, what is the value of current I?
If voltage $$V=(200\pm 8)V$$ and current $$I=(20\pm0.5)A$$, then the percentage error in resistance R is
Point out the right statements about the validity of Kirchhoff’s junction rule,
If $$P=Q=R=10\Omega$$ and $$S=20\Omega$$, then what resistance should be joined with S to balance the Wheatstone's network?
To the potentiometer wire of length L and 10$$\Omega$$ resistance, a battery of emf 2.5 V and a resistance R are connected in series. If a potential difference of 1 V is balanced across L/2 length, the value of R in $$\Omega$$ will be