Chemistry
1. The number of protons, neutrons and electrons in $$_6^{13}$$C respectively are 2. The masses of an electron, a proton and a neutron respectively will be n the ratio 3. Match the following species with the correct number of electrons present in them.
.tg {border-collapse:collapse;border 4. The correct order of electronegativity of carbon in various hybridisation states is 5. Which of the following is not arranged in the correct sequence? 6. Which of the following statement is incorrect? 7. Bond order is an inverse measure of 8. Which of the following molecule has the maximum dipole moment? 9. Which compound among the following will
have a permanent dipole moment?
10. Which among the following statements is/are
incorrect regarding real gases?
(i) Their compressibility factor is never eq 11. Which among the following species does not show disproportionation reaction? 12. An alloy of metals X and Y weighs 12 g and
contains atoms X and Y in the ratio of 2 : 5.
The percentage of metal X in th 13. For the reaction, $$\mathrm{H}_2 \mathrm{O}(l) \longrightarrow \mathrm{H}_2 \mathrm{O}(\mathrm{g})$$ at $$T=100^{\circ} 14. At $$60^{\circ} \mathrm{C}$$, dinitrogen tetroxide is dissociated. Find it's standard free energy change at this tempera 15. The solubility of $$\operatorname{AgBr}(s)$$, having solubility product $$5 \times 10^{-10}$$ in $$0.2 \mathrm{~M} \math 16. Le-Chatelier's principle is not applicable to 17. Which of the following does not form double
salts? 18. $$\mathrm{AlF}_3$$ is soluble in HF only in the presence of KF due to formation of 19. What would be the product of following reaction?
$$\mathrm{SiCl}_4 \stackrel{\text { Excess of } \mathrm{H}_2 \mathrm{O} 20. Which among the following is not a greenhouse gas? 21. An organic compound of molecular formula
$$\mathrm{C_6H_6Br_2}$$ has six carbon atoms in a ring
system, two non-conjugat 22. Using Kjeldahl’s method over 1g of a soil
sample, the ammonia evolved could neutralise
25 mL of 1 M H$$_2$$SO$$_4$$. The 23. Which compound among the following is
most reactive towards electrophilic reagents? 24. Which of the following is not explained by hyperconjugation? 25. In the face centered unit cell, the lattice points are present at 26. If the $$K_{\mathrm{H}}$$ values for $$\operatorname{Ar}(g), \mathrm{CO}_2(g), \mathrm{HCHO}(g)$$ and $$\mathrm{CH}_4(\m 27. If 500 mL of CaCl$$_2$$ solution contains 3.01 $$\times$$ 10$$^{22}$$ chloride ions, molarity of the solution will be 28. Which statement among the following is incorrect? 29. For zero order reaction, a plot of $$t_{1 / 2}$$ versus
$$[A]_0$$ will be 30. If hydrogen electrons dipped in two solutions
of pH = 3 and pH = 6 are connected by a salt
bridge, the emf of the result 31. In an adsorption experiment, a graph between $$\log (x / m)$$ versus $$\log p$$ was found to be linear with a slope of $ 32. The correct order of sulphur-oxygen bond in $$\mathrm{SO}_3, \mathrm{~S}_2 \mathrm{O}_3^{2-}$$ and $$\mathrm{SO}_4^{2-}$ 33. Potassium cyanide is made alkaline with
NaOH and boiled with thiosulphate ions.
The solution is cooled and acidified wit 34. Which among the following is coloured? 35. Which of the following complexes formed by nickel is tetrahedral and paramagnetic? 36. Vitamin-B$$_1$$ is 37. Identify the product of the following reaction.
38. The correct order of acidic strength among the following is 39. Identify (Z) in the following reaction.
$$\mathrm{C{H_3}COOH\buildrel {LiAl{H_4}} \over
\longrightarrow (X)\mathrel{\ma 40. Identify the major product of the following reaction.
Mathematics
1. $$f(x)=\sin x+\cos x \cdot g(x)=x^2-1$$, then $$g(f(x))$$ is invertible if 2. If $$f: z \rightarrow z$$ is defined by $$f(x)=x^9-11 x^8-2 x^7+22 x^6+x^4 -12 x^3+11 x^2+x-3, \forall x \in z$$, then $ 3. Let $$f(x)=x^3$$ and $$g(x)=3^x$$, then the quadratic equation whose roots are solutions of the equation $$(f \circ g)(x 4. The trace of the matrix $$A=\left[\begin{array}{ccc}1 & -5 & 7 \\ 0 & 7 & 9 \\ 11 & 8 & 9\end{array}\right]$$ is 5. If $$A, B$$ and $$C$$ are the angles of a triangle, then the system of equations
$$-x+y \cos C+z \cos B=0, x \cos C-y+z 6. If $$\left[\begin{array}{cc}1 & -\tan \theta \\ \tan \theta & 1\end{array}\right]\left[\begin{array}{cc}1 & \tan \theta 7. If $$z_1=2+3 i$$ and $$z_2=3+2 i$$, where $$i=\sqrt{-1}$$, then $$\left[\begin{array}{cc}z_1 & z_2 \\ -\bar{z}_2 & \bar{ 8. What is the value of $$\left|\begin{array}{ccc}a & b & c \\ a-b & b-c & c-a \\ b+c & c+a & a+b\end{array}\right|$$ ? 9. The radius of the circle represented by $$(1+i)(1+3i)(1+7i)=x+iy$$ is $$(i=\sqrt{-1})$$. 10. If $$1, \alpha_1, \alpha_2, \alpha_3$$ and $$\alpha_4$$ are the roots of $$z^5-1=0$$ and $$\omega$$ is a cube root of un 11. If $$a > 0$$ and $$z=x+i y$$, then
$$\log _{\cos ^2 \theta}|z-a|>\log _{\cos ^2 \theta}|z-a i|,(\theta \in R)$$
implies 12. If one root of the equation $$i x^2-2(i+1) x+(2-i)=0$$ is $$(2-i)$$, then the other root is 13. If $$\alpha$$ and $$\beta$$ are the roots of the quadratic equation $$x^2+x+1=0$$, then the equation whose roots are $$\ 14. If $$2, 1$$ and $$1$$ are roots of the equation $$x^3-4 x^2+5 x-2=0$$, then the roots of $$\left(x+\frac{1}{3}\right)^3- 15. If $$f(x)=2x^3+mx^2-13x+n$$ and 2, 3 are the roots of the equation $$f(x)=0$$, then the values of m and n are 16. The value of $${ }^6 P_4+4 \cdot{ }^6 P_3$$ is 17. The number of ways in which 3 boys and
2 girls can sit on a bench so that no two boys
are adjacent is 18. In how many ways can 5 balls be placed in
4 tins if any number of balls can be placed in
any tin? 19. Given, $$\frac{3 x-2}{(x+1)^2(x+3)}=\frac{A}{x+1} +\frac{B}{(x+1)^2}+\frac{C}{x+3}$$, then $$4 A+2 B+4 C$$ is equal to 20. What is the value of $$\cos \left(22 \frac{1}{2}\right)^{\circ}$$ ? 21. If $$\cos \theta=-\sqrt{\frac{3}{2}}$$ and $$\sin \alpha=\frac{-3}{5}$$, where '$$\theta$$' does not lie in the third qu 22. If $$\tan \beta=\frac{\tan \alpha+\tan \gamma}{1+\tan \alpha \tan \gamma}$$, then $$\frac{\sin 2 \alpha+\sin 2 \gamma}{1 23. If $$\sin \left(\frac{\pi}{4} \cos \theta\right)=\cos \left(\frac{\pi}{4} \tan \theta\right)$$, then $$\theta$$ is equal 24. If $$x=\sin \left(2 \tan ^{-1} 2\right), y=\cos \left(2 \tan ^{-1} 3\right)$$ and $$z=\sec \left(3 \tan ^{-1} 4\right)$$ 25. In $$\triangle A B C$$, medians $$A D$$ and $$B E$$ are drawn. If $$A D=4, \angle D A B=\frac{\pi}{6}$$ and $$\angle A B 26. In a $$\triangle A B C, 2 \Delta^2=\frac{a^2 b^2 c^2}{a^2+b^2+c^2}$$, then the triangle is 27. The sides of a triangle inscribed in a given circle subtend angles $$\alpha, \beta, \gamma$$ at the center. The minimum 28. The position vectors of the points $$A$$ and $$B$$ with respect to $$O$$ are $$2 \hat{\mathbf{i}}+2 \hat{\mathbf{j}}+\ha 29. Let $$\mathbf{u}=2 \hat{\mathbf{i}}+3 \hat{\mathbf{j}}+\hat{\mathbf{k}}, \mathbf{v}=-3 \hat{\mathbf{i}}+2 \hat{\mathbf{j 30. If the lines, $$\frac{x-3}{2}=\frac{y-2}{3}=\frac{z-1}{\lambda}$$ and $$\frac{x-2}{3}=\frac{y-3}{2}=\frac{z-2}{3}$$ are 31. If a = (1, 1, 0) and b = (1, 1, 1), then unit vector in the plane of a and b and perpendicular to a is 32. The line passing through $$(1,1,-1)$$ and parallel to the vector $$\hat{\mathbf{i}}+2 \hat{\mathbf{j}}-\hat{\mathbf{k}}$ 33. Let $$\mathbf{a}=\hat{\mathbf{i}}$$ and $$\mathbf{b}=\hat{\mathbf{j}}$$, the point of intersection of the lines $$\mathb 34. The mean deviation from the mean of the set
of observation $$-1,0,4$$ is 35. Let an angle of a triangle is 60$$^\circ$$. If the variance of the angles of the triangle is 1014$$^\circ$$, then the ot 36. One card is selected at random from 27 cards
numbered form 1 to 27. What is the
probability that the number on the card 37. Nine balls one drawn simultaneously from a bag containing 5 white and 7 black balls. The probability of drawing 3 white 38. The probabilities that $$A$$ and $$B$$ speak truth are $$\frac{4}{5}$$ and $$\frac{3}{4}$$ respectively. The probability 39. The mean and variance of a binomial variable
X are 2 and 1 respectively. The probability
that X takes values greater tha 40. For the random variable X with probability distribution is given by the table
.tg {border-collapse:collapse;border-spa 41. The locus of a point, which is at a distance of 4 units from $$(3,-2)$$ in $$x y$$-plane is 42. When the axes are rotated through an angle
45$$^\circ$$, the new coordinates of a point P are
(1, $$-$$1). The coordinat 43. Find the equation of a straight line passing through $$(-5,6)$$ and cutting off equal intercepts on the coordinate axes. 44. Line has slope $$m$$ and $$y$$-intercept 4 . The distance between the origin and the line is equal to 45. The equation of the base of an equilateral triangle is $$x+y=2$$ and one vertex is $$(2,-1)$$, then the length of the si 46. The equation of a straight line which passes through the point $$\left(a \cos ^3 \theta, a \sin ^3 \theta\right)$$ and p 47. The acute angle between lines $$6 x^2+11 x y-10 y^2=0$$ is 48. If the lines, joining the origin to the points of intersection of the curve $$2 x^2-2 x y+3 y^2+2 x-y-1=0$$ and the line 49. The equation of bisector of the angle between the lines represented by $$3 x^2-5 x y+4 y^2=0$$ is 50. If the bisectors of the pair of lines $$x^2-2 m x y-y^2=0$$ is represented by $$x^2-2 n x y-y^2=0$$, then 51. Find the equation of the circle which passes through origin and cuts off the intercepts $$-$$2 and 3 over the $$X$$ and 52. The angle between the pair of tangents drawn from $$(1,1)$$ to the circle $$x^2+y^2+4 x+4 y-1=0$$ is 53. If the circle $$x^2+y^2-4 x-8 y-5=0$$ intersects the line $$3 x-4 y-m=0$$ in two distinct points, then the number of int 54. Let $$C$$ be the circle center $$(0,0)$$ and radius 3 units. The equation of the locus of the mid-points of the chords o 55. The length of the common chord of the circles $$x^2+y^2+3x+5y+4=0$$ and $$x^2+y^2+5x+3y+4=0$$ is __________ units. 56. Find the equation of the circle which passes through the point $$(1,2)$$ and the points of intersection of the circles $ 57. The coordinates of the focus of the parabola described parametrically by $$x=5t^2+2$$ and $$y=10t+4$$ (where t is a para 58. If $$\tan \theta_1, \tan \theta_2=\frac{-a^2}{b^2}$$, then the chord joining 2 points $$\theta_1$$ and $$\theta_2$$ one 59. If one focus of a hyperbola is $$(3,0)$$, the equation of its directrix is $$4 x-3 y-3=0$$ and its eccentricity $$e=5 / 60. If the vertices of the triangles are (1, 2, 3), (2, 3, 1), (3, 1, 2) and if H, G, S and I respectively denote its orthoc 61. A(2, 3, 4), B(4, 5, 7), C(2, $$-$$6, 3) and D(4, $$-$$4, k) are four points. If the line AB is parallel to CD, then k is 62. If the direction cosines of two lines are $$\left( {{2 \over 3},{2 \over 3},{1 \over 3}} \right)$$ and $$\left( {{5 \ove 63. $$\mathop {\lim }\limits_{n \to \infty } {{n{{(2n + 1)}^2}} \over {(n + 2)({n^2} + 3n - 1)}}$$ is equal to 64. If the function $$f(x)$$, defined below, is continuous on the interval $$[0,8]$$, then $$f(x)=\left\{\begin{array}{cc}x^ 65. If $$f(x)$$, defined below, is continuous at $$x=4$$, then
$$f(x) = \left\{ {\matrix{
{{{x - 4} \over {|x - 4|}} + a} 66. If $$f(x)=2x^2+3x-5$$, then the value of $$f'(0)+3f'(-1)$$ is equal to 67. If $$y=\left(1+\frac{1}{x}\right)\left(1+\frac{2}{x}\right)\left(1+\frac{3}{x}\right) \ldots\left(1+\frac{n}{x}\right)$$ 68. $$\frac{d}{d x}\left\{\sin ^2\left(\cot ^{-1} \sqrt{\frac{1+x}{1-x}}\right)\right\}$$ is equal to 69. If $$y=\tan ^{-1}\left\{\frac{a x-b}{b x+a}\right\}$$, then $$y^{\prime}$$ is equal to 70. If $$y=4 x-6$$ is a tangent to the curve $$y^2=a x^4+b$$ at $$(3,6)$$, then the values of $$a$$ and $$b$$ are 71. Find the positive value of $$a$$ for which the equality $$2 \alpha+\beta=8$$ holds, where $$\alpha$$ and $$\beta$$ are t 72. If the radius of a sphere is measured as 9 cm
with an error of 0.03 cm, then find the
approximate error in calculating i 73. The diameter and altitude of a right circular
cone, at a certain instant, were found to be
10 cm and 20 cm respectively. 74. $$\int \frac{\sin \alpha}{\sqrt{1+\cos \alpha}} d \alpha$$ is equal to 75. If $$\int \frac{\cos 4 x+1}{\cot x-\tan x}=k \cos 4 x+C$$, then $$k$$ is equal to 76. If $$\int\left[\cos (x) \cdot \frac{d}{d x}(\operatorname{cosec}(x)] d x=f(x)+g(x)+c\right.$$ then $$f(x) \cdot g(x)$$ i 77. If $$\int \frac{(2 x+1)^6}{(3 x+2)^8} d x=P\left(\frac{2 x+1}{3 x+2}\right)^Q+R$$, then $$\frac{P}{Q}$$ is equal to
78. If $$\int_0^a {{{dx} \over {4 + {x^2}}} = {\pi \over 8}} $$, then the value of a is equal to 79. $$\int_1^2 {{{{x^3} - 1} \over {{x^2}}}} $$ is equal to 80. The solution of the differential equation $$2x\left(\frac{dy}{dx}\right)-y=4$$ represents a family of
Physics
1. The displacement of a particle starting from rest at $$t=0$$ is given by $$s=9 t^2-2 t^3$$. The time in seconds at which 2. The range of a projectile is 100 m. Its kinetic
energy will be maximum after covering a
distance of 3. Two cars A and B are moving with a velocity
of 30 km/h in the same direction. They are
separated by 10 km. The speed of 4. A book is lying on a table. What is the angle
between the normal reaction acting on the
book on the table and the weight 5. A boy throws a cricket ball from the
boundary to the wicket keeper. If the
frictional force due to air $$(f_a )$$ cannot 6. When a force F = 17 $$-$$ 2x + 6x$$^2$$N acts on a body of mass 2 kg and displaces it from x = 0 m to x = 8 m, the work 7. A rifle bullet loses $$\left(\frac{1}{25}\right)$$th of its velocity in passing through a plank. The least number of suc 8. A uniform chain has a mass m and length $$l$$.
It is held on a frictionless table with
one-sixth of its length hanging o 9. A sphere and a hollow cylinder without
slipping, roll down two separate inclined
planes A and B, respectively. They cove 10. Four spheres each of diameter $$2 a$$ and mass $$m$$ are placed in a way that their centers lie on the four corners of a 11. If an energy of 684 J is needed to increase the
speed of a flywheel from 180 rpm to
360 rpm, then find its moment of ine 12. A particle executing simple harmonic motion
along a straight line with an amplitude A,
attains maximum potential energy 13. The bob of a simple pendulum is a spherical
hollow ball filled with water. A plugged hole
near the bottom of the oscilla 14. The gravitational potential energy is maximum at 15. A geostationary satellite is taken to a new
orbit, such that its distance from centre of
the earth is doubled. Then, fin 16. A body of mass 10 kg is attached to a wire of 0.3 m length. The breaking stress is 4.8 $$\times$$ 10$$^7$$ Nm$$^{-2}$$. 17. A glass flask weighting 390 g, having internal
volume 500 cc just floats when half of it is
filled with water. Specific 18. Water does not wet an oily glass because 19. Boiling water is changing into steam. The specific heat of boiling water is 20. If the volume of a block of metal changes by $$0.12 \%$$ when heated through $$20^{\circ} \mathrm{C}$$, then find its co 21. Isothermal process is the graph between 22. For a monoatomic ideal gas is following the
cyclic process ABCA shown in the U versus p
plot, identify the incorrect opt 23. The pressure of a gas is proportional to 24. A string fixed at both ends vibrate in 5 loops
as shown in the figure. The total number of
nodes and anti-nodes respecti 25. The position of the direct image obtained at
O, when a monochromatic beam of light is
passed through a plane transmissio 26. What is the electric flux for Gaussian surface $$A$$ that encloses the charged particles in free space? [Given, $$q_1=-1 27. Two charges 8 $$\mu$$C each are placed at the corners A and B of an equilateral triangle of side 0.2 m in air. The elect 28. A 60 $$\mu$$F parallel plate capacitor whose plates
are separated by 6 mm is charged to 250 V,
and then the charging sou 29. Five current carrying conductors meet at a
point P. What is the magnitude and direction
of the current in the fifth cond 30. A wire of length $$L$$ metre carrying a current $$I$$ ampere is bent in the form of a circle. Magnitude of its magnetic 31. What is the net force on the square coil?
32. A paramagnetic sample showing a net magnetisation of $$0.8 \mathrm{~A} \mathrm{~m}^{-1}$$, when placed in an external ma 33. The induced emf cannot be produced by 34. Assertion (A) When plane of coil is perpendicular to magnetic field, magnetic flux linked with the coil is minimum, but 35. A 20 V AC is applied to a circuit consisting of
a resistor and a coil with negligible
resistance. If the voltage across 36. The electric and the magnetic fields associated with an electromagnetic wave propagating along the $$z$$-axis, can be re 37. The graph between the maximum speed $$(v_{max})$$ of a photoelectron and frequency $$(\nu)$$ of the incident radiation, 38. The angular momentum of the orbital
electron is integral multiple of 39. Which of the following values is the correct order of nuclear density? 40. The truth table given below corresponds to logic gate.
1
AP EAPCET 2021 - 20th August Morning Shift
MCQ (Single Correct Answer)
+1
-0
Two cars A and B are moving with a velocity of 30 km/h in the same direction. They are separated by 10 km. The speed of another car C moving in the opposite direction, if it meets these two cars at an interval of eight minutes is
A
45 km/h
B
40 km/h
C
15 km/h
D
30 km/h
2
AP EAPCET 2021 - 20th August Morning Shift
MCQ (Single Correct Answer)
+1
-0
A book is lying on a table. What is the angle between the normal reaction acting on the book on the table and the weight of the book?
A
0$$^\circ$$
B
45$$^\circ$$
C
90$$^\circ$$
D
180$$^\circ$$
3
AP EAPCET 2021 - 20th August Morning Shift
MCQ (Single Correct Answer)
+1
-0
A boy throws a cricket ball from the boundary to the wicket keeper. If the frictional force due to air $$(f_a )$$ cannot be ignored, the forces acting on the ball at the position X are represented by

A

B
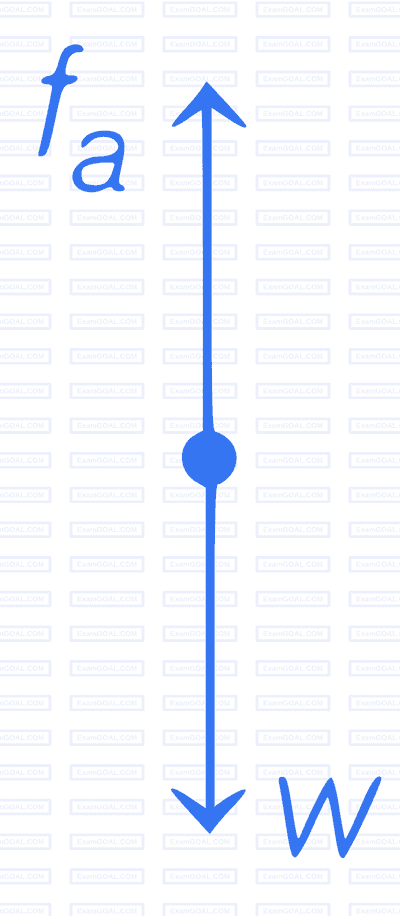
C
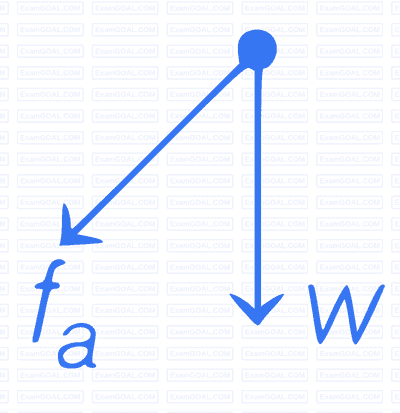
D
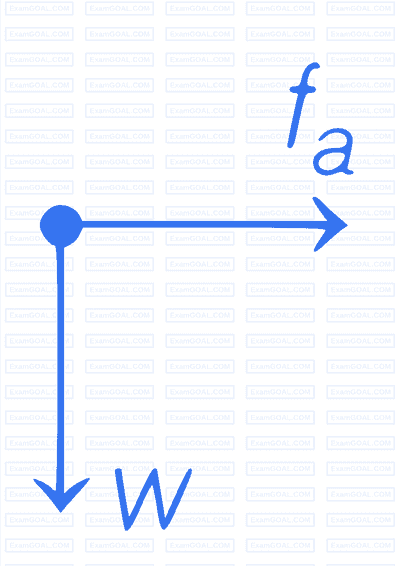
4
AP EAPCET 2021 - 20th August Morning Shift
MCQ (Single Correct Answer)
+1
-0
When a force F = 17 $$-$$ 2x + 6x$$^2$$N acts on a body of mass 2 kg and displaces it from x = 0 m to x = 8 m, the work done is
A
1096 J
B
270 J
C
35 J
D
135 J
Paper analysis
Total Questions
Chemistry
40
Mathematics
80
Physics
40
More papers of AP EAPCET
AP EAPCET 2024 - 23th May Morning Shift
AP EAPCET 2024 - 22th May Evening Shift
AP EAPCET 2024 - 22th May Morning Shift
AP EAPCET 2024 - 21th May Evening Shift
AP EAPCET 2024 - 21th May Morning Shift
AP EAPCET 2024 - 20th May Evening Shift
AP EAPCET 2024 - 20th May Morning Shift
AP EAPCET 2024 - 19th May Evening Shift
AP EAPCET 2024 - 18th May Morning Shift
AP EAPCET 2022 - 5th July Morning Shift
AP EAPCET 2022 - 4th July Evening Shift
AP EAPCET 2022 - 4th July Morning Shift
AP EAPCET 2021 - 20th August Morning Shift
AP EAPCET 2021 - 19th August Evening Shift
AP EAPCET 2021 - 19th August Morning Shift
AP EAPCET
Papers
2024
2022