AP EAPCET 2024 - 21th May Morning Shift

Paper was held on Tue, May 21, 2024 3:30 AM

View Questions

Chemistry

The energy of third orbit of $\mathrm{Li}^{2+}$ ion (in J ) is

The number of $d$ electrons in Fe is equal to which of the following?

(i) Total number of ' $s$ ' electrons of Mg .

(ii) Total number of ' $p$ ' electrons of Cl .

(iii) Total number of ' $p$ ' electrons of Ne .

The correct option is

The correct order of atomic radii of given element is

Which of the following orders are correct regarding their covalent character?

(i) $\mathrm{KF}<\mathrm{KI}$

(ii) $\mathrm{LiF}<\mathrm{KF}$

(iii) $\mathrm{SnCl}_2<\mathrm{SnCl}_4$

(iv) $\mathrm{NaCl}<\mathrm{CuCl}$

The correct option is

$$ \text { Observe the following sets. } $$

$$ \begin{array}{lll} \hline \text { Order } & \text { Property } \\ \hline \text { (i) } \mathrm{NH}_3>\mathrm{H}_2 \mathrm{O}>\mathrm{SO}_2 & \text { Bond angle } \\ \hline \text { (ii) } \mathrm{H}_2 \mathrm{O}>\mathrm{NH}_3>\mathrm{H}_2 \mathrm{~S} & \text { Dipole moment } \\ \text { (iii) } \mathrm{N}_2>\mathrm{O}_2>\mathrm{H}_2 & \text { Bond enthalpy } \\ \hline \text { (iv) } \mathrm{NO}^{+}>\mathrm{O}_2>\mathrm{O}_2^{2-} & \text { Bond order } \\ \hline \end{array} $$

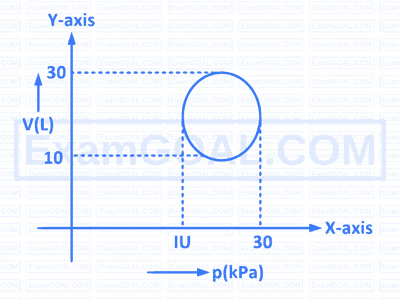
The RMS velocity ( $u_{\mathrm{rms}}$ ) of one mole of an ideal gas was measured at different temperatures and the following graph is obtained. What is the slope $(m)$ of straight line ?

$$ \begin{aligned} & \left(X \text {-axis }=T(\mathrm{~K}): Y \text {-axis }=\left(u_{\mathrm{rms}}\right)^2: M=\right.\text { molar mass : } \\ & R=\text { gas constant } \end{aligned} $$

AP EAPCET 2024 - 21th May Morning Shift Chemistry - States of Matter Question 3 English

Two statements are given below.

Statement I : Viscosity of liquid decreases with increase in temperature.

Statement II : The units of viscosity coefficient are pascal.

The correct answer is

0.1 mole of potassium permanganate was heated at $300^{\circ} \mathrm{C}$. What is weight (ing) of the residue? $$ (\mathrm{Mn}=55 \mathrm{u}, \mathrm{~K}=39 \mathrm{u}, \mathrm{O}=16 \mathrm{u}) $$

Identify the correct statements from the following. I. $\Delta_r G$ is zero for $A \rightleftharpoons B$ reaction. II. The entropy of pure crystalline solids approaches. zero as the temperature approaches absolute zero. III. $\Delta U$ of a reaction can be determined using bomb calorimeter.

Observe the following reactions.

$$ \begin{array}{ll} A B(g)+25 \mathrm{H}_2 \mathrm{O}(l) \longrightarrow\left(25 \mathrm{H}_2 \mathrm{O}\right) A B ; & \Delta H=x \mathrm{~kJ} \mathrm{~mol}^{-1} \\ A B(g)+50 \mathrm{H}_2 \mathrm{O}(l) \longrightarrow\left(50 \mathrm{H}_2 \mathrm{O}\right) A B ; & \Delta H=y \mathrm{~kJ} \mathrm{~mol}^{-1} \end{array} $$

$K_C$ for the reaction,
$A_2(g) \stackrel{T(\mathrm{~K})}{\rightleftharpoons} B_2(\mathrm{~g})$
is 39.0. In a closed one litre flask, one mole of $A_2(g)$ was heated to $T(\mathrm{~K})$. What are the concentrations of $A_2(g)$ and $B_2(g)$ (in mol L ${ }^{-1}$ ) respectively at equilibrium?

At $T(\mathrm{~K})$, the solubility product of $A X$ is $10^{-10}$. What is the molar solubility of $A X$ in 0.1 MHX ?

The equation that represents 'coal gasification' is

As per standard reduction potential values, which is the strongest reducing agent among the given elements?

A Lewis acid ' $X$ ' reacts with $\mathrm{LiAlH}_4$ in ether medium to give a highly toxic gas. ${ }^{\prime} Y^{\prime}$, ' $Y$ ' when heated with $\mathrm{NH}_3$ gives a compound known as inorganic benzene. ' $Y^{\prime}$ burns in oxygen and gives $\mathrm{H}_2 \mathrm{O}$ and ' $Z$ ', ' $Z$ ' is

The method for preparation of water gas is

The BOD values for pure water and highly polluted water are respectively.

A mixture of ethyl iodide and $n$-propyl iodide is subjected to Wurtz reaction. The hydrocarbon which will not be formed is

Which of the following alkenes does not undergo anti Markownikoff addition of HBr ?

What are the variables in the graph of powder diffraction pattern of a crystalline solid?

100 mL of $\frac{M}{10} \mathrm{Ca}\left(\mathrm{NO}_3\right)_2$ and 200 mL of $\frac{M}{10} \mathrm{KNO}_3$ solutions are mixed. What is the normality of resulted solution with respect to $\mathrm{NO}_3^{-}$?

A solution was prepared by dissolving 0.1 mole of a non- volatile solute in 0.9 moles of water. What is the relative lowering of vapour pressure of solution?

The standard free energy change $\left(\Delta G^{\circ}\right)$ for the following reaction (in kJ ) at $25^{\circ} \mathrm{C}$ is $$ 3 \mathrm{Ca}(s)+2 \mathrm{Au}^{3+}(a q, 1 M) \longrightarrow 3 \mathrm{Ca}^{2+}(a q, 1 M)+2 \mathrm{Au}(s) $$

The rate constant of a first order reaction is $3.46 \times 10^{-2} \mathrm{~s}^{-1}$ at 298 K . What is the rate constant of the reaction at 350 K if its activation energy is $50.1 \mathrm{~kJ} \mathrm{~mol}^{-1}$, $\left(R=8.314 \mathrm{JK}^{-1} \mathrm{~mol}^{-1}\right)(\log 2=0.3010)$

The correct statement regarding chemisorption is

Which of the following is incorrectly matched?

Improve silver ore $+\mathrm{CN}^{-}+\mathrm{H}_2 \mathrm{O} \xrightarrow{\mathrm{O}_2}[\mathrm{X}]^{-}+\mathrm{OH}^{-}$

$$ [X]^{-}+\mathrm{Zn} \longrightarrow[Y]^{2-}+\mathrm{Ag} \text { (pure) } $$

The co-ordination numbers of the metals in $[X]$. [ $Y$ ] are respectively

In the reaction of sulphur with concentrated sulphuric acid, the oxidised product is $X$ and reduced product is $Y, X$ and $Y$ are respectively.

Which of the following lanthanoids have [Xe] $4 f^x 5 d^1 6 s^2$ configuration in their ground state. $$ (X=1-14) $$

How many of the following ligands are stronger than

$$ \begin{aligned} & \mathrm{H}_2 \mathrm{O} \text { ? } \\ & \mathrm{S}^{2-}, \mathrm{Br}^{-}, \mathrm{C}_2 \mathrm{O}_4^{2-}, \mathrm{CN}^{-} \text {, en, } \mathrm{NH}_3, \mathrm{CO}, \mathrm{OH}^{-} \\ & \begin{array}{ll} \text { } & \text { } \end{array} \end{aligned} $$

$$ \text { The common monomer for both terylene and glyptal is } $$

Which of the following structure of proteins represellis its constitution?

Carrot and curd are sources for the vitamins repectively.

$$ \text { Match the following. } $$

List I (Drug)		List I (Use)
A	Veronal	i	Antihistamine
B	Morphine	ii	Hypnotic
C	Seldane	iii	Analgesic
		iv	$$ \text { Antidepressant } $$

$$ \text { Correct answer is } $$

The major products $X$ and $Y$ respectively from the following reactions are

AP EAPCET 2024 - 21th May Morning Shift Chemistry - Hydrogen and It's Compounds Question 1 English

An isomer of $\mathrm{C}_5 \mathrm{H}_{12}$ on reaction with $\mathrm{Br}_2$ /light gave only one isomer $\mathrm{C}_5 \mathrm{H}_{11} \mathrm{Br}(X)$. Reaction of $X$ with $\mathrm{AgNO}_2$ gave $Y$ as major product. What is $Y$ ?

What are the major products $X$ and $Y$ respectively in the following reactions?

$$ \begin{aligned} & \left(\mathrm{CH}_3\right)_3 \mathrm{CONa}+\mathrm{CH}_3 \mathrm{CH}_2 \mathrm{Br} \longrightarrow X \\ & \left(\mathrm{CH}_3\right)_3 \mathrm{CBr}+\mathrm{CH}_3 \mathrm{CH}_2 \mathrm{ONa} \longrightarrow Y \end{aligned} $$

Match the following reagents with the products obtained when they reacted with a ketone.

List I		List II
A.	$$ \mathrm{C}_6 \mathrm{H}_5 \mathrm{NHNH}_2 $$	i.	Schiff base
B.	$$ \mathrm{NH}_2 \mathrm{OH} $$	ii.	Hydrazone
C.	$$ \mathrm{C}_6 \mathrm{H}_5 \mathrm{NH}_2 $$	iii.	Oxime
		iv.	Phenyl hydrazone

$$ \text { Correct answer is } $$

What are $X$ and $Y$ respectively in the following reactions?

AP EAPCET 2024 - 21th May Morning Shift Chemistry - Carboxylic Acids and Its Derivatives Question 2 English

Arrange the following in decreasing order of their basicity.

AP EAPCET 2024 - 21th May Morning Shift Chemistry - General Organic Chemistry Question 2 English

Mathematics

The domain of the real valued function $f(x)$ $=\log _2 \log _3 \log _5\left(x^2-5 x+11\right)$ is

The range of the real valued function $f(x)=\left(\frac{x^2+2 x-15}{2 x^2+13 x+15}\right)$ is

$\frac{1}{1 \cdot 5}+\frac{1}{5 \cdot 9}+\frac{1}{9 \cdot 13}+\ldots$. upto $n$ terms $=$

If $A=\left|\begin{array}{lll}2 & 3 & 4 \\ 1 & k & 2 \\ 4 & 1 & 5\end{array}\right|$ is singular matrix, then the quadratic equation having the roots $k$ an $\frac{1}{k}$ is

Let $A$ be a $4 \times 4$ matrix and $P$ be is adjoint matrix, If $|P|=\left|\frac{A}{2}\right|$ then $\left|A^{-1}\right|$

The system $x+2 y+3 z=4,4 x+5 y+3 z=5,3 x+4 y+3 z=\lambda$ is consistent and $3 \lambda=n+100$, then $n=$

The complex conjugate of $(4-3 i)(2+3 i)(1+4 i)$ is.

If the amplitude of $(z-2)$ is $\frac{\pi}{2}$, then the locus of $z$ is

If $\omega$ is the cube root of unity, $$ \frac{a+b \omega+c \omega^2}{c+a \omega+b \omega^2}+\frac{a+b \omega+c \omega^2}{b+c \omega+b \omega^2}= $$

Roots of the equation $a(b-c) x^2+b(c-a) x+c(a-b)=0$ are

If $(3+i)$ is a root of $x^2+a x+b=0$, then $a=$

The algebraic equation of degree 4 whose roots are translate of the roots of the equation. $x^4+5 x^3+6 x^2+7 x+9=0$ by -1 is

If the roots of the equation $4 x^3-12 x^2+11 x+m=0$ are in arithmetic progression, then $m=$

The number of 5 -digit odd numbers greater than 40000 that can be formed by using 3,4,5,6,7,0 so that at least one of its digit must be repeated is

The number of ways in which 3 men and 3 women can be arranged in a row of 6 seats, such that the first and last seats must be filled by men is

If a committee of 10 members is to be formed from 8 men and 6 women, then the number of different possible committees in which the men are in majority is

If the eleventh term in the binomial expansion of $(x+a)^{15}$ is the geometric mean of the eighth and twelfth terms, then the greatest term in the expansion is

The sum of the rational terms in the binomial expansion of $\left(\sqrt{2}+3^{1 / 5}\right)^{10}$ is

If $\frac{1}{(3 x+1)(x-2)}=\frac{A}{3 x+1}+\frac{B}{x-2}$ and $\frac{x+1}{(3 x+1)(x-2)}=\frac{C}{3 x+1}+\frac{D}{x-2}$, then

If the period of the function $f(x)=\frac{\tan 5 x \cos 3 x}{\sin 6 x}$ is $\alpha$, then $f\left(\frac{\alpha}{8}\right)=$

If $\sin x+\sin y=\alpha, \cos x+\cos y+\beta$, then $\operatorname{cosec}(x+y)=$

If $P+Q+P=\frac{\pi}{4}$, then $\cos \left(\frac{\pi}{8}-P\right)+\cos \left(\frac{\pi}{8}-Q\right)+\cos$ $\left(\frac{\pi}{8}-R\right)=$

For $a \in R-\{0\}$, if $a \cos x+a \sin x+a=2 k+1$ has a solution, then $k$ lies in the interval

If the general solution .set of $\sin x+3 \sin 3 x+\sin 5 x=1$ is $S$, then $\{\sin \alpha / \alpha \in S\}=$

If $\theta$ is an acute angle, $\cosh x=K$ and $\sinh x=\tan \theta$, then $\sin \theta=$

In a $\Delta$ if the angles are in the ratio $3: 2: 1$, then the ratio of its sides is

In a $\triangle A B C$, if $B C=5, C A=6$ and $A B=7$, then the length of the median drawn from $B$ onto $A C$ is

In $\triangle A B C$, if $A B: B C: C A=6: 4: 5$, then $R: r$ is equal to

$\mathbf{a}=\alpha \hat{\mathbf{i}}+\beta \hat{\mathbf{j}}+3 \hat{\mathbf{k}}, \quad \mathbf{b}=\hat{\mathbf{j}}+2 \hat{\mathbf{k}}$ and $\mathbf{c}=3 \hat{\mathbf{i}}+2 \hat{\mathbf{j}}+\hat{\mathbf{k}}$ ar linearly dependent vectors and magnitude of $ \alpha $$ \sqrt{14} $${\text {}}{ }^{}$ If $\alpha, \beta$ are integers, then $\alpha+\beta=$

$\mathbf{c}$ is a vector along the bisector of the internal angle between the vectors $\mathbf{a}=4 \hat{\mathbf{i}}+7 \hat{\mathbf{j}}-4 \hat{\mathbf{k}}$ and $\mathbf{b}=12 \hat{\mathbf{i}}-3 \hat{\mathbf{j}}+4 \hat{\mathbf{k}}$. If the magnitude of $\mathbf{c}$ is $3 \sqrt{13}$, then c=

$\mathbf{a}=\hat{\mathbf{i}}-\hat{\mathbf{j}}+\hat{\mathbf{k}}, \mathbf{b}=2 \hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}}$ are two vectors and $\mathbf{c}$ is a unit vectors lying in the plane of $\mathbf{a}$ and $\mathbf{b}$. If $\mathbf{c}$ is perpendicular to $\mathbf{b}$, then $\mathbf{c}(\hat{\mathbf{i}}+\hat{\mathbf{j}}+2 \hat{\mathbf{k}})=$

$A(1,2,1), B(2,3,2), C(3,1,3)$ and $D(2,1,3)$ are the vertices of a tetrahedron. If $\theta$ is the angle between the faces $A B C$ and $A B D$, then $\cos \theta=$

If $\mathbf{a}=\hat{\mathbf{i}}-\hat{\mathbf{j}}+\hat{\mathbf{k}}, \mathbf{b}=\hat{\mathbf{i}}+\hat{\mathbf{j}}-2 \hat{\mathbf{k}}, \mathbf{c}=2 \hat{\mathbf{i}}-3 \hat{\mathbf{j}}-\hat{\mathbf{k}}$. $\mathbf{d}=2 \hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}}$ are four vector, then $(\mathbf{a} \times \mathbf{c}) \times(\mathbf{b} \times \mathbf{d})=$

Mean deviation about the mean for the following data is

$$ \begin{array}{llllll} \hline \text { Class Interval } & 0-6 & 6-12 & 12-18 & 18-24 & 24-30 \\ \hline \text { Frequency } & 1 & \,2 & \,3 & \,2 & \,1 \\ \hline \end{array} $$

If 12 dice are thrown at a time, then the probability that a multiple of 3 does not appear on any dice is

If a number is drawn at random from the set $\{1,3,5,7, \ldots . .59\}$, then the probability that it lies in the interval in which the function $f(x)=x^3-16 x^2+20 x-5$ is stricly decreasing is

In a class consisting of 40 boys and 30 girls. $30 \%$ of the boy and $40 \%$ of the girls are good at Mathematics. If a student selected at random from that class is found to be a girl, then the probability that she is not good at Mathematics is

A basket contains 12 apples in which 3 are rotten. If 3 apples are drawn at random simultaneously from it, then the probability of getting atmost one rotten apple is

7 coins are tossed simultaneously and the number of heads turned up is denoted by random variable $X$. If $\mu$ is the mean and $\sigma^2$ is the variance of $X$, then $\frac{\mu \sigma^2}{P(X=3)}=$

A manufacturing company noticed that $1 \%$ of its products are defective. If a dealer order for 300 items from this company, then the probability that the number of defective items is atmost one is

$P$ is a variable point such that the distance of $P$ from $A$ $(4,0)$ is twice the distance of $P$ from $B(-4,0)$. If the line $3 y-3 x-20=0$ intersects the locus of $P$ at the points $C$ and $D$, then the distance between $C$ and $D$ is

When the origin is shifted to $(h, k)$ by translation of axes, the transformed equation of $x^2+2 x+2 y-7=0$ does not contain $x$ term and constant term. Then, $(2 h+k)=$

Let $\alpha \in R$. If the line $(\alpha+1) x+\alpha y+\alpha=1$ passes through a fixed point $(h, k)$ for all $\alpha$, then $h^2+k^2=$

If $(\alpha, \beta)$ is the orthocentre of the triangle with the vertices $(2,2),(5,1),(4,4)$, then $\alpha+\beta=$

The area of the triangle formed by the lines represented by $3 x+y+15=0$ and $3 x^2+12 x y-13 y^2=0$ is

If all chords of the curve $2 x^2-y^2+3 x+2 y=0$, which subtend a right angle at the origin always passing through the point $(\alpha, \beta)$, then $(\alpha, \beta)=$

$2 x-3 y+1=0$ and $4 x-5 y-1=0$ are the equations of two diameters of the circle $S \equiv x^2+y^2+2 g x+2 f y-11=0 . Q$ and $R$ are the points of contact of the tangents drawn from the point $P(-2,-2)$ to this circle. If $C$ is the centre of the circle $S=0$, then the area (in square units ) of the quadrilateral $P Q C R$ is

If the inverse point of the point $(-1,1)$ with respect to the circle $x^2+y^2-2 x+2 y-1=0$ is $(p, q)$, then $p^2+q^2=$

If $(a, b)$ is the mid-point of the chord $2 x-y+3=0$ of the circle $x^2+y^2+6 x-4 y+4=0$, then $2 a+3 b=$

If a direct common tangent drawn to the circle $x^2+y^2-6 x+4 y+9=0$ and $x^2+y^2+2 x-2 y+1=0$ touches the circles at $A$ and $B$, then $A B=$

The radius of the circle which cuts the circles $x^2+y^2-4 x-4 y+7=0, x^2+y^2+4 x-4 y+6=0$ and $x^2+y^2+4 x+4 y+5=0$ orthogonally is

The equation of the normal drawn to the parabola $y^2=6 x$ at the point $(24,12)$ is

If $A_1, A_2, A_3$ are the areas of ellipse $x^2+4 y^2-4=0$ its director circle and auxiliary circle respectively, then $A_2+A_3-A_1=$

The equation of the pair of asymptotes of the hyperbola $4 x^2-9 y^2-24 x-36 y-36=0$ is

The equation of one of the tangents drawn from the point $(0,1)$ to the hyperbola $45 x^2-4 y^2=5$ is

Consider the tetrahedron with the vertices $A(3,2,4)$, $B\left(x_1, y_1, 0\right), C\left(x_2, y_2, 0\right)$ and $D\left(x_3, y_3, 0\right)$.If the $\triangle B C D$ is formed by the lines $y=x, x+y=6$ and $y=1$, then the centroid of the tetrahedron is

If $P(2, \beta, \alpha)$ lies on the plane $x+2 y-z-2=0$ and $Q(\alpha,-1, \beta)$ lies on the plane $2 x-y+3 z+6=0$, then the direction cosines of the $P Q$ are

Let $\pi$ be the plane that passes through the point $(-2,1,-1)$ and parallel to the plane $2 x-y+2 z=0$. Then the foot of perpendicular drawn from the point $(1,2,1)$ to the plane $\pi$ is

If $f(x)=\frac{5 x \cdot \operatorname{cosec}(\sqrt{x})-1}{(x-2) \operatorname{cosec}(\sqrt{x})}$, then $\lim \limits_{x \rightarrow \infty} f\left(x^2\right)=$

$\lim \limits_{x \rightarrow 2} \frac{\sqrt{1+4 x}-\sqrt{3+3 x}}{x^3-8}=$

If $$ \lim _{x \rightarrow \infty} \frac{(\sqrt{2 x+1}+\sqrt{2 x-1})^8+(\sqrt{2 x+1}-\sqrt{2 x-1})^8\left(P x^4-16\right)}{\left(x+\sqrt{x^2-2}\right)^8+\left(x-\sqrt{x^2-2}\right)^8}=1 $$ then $P=$

The rate of change of $x^{\sin x}$ with respect to $(\sin x)^x$ is

If $y=\frac{\alpha x+\beta}{\gamma \alpha+\delta}$, then $2 y_1 y_3=$

Which one of the following is false ?

The point which lies on the tangent drawn to the curve $x^4 e^y+2 \sqrt{y+1}=3$ at the point $(1,0)$ is

If $f(x)=x^x$, then the interval in which $f(x)$ decrease is

If the Rolle's theorem is applicable for the function $f(x)$ defined by $f(x)=x^3+P x-12$ on $[0,1]$ then the value of $C$ of the Rolle's theorem is

The number of all the value of $x$ for which the function $f(x)=\sin x+\frac{1-\tan ^2 x}{1+\tan ^2 x}$ attains it maximum value on [ $0.2 \pi$ ] is

If $x \in\left[2 n \pi-\frac{\pi}{4}, 2 n \pi+\frac{3 \pi}{4}\right]$ and $n \in Z$, then $\int \sqrt{1-\sin 2 x} d x=$

$\int e^x\left(\frac{x+2}{x+4}\right)^2 d x=$

If $\int \frac{1}{1-\cos x} d x=\tan \left(\frac{x}{\alpha}+\beta\right)+c$, then one of the values of $\frac{\pi \alpha}{4}-\beta$ is

If $729 \int_1^3 \frac{1}{x^3\left(x^2+9\right)^2} d x=a+\log b$, then $(a-b)=$

If $n \geq 2$ is a natural number and $0<\theta<\frac{\pi}{2}$, then $\int \frac{\left(\cos ^n \theta-\cos \theta\right)^{1 / n}}{\cos ^{n+1} \theta} \sin \theta d \theta=$

$\lim \limits_{n \rightarrow \infty} \frac{1^{17}+2^{77}+\ldots+n^{77}}{n^{78}}=$

$$ \text { If } f(x)=\left\{\begin{array}{cc} \frac{6 x^2+1}{4 x^3+2 x+3} & , 0 < x < 1 \\ x^2+1 & , 1 \leq x < 2 \end{array} \text {, then } \int_0^2 f(x) d x=\right. $$

If $\int_1^n[x] d x=120$, then $n=$

The area of the region under the curve $y=|\sin -\cos x|$, $0 \leq x \leq \frac{n}{2}$ and above $X$-axis, is (in sq units)

The differential equation formed by eliminating $a$ and $b$ from the equation $y=a e^{2 x}+b x e^{2 x}$ is

If $y=a^3 e^{y^2 x+c}$ is the general solution of a differential equation, where $a$ and $c$ are arbitrary constants and $b$ is fixed constant, then the order of differential equation is

The solution of differential equation $\left(x+2 y^3\right) \frac{d y}{d x}=y$ ls