In the Young's double slit experiment a monochromatic source of wavelength $$\lambda$$ is used. The intensity of light passing through each slit is $$I_0$$. The intensity of light reaching the screen $$S_C$$ at a point $$P$$, a distance $$x$$ from $$O$$ is given by (Take, $$d<< D$$)

The work-function of a metal is 1 eV. Light of wavelength $$3000 \mathop A\limits^o$$ is incident on this metal surface. The velocity of emitted photoelectrons will be
A proton moving with a momentum $$p_1$$ has a kinetic energy $$1 / 8$$th of its rest mass-energy. Another light photon having energy equal to the kinetic energy of the possesses a momentum $$p_2$$. Then, the ratio $$\frac{p_1-p_2}{p_1}$$ is equal to
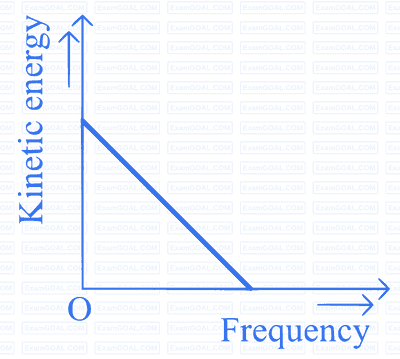
According to Einstein's photoelectric equation to the graph between kinetic energy of photoelectrons ejected and the frequency of incident radiation is