A resistance $$R$$ and inductance $$L$$ and a capacitor $$C$$ all are connected in series with an $$\mathrm{AC}$$ supply. The resistance of $$R$$ is $$24 \mathrm{~ohm}$$ and for a given frequency, the inductive reactance of $$L$$ is 36 ohm and capacitive reactance of $$C$$ is $$24 \mathrm{~ohm}$$. If the current in the circuit is $$5 \mathrm{~amp}$$. Find the potential difference across $$R, L$$ and $$C$$.
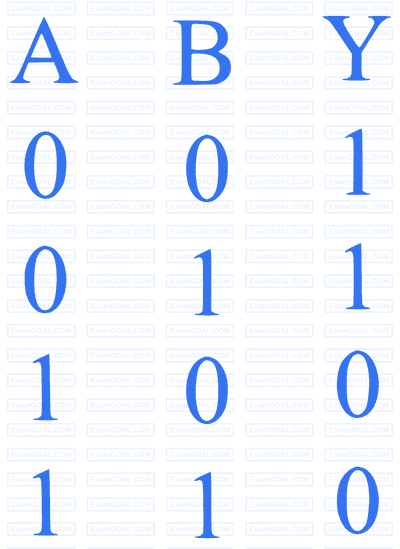
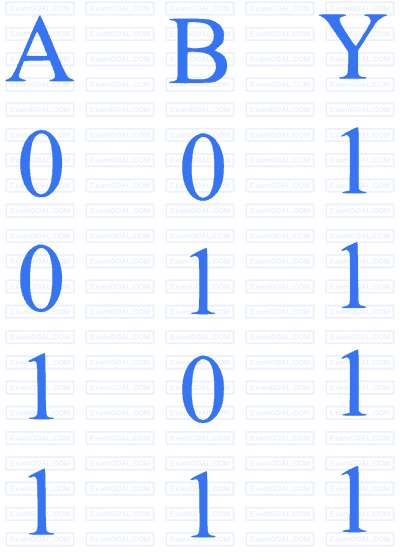
Truth table for system of four NAND gate and one NOT gate as shown in figure is.



A boat crosses a river from part $$A$$ to part $$B$$, which are just on the opposite side. The speed of the water. $$v_\omega$$ and the of boat is $$v_B$$ relative to still water. Assume $$v_B=\sqrt{2} v_\omega$$. What is the time taken by the boat, if it has to cross the river directly on the $$A B$$ line ($$D=$$ width of the river) ?
Two rings of radius $$R$$ and $$n R$$ made of same material have the ratio of moment of inertia about an axis passing through centre is $$1: 64$$. The value of $$n$$ is