Chemistry
1. In a set of reactions, m-bromobenzoic acid gives a product D. Identify the product D:
2. A volume of 50.00 mL of a weak acid of unknown concentration is titrated with 0.10 M solution of NaOH. The equivalence p 3. 1-phenyl-1, 3-dibromopropane on treatment with alc. KOH givens diastereomeric mixture in which compound (A) is major pro 4. Which of the following statement is incorrect? 5. Which of the following is not a mixed pair of oxides? 6. A solution of copper sulphate is electrolyzed between copper electrodes by a current of 10.0A passing for one hour. Whic 7.
Identify structure of compound (D). 8. Which of the following statement is correct? 9. Which of the following statements on critical constants of gases are correct?
I. Larger the Tc/pc value of a gas, larger 10. In the given reaction,
Product (D) is 11.
(B) is identified by the characteristic colour of the flame. (A) and (B) are respectively : 12. How many mL of perhydrol is required to produce sufficient oxygen which can be used to completely convert 2 L of SO2 gas 13. At the top of a mountain the thermometer reads 0$$^\circ$$C and the barometer reads 710 mm Hg. At the bottom of the moun 14. Match the following columns:
.tg {border-collapse:collapse;border-spacing:0;}
.tg td{border-color:black;border-style:s 15. Most acidic hydrogen is present in 16. The process of 'eutrophication' is due to the 17. Ionisation energy of H-atom is 13.6 eV. The wavelengths of the spectral line emitted when an electron in Be3+ comes them 18. The enthalpies of combustion of carbon and carbon monoxide in excess of oxygen at 298 K and constant pressure are $$-$$3 19. If the quantum number I could have the value 'n' also then, Sc(21) would have electronic configuration as (other rules s 20. 1.1 mole of A mixed with 2.2 moles of B and the mixture is kept in a 1 L flask and the equilibrium, A + 2B $$\rightlefth 21. Choose the correct chemical reaction among the following: 22. Which of the following reactions represents disproportionation? 23.
Compound C is 24. On the basis of Ellingham diagram, which of the following is not correct? 25. Out of the options listed below, which one cannot be explained by adsorption? 26. Identify 'A' and 'B' in the following reaction
27. An element occurs in two crystalline forms $$\alpha$$ and $$\beta$$. The $$\alpha$$-form has an fcc with $$a = 3.68\,\ma 28. The molar heat of vaporisation of water at 100$$^\circ$$C is 40.585 kJ/mol. The temperature at which a solution containi 29. An organic base C8H11N(X) reacts with nitrous acid at 0$$^\circ$$C to give a clear solution. Heating the solution with K 30. The final product of the following reaction is/are
31. The correct order of pseudohalide, polyhalide and interhalogen is 32. An inorganic halide (A) reacts with water to form two acids (B) and (C). (A) also reacts with NaOH to form two salts (D) 33. Following statements regarding the periodic trends of chemical reactivity to the alkali metals and the halogens are give 34. According to IUPAC nomenclature sodium nitroprusside is named as 35. A(g) $$\to$$ P(g) + Q(g) + R(g),
Follow first order kinetics with a half-life of 69.3 s at 500$$^\circ$$C. Starting from 36. Which of the following gives paracetamol on acetylation? 37. In qualitative analysis when H2S is passed through an aqueous solution of salt acidified with dilute HCl, a black ppt. i 38. Titration of 0.1467 g of primary standard Na2C2O4 required 28.85 mL of KMnO4 solution. Calculate the molar concentration 39. Identify the incorrect statement regarding the complex [Cr(NH3)6]Cl3. 40. Sulphuric acid is a dibasic acid. It ionises in two stages and hence has two dissociation constants Ka1 and Ka2. Which o
English Proficiency
1. Find out which part of a sentence has an error. If there is no error mark part (d) as your answer.
The road a. / to famo 2. Find out which part of a sentence has an error. If there is no error mark part (d) as your answer. 3. Find out which part of a sentence has an error. If there is no error mark part (d) as your answer. 4. Her trekking was met .................. obstacles. 5. She has not got .................... the shock of losing her father. 6. Yuvraj Singh is suffering from a BENIGN cancer. 7. He is a NOTED figure of film industry. 8. SAGACIOUS decisions taken at right time in one's career has long effects. 9. The actor got 'PEEVISH' on asking personal questions. 10. The engineer 'ROUGHED OUT' his ideas on a piece of paper while he talked. 11. Which one of the following statements is correct? 12. What should be given priority attention? 13. Which one of the following statements is not correct? 14. What would force the planning process to undergo a change? 15. Which one of the following is implied by the expression 'momentous trends'?
Logical Reasoning
1. Find out the wrong number.
2, 6, 12, 72, 865, 62208 2. Each of P, Q, T, A and B has different heights. T is taller than P and B but shorter than A and Q. P is not the shortest 3. Identify the missing part of the question figure and select it from given answer figures.
4. Select the related word from the given alternatives.
Mechanic : Spanner : : Carpenter : ? 5. How many rectangles are there in the following figure?
6. In the following question find the odd letters/group from the given alternatives. 7. Find out which of the answer figures (a), (b), (c) and (d) completes the figure matrix?
8. Among the four answer figures, which one can be formed from the cut out pieces given below in the question figures?
9. A piece of paper is folded and cut as shown below in the question figures. From the given answer figures, indicates how 10. In the following question three dots are placed in the figure marked as (A). The figure is followed by four alternatives
Mathematics
1. If $$A = \{ x:{x^2} = 1\} $$ and $$B = \{ x:{x^4} = 1\} $$, then A $$\Delta$$ B is equal to 2. If $$2f(xy) = {(f(x))^x} + {(f(y))^x}$$ for all $$x,y \in R$$ and $$f(1) = a( \ne 1)$$. Then $$\sum\limits_{k = 1}^n {f( 3. Let f(x) = x $$-$$ 3, g(x) = 4 $$-$$ x. Then the set of values of x for which $$|f(x) + g(x)|\, 4. If a1, a2, a3, ......., a20 are AM's between 13 and 67, then the maximum value of a1, a2, a3, ......, a20 is equal to 5. If p, q, r are in AP and are positive, the roots of the quadratic equation px2 + qx + r = 0 are all real for 6. The value of $${}^{47}{C_4} + \sum\limits_{r = 1}^5 {{}^{52 - r}{C_3}} $$ is equal to 7. The number of numbers divisible by 3 that can be formed by four different even digits is 8. If n(A) = 1000, n(B) = 500, n(A $$\cap$$ B) $$\ge$$ 1 and n(A $$\cup$$ B) = P, then 9. $$\left\{ {x \in R:{{2x - 1} \over {{x^3} + 4{x^2} + 3x}} \in R} \right\}$$ is equal to 10. Let f(x) be a polynomial function of second degree. If f(1) = f($$-$$1) and a, b, c are in AP, then f'(a), f'(b) and f'( 11. The value of $$\mathop {\lim }\limits_{x \to \infty } {1 \over n}\left\{ {{1 \over {n + 1}} + {2 \over {n + 2}} + .... + 12. The coefficient of x8 in the polynomial (x $$-$$ 1) (x $$-$$ 2) ..... (x $$-$$ 10) 13. If $$z = {{7 + i} \over {3 + 4i}}$$, then z14 is 14. The solution of the equation $${{dy} \over {dx}} + {1 \over x}\tan y = {1 \over {{x^2}}}\tan y\sin y$$ is 15. The value of the definite integral $$\int\limits_0^{\pi /2} {{{dx} \over {\tan x + \cot x + \cos ec\,x + \sec x}}} $$ 16. If a and b are two vectors such that | a | = 1, | b | = 4 a . b = 2. If c = (2a $$\times$$ b) $$-$$ 3b, then angle betwe 17. Let x1 and x2 be the real roots of the equation $${x^2} - (k - 2)x + ({k^2} + 3k + 5) = 0$$, then maximum value of $$x_1 18. Circle centered at origin and having radius $$\pi$$ units is divided by the curve y = sin x in two parts. Then area of u 19. The root of the equation $$2(1 + i){x^2} - 4(2 - i)x - 5 - 3i = 0$$, where $$i = \sqrt { - 1} $$, which has greater modu 20. The equation $$(\cos \beta - 1){x^2} + (\cos \beta )x + \sin \beta = 0$$ in the variable x has real roots, then $$\bet 21. An ordered pair ($$\alpha$$, $$\beta$$) for which the system of linear $$(1 + \alpha )x + \beta y + z = 2$$, $$\alpha x 22. A bird is sitting on the top of a vertical pole 20 m high and its elevation from a point O on the ground is 45$$^\circ$$ 23. If one GM, g and two AM's p and q are inserted between two numbers a and b, then (2p $$-$$ q) (p $$-$$ 2q) is equal to 24. When x100 is divided by x2 $$-$$ 3x + 2, the remainder is (2k + 1 $$-$$ 1)x $$-$$(2k $$-$$ 1), then k is 25. The mean of five observation is 5 and their variance is 9.20. If three of the given five observation are 1, 3 and 8, the 26. How many three digit number satisfy the property that the middle digit is arithmetic mean of the first and the last digi 27. If $$z = r{e^{i\theta }}$$, then arg(eiz) is 28. If 4 dice are rolled, then the number of ways of getting the sum 10 is 29. The measurement of the distance from point A(1, 2) parallel to the line 3x $$-$$ y = 10 to the line x + y + 5 = 0 is 30. Let $$f(x) = {a_0} + {a_1}{x^2} + {a_2}{x^4} + {a_3}{x^6} + ... + {a_n}{x^{2n}}$$ be a polynomial in a real variable x w 31. Given that $$f(x) = 2{x^3} + {x^4} + \log x$$ and assuming g to be the inverse function of f, compute the value of g'(3) 32. A line passing through P(3, 7, 1) and R(2, 5, 7) meet the plane 3x + 2y + 11z $$-$$ 9 = 0 at Q. Then PQ is equal to 33. $$\int {{{8{x^{43}} + 13{x^{38}}} \over {{{({x^{13}} + {x^5} + 1)}^4}}}dx} $$ equals to 34. If $$a = - \widehat i + \widehat j + \widehat k$$ and $$b = 2\widehat i + \widehat k$$, then find z component of a vect 35. Given that x, y, and z are three consecutive positive integers and x $$-$$ z + 2 = 0, what is the value of $${1 \over 2} 36. The solution of differential equation $$(x{y^5} + 2y)dx - xdy = 0$$, is
37. The solution set of $${{|x - 2|\, - 1} \over {|x - 2|\, - 2}} \le 0$$ is 38. Let $$f(x) = {x \over {\sqrt {1 + {x^2}} }}$$, $$\underbrace {fofofo.....of(x)}_{x\,times}$$ is 39. If $${\log _5}{{(a + b)} \over 3} = {{{{\log }_5}a + {{\log }_5}b} \over 2}$$, then $${{{a^4} + {b^4}} \over {{a^2}{b^2} 40. The number of distinct solutions of the equation $${5 \over 4}{\cos ^2}2x + {\cos ^4}x + {\sin ^4}x + {\cos ^6}x = 2$$ i 41. If the tangent at a point $$\left( {4\cos \phi ,{{16} \over {\sqrt {11} }}\sin \phi } \right)$$ to the ellipse $$16{x^2} 42. The distance of point of intersection of the tangents to the parabola x = 4y $$-$$ y2 drawn at the points where it is me 43. The value of the sum $$\sum\limits_{k = 1}^\infty {\sum\limits_{n = 1}^\infty {{k \over {{2^{n + k}}}}} } $$ is 44. A curve passes through (2, 0) and the slope of the tangent at P(x, y) is equal to $${{{{(x + 1)}^2} + y - 3} \over {x + 45. Consider matrix $$A = \left[ {\matrix{
2 & 1 \cr
1 & 2 \cr
} } \right]$$, if $${A^{ - 1}} = \alpha I + \beta
Physics
1. Three solenoid coils of same dimensions, same number of turns and same number of layers of windings are taken. Coil 1 ha 2. Three capacitors C1, C2 and C3 are connected as shown in the figure below. If capacitor C3 breaks down electrically, the 3. A black body radiates energy at the rate E Wm$$-$$2 at high temperature TK. When the temperature is reduced to $$\left( 4. The length of the rectangle is l = 15.2 cm and breadth is b = 2.9 cm and the minimum possible measurement by scale = 0.1 5. In an adiabatic process, where pressure is decreased by $${3 \over 4}$$%, if $${{{C_p}} \over {{C_v}}} = {4 \over 3}$$, 6. The vibrations of a string of length 60 cm fixed at both ends are represented by the equation
$$y = 4\sin \left( {{{\pi 7. A charge +q is placed at the origin O of XY-axes as shown in the figure. The work done in taking a charge Q from A to B 8. Identify the hydrogen-like element whose spectral lines are four times shorter in wavelength compared to those of atomic 9. Two small conducting spheres of equal radius have charges +20 $$\mu$$ C and $$-$$40 $$\mu$$C respectively and placed at 10. A Carnot engine has the same efficiency between 600 K to 300 K and 1600 K to x K, then the value of x is 11. A ball of mass 0.5 kg is thrown up with initial speed 16 ms$$-$$1 and reaches maximum height of 9 m. How much energy is 12. In the circuit shown, if the 10 $$\Omega$$ resistor is replaced by a resistor of 15 $$\Omega$$, then what is the amount 13. A time dependent force F = (8t) acts on a particle of mass 2 kg. If the particle starts from rest, the work done by the 14. If L, R, C and V represent inductance, resistance, capacitance and potential difference respectively, then dimensions of 15. The magnetic field of a beam emerging from a fitter facing a flood light is given by
B = 10 $$\times$$ 10$$-$$8 sin(1 $$ 16. Based on the provided velocity-time graph for an object’s straight-line movement, determine the total distance covered a 17. A thin equi-convex lens is made of glass of refractive index 1.5 and its focal length is 0.2 m. If it acts as a concave 18. A moving coil galvanometer has a resistance of 60 $$\Omega$$ and it indicates full deflection on passing a current of 4. 19. The combination of the gates shown in following figure yields
20. A vessel contains one mole of O2 gas (molar mass 32) at a temperature T. The pressure of the gas is p. An identical vess 21. The acceleration of a particle in m/s2 is given by a = (3t2 $$-$$ 2t + 1), where t is in second. If the particle starts 22. The figure shows graphs of pressure (p) versus density (d) for an ideal gas at two temperatures T1 and T2, then
23. Two spheres of the same material and same radii r are touching each other. The gravitational force between the spheres i 24. A spherical lens of power $$-$$4D is placed at a distance of 15 cm from another spherical lens of power 5 D. A beam of p 25. The wheel of a car, accelerated uniformly from rest, rotates through 5 radians during the first second. The angle (in ra 26. Lights of two different frequencies whose photons have energies 1.5 eV and 2.5 eV respectively illuminate a metallic sur 27. When a body is dropped from a height h, then it hits the ground with a momentum p. If the same body is dropped from a he 28. The reading of the ammeter for a germanium diode in the given circuit is
29. The decay constants of two radioactive substances X and Y are 4$$\lambda$$ and $$\lambda$$ respectively. At t = 0, a sam 30. What is the magnitude of the force experienced per unit length by a thin wire that carries a current of I = 10 A and is 31. A long cylindrical iron core of cross-sectional area 5 cm2 is inserted into a long solenoid having 4000 turns/meter and 32. A plane requires for take off a speed of 72 kmh$$-$$1, the run on the ground being 50 m. The mass of the plane is 10000 33. Given that in a fluorescent lamp's choke, a reverse voltage of 120 V is generated as the choke's current changes steadil 34. If a man becomes a giant, expanding his linear dimensions by a factor of eight, and assuming his density remains unchang 35. When 2 moles of a monoatomic gas are mixed with 3 moles of a diatomic gas, the value of adiabatic exponent for the mixtu 36. A simple pendulum is placed inside a lift, the lift is moving with a uniform acceleration. If the time periods of the pe 37. A satellite is moving around the earth with speed v in a circular orbit of radius r. If the orbit radius is decreased by 38. In the circuit shown in figure, the AC source has angular frequency $$\omega$$ = 2000 rad s$$-$$1. The amplitude of the 39. The work done by a force acting on a body is as shown in the following graph. The total work done in covering an initial 40. A potentiometer wire, 10 m long, has a resistance of 40$$\Omega$$. It is connected in series with a resistance box and a
1
BITSAT 2020
MCQ (Single Correct Answer)
+3
-1
A(g) $$\to$$ P(g) + Q(g) + R(g),
Follow first order kinetics with a half-life of 69.3 s at 500$$^\circ$$C. Starting from the gas 'A' an container at 500$$^\circ$$C and at a pressure of 0.4 atm, the total pressure of the system after 230 s will be
A
1.15 atm
B
1.32 atm
C
1.22 atm
D
1.12 atm
2
BITSAT 2020
MCQ (Single Correct Answer)
+3
-1
Which of the following gives paracetamol on acetylation?
A

B
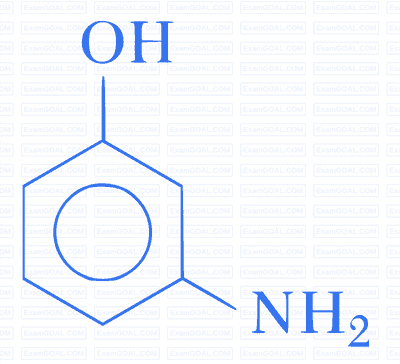
C
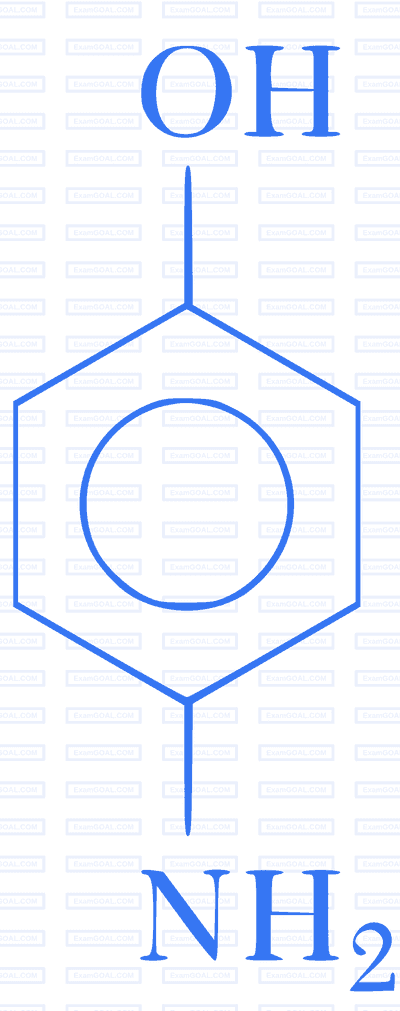
D

3
BITSAT 2020
MCQ (Single Correct Answer)
+3
-1
In qualitative analysis when H2S is passed through an aqueous solution of salt acidified with dilute HCl, a black ppt. is obtained. On boiling the precipitate with dil. HNO3, it forms a solution of blue colour. Addition of excess of aqueous solution of NH3 to this solution gives
A
deep blue ppt. of Cu(OH)2
B
deep blue solution of [Cu(NH3)4]2+
C
deep blue solution of Cu(NO3)2
D
deep blue solution of Cu(OH)2 . Cu(NO3)2
4
BITSAT 2020
MCQ (Single Correct Answer)
+3
-1
Titration of 0.1467 g of primary standard Na2C2O4 required 28.85 mL of KMnO4
solution. Calculate the molar concentration of KMnO4 solution.A
0.01518 M
B
0.001518 M
C
0.15180 M
D
1.5180 M
Paper analysis
Total Questions
Chemistry
40
English Proficiency
15
Logical Reasoning
10
Mathematics
45
Physics
40
More papers of BITSAT
BITSAT
Papers
2024
2023
2022
2021
2020