1
IAT (IISER) 2024
MCQ (Single Correct Answer)
+4
-1
A solid bob of a material having density twice that of water is suspended with a massless and inextensible string of length $L$. The whole set-up is placed inside a water-filled tank. The bob is imparted a horizontal velocity $V_0$ at the lowest point A , while the other end of the string is fixed, such that the bob completes a semi-circular trajectory in the vertical plane. The string becomes slack only when the bob reaches the topmost point C. Assume that the effects of viscosity and water currents are negligible. The acceleration due to gravity is $g$. What is the expression for $V_0$ ?
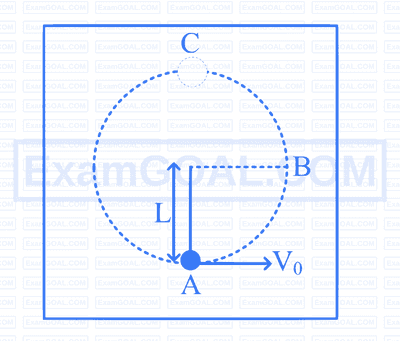
2
IAT (IISER) 2024
MCQ (Single Correct Answer)
+4
-1
Consider a solid sphere of radius $R$ floating in a pond with half of the sphere submerged. The sphere is pushed vertically downwards at the topmost point and released, such that it executes a simple harmonic motion. Acceleration due to gravity is $g$. What is the time period of oscillation?
3
IAT (IISER) 2024
MCQ (Single Correct Answer)
+4
-1
One mole of an ideal gas of volume $V$ and temperature $T$ is allowed to expand adiabatically to volume $2 V$ while doing no external work. The universal gas constant is $R$. What is the pressure of the gas after expansion?
4
IAT (IISER) 2024
MCQ (Single Correct Answer)
+4
-1
Two identical boxes contain the same ideal gas. Let $\left(n_1, \lambda_1, T_1\right)$ and $\left(n_2, \lambda_2, T_2\right)$ be the number density, mean free path and temperature of the gas in the first and the second box, respectively. One of the boxes is empty into the other one. What will be the mean free path? $\lambda$ and temperature $T$ of the gas now?
Paper analysis
Total Questions
Biology
15
Chemistry
15
Mathematics
15
Physics
15
More papers of IAT (IISER)
IAT (IISER)
Papers
2024
2023
2022
2020