The magnetic field at the origin due to a current element $$i d \mathbf{l}$$ placed at a point with vector position $$\mathrm{r}$$ is
A long cylindrical wire of radius $$R$$ carries a uniform current $$I$$ flowing through it. The variation of magnetic field with distance $$r$$ from the axis of the wire is shown by


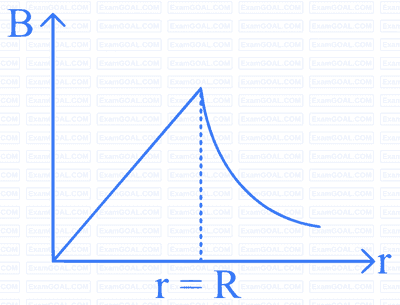
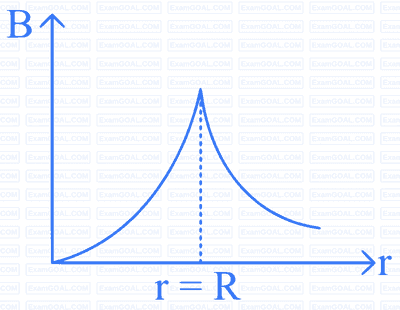
A cyclotron is used to accelerate protons $$\left({ }_1^l \mathrm{H}\right)$$ deuterons $$\left({ }_1^2 \mathrm{H}\right)$$ and $$\alpha$$-particles $$\left({ }_2^4 \mathrm{He}\right)$$. While exiting under similar conditions, the minimum $$\mathrm{KE}$$ is gained by
A paramagnetic sample shows a net magnetisation of $$8 \mathrm{Am}^{-1}$$ when placed in an external magnetic field of $$0.6 \mathrm{~T}$$ at a temperature of $$4 \mathrm{~K}$$. When the same sample is placed in an external magnetic field of $$0.2 \mathrm{~T}$$ at a temperature of $$16 \mathrm{~K}$$, the magnetisation will be